Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Bài 1:
\(a=\lim\limits_{x\rightarrow-\infty}\frac{2\left|x\right|+1}{3x-1}=\lim\limits_{x\rightarrow-\infty}\frac{-2x+1}{3x-1}=\lim\limits_{x\rightarrow-\infty}\frac{-2+\frac{1}{x}}{3-\frac{1}{x}}=-\frac{2}{3}\)
\(b=\lim\limits_{x\rightarrow+\infty}\frac{\sqrt{9+\frac{1}{x}+\frac{1}{x^2}}-\sqrt{4+\frac{2}{x}+\frac{1}{x^2}}}{1+\frac{1}{x}}=\frac{\sqrt{9}-\sqrt{4}}{1}=1\)
\(c=\lim\limits_{x\rightarrow+\infty}\frac{\sqrt{1+\frac{2}{x}+\frac{3}{x^2}}+4+\frac{1}{x}}{\sqrt{4+\frac{1}{x^2}}+\frac{2}{x}-1}=\frac{1+4}{\sqrt{4}-1}=5\)
\(d=\lim\limits_{x\rightarrow+\infty}\frac{\frac{3}{x}-\frac{2}{x\sqrt{x}}+\sqrt{1-\frac{5}{x^3}}}{2+\frac{4}{x}-\frac{5}{x^2}}=\frac{1}{2}\)
Bài 2:
\(a=\lim\limits_{x\rightarrow-\infty}\frac{2+\frac{1}{x}}{1-\frac{1}{x}}=2\)
\(b=\lim\limits_{x\rightarrow-\infty}\frac{2+\frac{3}{x^3}}{1-\frac{2}{x}+\frac{1}{x^3}}=2\)
\(c=\lim\limits_{x\rightarrow+\infty}\frac{x^2\left(3+\frac{1}{x^2}\right)x\left(5+\frac{3}{x}\right)}{x^3\left(2-\frac{1}{x^3}\right)x\left(1+\frac{4}{x}\right)}=\frac{15}{+\infty}=0\)
Giới hạn của dãy nên bạn tự hiểu n tiến tới dương vô cực
1.
\(lim\frac{3n+1}{\sqrt[3]{\left(n^3+3n+1\right)^2}+n\sqrt{n^3+3n+1}+n^2}=lim\frac{3+\frac{1}{n}}{\sqrt[3]{\frac{\left(n^3+3n+1\right)^2}{n^3}}+\sqrt{n^3+3n+1}+n}=\frac{3}{\infty}=0\)
b=\(lim\left(\sqrt[3]{n^3+2n}-n+n-\sqrt{n^2+1}\right)=lim\left(\frac{2n}{\sqrt[3]{\left(n^3+2n\right)^2}+n\sqrt[3]{n^3+2n}+n^2}-\frac{1}{n+\sqrt{n^2+1}}\right)\)
\(=lim\left(\frac{2}{\sqrt[3]{\frac{\left(n^3+2n\right)^2}{n^3}}+\sqrt[3]{n^3+2n}+n}-\frac{1}{n+\sqrt{n^2+1}}\right)=0-0=0\)
c\(=lim\left(\frac{2n^2+n}{\sqrt[3]{\left(n^3+n\right)^2}+\sqrt[3]{\left(n^3+n\right)\left(n^3-2n^2\right)}+\sqrt[3]{\left(n^3-2n^2\right)^2}}\right)\)
\(=lim\left(\frac{2+\frac{1}{n}}{\sqrt[3]{\left(1+\frac{1}{n^2}\right)^2}+\sqrt[3]{\left(1+\frac{1}{n^2}\right)\left(1-\frac{2}{n}\right)}+\sqrt[3]{\left(1-\frac{2}{n}\right)^2}}\right)=\frac{2}{1+1.1+1}=\frac{2}{3}\)
2.
a\(=lim\left[n\left(2-\sqrt{1+\frac{3}{n}}\right)\right]=+\infty\left(2-1\right)=+\infty\)
\(b=lim\left[n\left(\sqrt{1+\frac{2}{n^2}}-\sqrt{\frac{3}{n}+\frac{1}{n^2}}\right)\right]=+\infty\left(1-0\right)=+\infty\)
\(c=lim\left[n^3\left(\frac{sin2n}{n^2}-3\right)\right]=+\infty\left(0-3\right)=-\infty\)
\(K=\lim\limits n\left(\sqrt[3]{1+\frac{1}{n}-\frac{1}{n^3}}-1+3\left(2-\sqrt{4+\frac{1}{n}+\frac{1}{n^2}}\right)\right)\)
\(=\lim\limits n\left[\frac{\frac{1}{n}-\frac{1}{n^3}}{\sqrt[3]{\left(1+\frac{1}{n}-\frac{1}{n^3}\right)^2}+\sqrt[3]{1+\frac{1}{n}-\frac{1}{n^3}}+1}-\frac{3\left(\frac{1}{n}+\frac{1}{n^2}\right)}{2+\sqrt{4+\frac{1}{n}+\frac{1}{n^2}}}\right]\)
\(=\lim\limits\left[\frac{1-\frac{1}{n^2}}{\sqrt[3]{\left(1+\frac{1}{n}-\frac{1}{n^3}\right)^2}+\sqrt[3]{1+\frac{1}{n}-\frac{1}{n^3}}+1}-\frac{3\left(1+\frac{1}{n}\right)}{2+\sqrt{4+\frac{1}{n}+\frac{1}{n^2}}}\right]\)
\(=\frac{1}{1+1+1}-\frac{3}{2+2}=-\frac{5}{12}\)
a) 
(x4 – x2 + x - 1) = x4(1 - 
) = +∞.
b)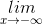 
(-2x3 + 3x2 -5 ) = 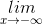x3(-2 + 
) = +∞.
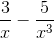c)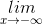 
= 
= +∞.
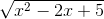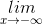
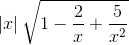d) \(\lim\limits_{x\rightarrow+\infty}\dfrac{\sqrt{x^2+1}+x}{5-2x}=\lim\limits_{x\rightarrow+\infty}\dfrac{\left|x\right|\sqrt{1+\dfrac{1}{x^2}}+x}{5-2x}\)
\(=\lim\limits_{x\rightarrow+\infty}\dfrac{x\sqrt{1+\dfrac{1}{x^2}}+x}{5-2x}\)\(=\lim\limits_{x\rightarrow+\infty}\dfrac{\sqrt{1+\dfrac{1}{x^2}}+1}{\dfrac{5}{x}-2}=-1\).
Bài 1:
\(a=\lim\limits_{x\rightarrow+\infty}\frac{\frac{1}{x}+\frac{2}{\sqrt{x}}-1}{1+\frac{3}{x}}=-1\)
\(b=\lim\limits_{x\rightarrow+\infty}\frac{1+\frac{3}{x^2}-\frac{1}{x^3}}{\frac{1}{\sqrt{x}}+\frac{1}{x^2}}=\frac{1}{0}=+\infty\)
\(c=\lim\limits_{x\rightarrow-\infty}\frac{1-2\sqrt{\frac{1}{x^2}-\frac{1}{x}}}{\frac{1}{x}-1}=\frac{1}{-1}=-1\)
Bài 2:
\(a=\lim\limits_{x\rightarrow0}\frac{1-cosx}{1-cos3x}=\lim\limits_{x\rightarrow0}\frac{sinx}{3sin3x}=\lim\limits_{x\rightarrow0}\frac{\frac{sinx}{x}}{9.\frac{sin3x}{3x}}=\frac{1}{9}\)
\(b=\lim\limits_{x\rightarrow0}\frac{cotx-sinx}{x^3}=\frac{\infty}{0}=+\infty\)
\(c=\lim\limits_{x\rightarrow\infty}\frac{sinx}{2x}\)
Mà \(\left|sinx\right|\le1\Rightarrow\left|\frac{sinx}{2x}\right|\le\frac{1}{\left|2x\right|}\)
Mà \(\lim\limits_{x\rightarrow\infty}\frac{1}{2\left|x\right|}=0\Rightarrow\lim\limits_{x\rightarrow\infty}\frac{sinx}{2x}=0\)
1/ \(\lim\limits_{x\rightarrow0}\dfrac{\sqrt{1+4x}.\sqrt[3]{1+6x}.\sqrt[4]{1+8x}-\sqrt[3]{1+6x}.\sqrt[4]{1+8x}}{x}+\lim\limits_{x\rightarrow0}\dfrac{\sqrt[3]{1+6x}.\sqrt[4]{1+8x}-\sqrt[3]{1+6x}}{x}+\lim\limits_{x\rightarrow0}\dfrac{\sqrt[3]{1+6x}-1}{x}\)
Liên hợp dài quá ko muốn gõ tiếp, bạn tự đặt nhân tử chung rồi liên hợp nhé, kết quả ra 5
2/ \(\lim\limits_{x\rightarrow1}\dfrac{\sqrt[3]{1+7x}-2-\left(x^3-3x+2\right)}{x-1}\)
\(=\lim\limits_{x\rightarrow1}\dfrac{\dfrac{7\left(x-1\right)}{\sqrt[3]{\left(1+7x\right)^2}+2\sqrt[3]{1+7x}+4}-\left(x-1\right)^2\left(x+2\right)}{x-1}\)
\(=\lim\limits_{x\rightarrow1}\dfrac{7}{\sqrt[3]{\left(1+7x\right)^2}+2\sqrt[3]{1+7x}+4}-\left(x-1\right)\left(x+2\right)=\dfrac{7}{12}\)
3/ \(\lim\limits_{x\rightarrow-\infty}\dfrac{x^3-x^2+1}{2x^2+3x-1}=\lim\limits_{x\rightarrow-\infty}\dfrac{x-1+\dfrac{1}{x^2}}{2+\dfrac{3}{x}-\dfrac{1}{x^2}}=-\infty\)
4/ \(\lim\limits_{x\rightarrow+\infty}\dfrac{\sqrt{x}+\sqrt[3]{x}+\sqrt[4]{x}}{\sqrt{4x+1}}=\lim\limits_{x\rightarrow+\infty}\dfrac{1+\dfrac{1}{\sqrt[6]{x}}+\dfrac{1}{\sqrt[4]{x}}}{\sqrt{4+\dfrac{1}{x}}}=\dfrac{1}{\sqrt{4}}=\dfrac{1}{2}\)
5/ \(\lim\limits_{x\rightarrow-\infty}\dfrac{x+\sqrt{x^2+2}}{\sqrt[3]{8x^3+x^2+1}}=\lim\limits_{x\rightarrow-\infty}\dfrac{1-\sqrt{1+\dfrac{2}{x^2}}}{\sqrt[3]{8+\dfrac{1}{x}+\dfrac{1}{x^3}}}=\dfrac{1-1}{\sqrt[3]{8}}=0\)
6/ \(\lim\limits_{x\rightarrow-\infty}\dfrac{\sqrt{4x^2+3x-7}}{\sqrt[3]{27x^3+5x^2+x-4}}=\lim\limits_{x\rightarrow-\infty}\dfrac{-\sqrt{4+\dfrac{3}{x}-\dfrac{7}{x^2}}}{\sqrt[3]{27+\dfrac{5}{x}+\dfrac{1}{x^2}-\dfrac{4}{x^3}}}=\dfrac{-\sqrt{4}}{\sqrt[3]{27}}=\dfrac{-2}{3}\)
\(lim\left(\sqrt{9n^2+10n}-an\right)=-\infty\)
\(\Leftrightarrow lim\dfrac{9n^2+10n-a^2n^2}{\sqrt{9n^2+10n}}=-\infty\)
\(\Leftrightarrow lim\dfrac{9-a^2+\dfrac{10}{n}}{\sqrt{\dfrac{9}{n^2}+\dfrac{10}{n^3}}}=-\infty\)
\(\Leftrightarrow\dfrac{9-a^2}{0}=-\infty\)
\(\Rightarrow a^2>9\)
\(\Leftrightarrow a>3\) \(\Rightarrow a\in\left[4;2023\right]\)