Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

\(VT=\frac{\left(5\sqrt{3}+5\sqrt{2}\right).\left(5-2\sqrt{6}\right)}{5\sqrt{3}-5\sqrt{2}}\)
\(=\frac{\left(5\sqrt{3}+5\sqrt{2}\right)^2.\left(5-2\sqrt{6}\right)}{\left(5\sqrt{3}+5\sqrt{2}\right)\left(5\sqrt{3}-5\sqrt{2}\right)}\)\(=\frac{\left(75+50\sqrt{6}+50\right).\left(5-2\sqrt{6}\right)}{75-50}\)
\(=\frac{25\left(5+2\sqrt{6}\right).\left(5-2\sqrt{6}\right)}{25}=5^2-\left(2\sqrt{6}\right)^2\)\(=25-24=1=VP\)
bn chép lại đề nhé
\(=\frac{\left(5\sqrt{3}+5\sqrt{2}\right)\left(5-2\sqrt{6}\right)}{5\sqrt{3}-5\sqrt{2}}\)
\(=\frac{\left(75+50\sqrt{6}+50\right)\left(\sqrt{3}-\sqrt{2}\right)}{75-50}\)

=\(\left(\frac{\left(\sqrt{x}+\sqrt{y}\right)\left(\sqrt{x}-\sqrt{y}\right)}{\sqrt{x}-\sqrt{y}}-\frac{\left(\sqrt{x}-\sqrt{y}\right)\left(x+\sqrt{xy}+y\right)}{\left(\sqrt{x}+\sqrt{y}\right)\left(\sqrt{x}-\sqrt{y}\right)}\right).\frac{\sqrt{x}+\sqrt{y}}{x-\sqrt{xy}+y}\)
\(=\left[\left(\sqrt{x}+\sqrt{y}\right)-\frac{x+\sqrt{xy}+y}{\sqrt{x}+\sqrt{y}}\right].\frac{\sqrt{x}+\sqrt{y}}{x-\sqrt{xy}+y}\)
\(=\frac{x+2\sqrt{xy}+y-x-\sqrt{xy}-y}{\sqrt{x}+\sqrt{y}}.\frac{\sqrt{x}+\sqrt{y}}{x-\sqrt{xy}+y}\)
\(=\frac{\sqrt{xy}}{x-\sqrt{xy}+y}\)
Mình gi rút gọn bạn tự hiểu nha:
\(\left(\frac{x-y}{\sqrt{x}-\sqrt{y}}+\frac{\sqrt{x^3}-\sqrt{y^3}}{y-x}\right):\frac{\left(\sqrt{x}-\sqrt{y}\right)^2+\sqrt{xy}}{\sqrt{x}+\sqrt{y}}\)
=\(\left(\sqrt{x}-\sqrt{y}-\frac{\left(\sqrt{x}-\sqrt{y}\right)\left(x-\sqrt{xy}+y\right)}{x-y}\right).\frac{\sqrt{x}+\sqrt{y}}{x+y-\sqrt{xy}}\)
=\(\frac{\left(\sqrt{x}-\sqrt{y}\right)\left(\sqrt{x}+\sqrt{y}\right)}{x+y-\sqrt{xy}}-\frac{\left(\sqrt{x}-\sqrt{y}\right)\left(\sqrt{x}+\sqrt{y}\right)\left(x+y-\sqrt{xy}\right)}{\left(x-y\right)\left(x+y-\sqrt{xy}\right)}\)
=

\(1,\sqrt{\left(2+\sqrt{7}\right)^2-\sqrt{\left(2-\sqrt{7}\right)^2}}\) ( áp dụng hđt thứ 3 \(a^2-b^2=\left(a-b\right)\left(a+b\right)\))
\(=\sqrt{\left(2+\sqrt{7}+2-\sqrt{7}\right)\left(2+\sqrt{7}-2+\sqrt{7}\right)}\)
\(=\sqrt{4\cdot\sqrt{7}}\)
\(2,\sqrt{\left(3\sqrt{5}-5\sqrt{2}\right)^2}-\sqrt{\left(5\sqrt{2}+3\sqrt{5}\right)^2}\)
\(\Leftrightarrow\sqrt{\left(3\sqrt{5}-5\sqrt{2}\right)^2}=\sqrt{\left(5\sqrt{2}+3\sqrt{5}\right)^2}\)
\(\Leftrightarrow\left(3\sqrt{5}-5\sqrt{2}\right)^2=\left(5\sqrt{2}+3\sqrt{5}\right)^2\)
\(\Leftrightarrow\left(3\sqrt{5}-5\sqrt{2}\right)^2-\left(5\sqrt{2}+3\sqrt{5}\right)^2\)
\(=\left(3\sqrt{5}-5\sqrt{2}+5\sqrt{2}+3\sqrt{5}\right)\left(3\sqrt{5}-5\sqrt{2}-5\sqrt{2}-3\sqrt{5}\right)\)
\(=6\sqrt{5}\cdot\left(-10\sqrt{2}\right)\)
\(3,\sqrt{10+2\sqrt{21}}-\sqrt{10-2\sqrt{21}}\)
\(\Leftrightarrow\sqrt{10+2\sqrt{21}}=\sqrt{10-2\sqrt{21}}\)
\(\Leftrightarrow10+2\sqrt{21}=10-2\sqrt{21}\)
\(\Leftrightarrow4\sqrt{21}\)
cuối lười tính nên thôi nhá :>

a. \(A=\frac{\sqrt{x}}{\sqrt{x}+3}+\frac{2\sqrt{x}}{\sqrt{x}-3}-\frac{3\left(x+3\right)}{\left(\sqrt{x}+3\right)\left(\sqrt{x}-3\right)}\)
\(=\frac{x-3\sqrt{x}+2x+6\sqrt{x}-3x-9}{\left(\sqrt{x}+3\right)\left(\sqrt{x}-3\right)}=\frac{3\sqrt{x}-9}{\left(\sqrt{x}+3\right)\left(\sqrt{x}-3\right)}\)
\(=\frac{3}{\sqrt{x}+3}\)
. \(x=2.\left(4+\sqrt{15}\right).\left(\sqrt{10}-\sqrt{6}\right).\sqrt{4-\sqrt{15}}\)
\(\Rightarrow x=\left(\sqrt{5}+\sqrt{3}\right)^2.\sqrt{2}\left(\sqrt{5}-\sqrt{3}\right).\frac{\left(\sqrt{5}-\sqrt{3}\right)^2}{\sqrt{2}}\)
\(=\left(\sqrt{5}+\sqrt{3}\right)^2.\left(\sqrt{5}-\sqrt{3}\right)^3\)\(=4\left(\sqrt{5}-\sqrt{3}\right)\)
Thay \(x=4\left(\sqrt{5}-\sqrt{3}\right)\Rightarrow A=\frac{3}{\sqrt{4\left(\sqrt{5}-\sqrt{3}\right)}+3}\)
\(=\frac{3}{2\sqrt{\left(\sqrt{5}-\sqrt{3}\right)}+3}\)
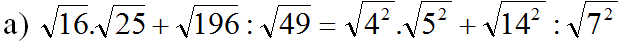
\(=\left(\sqrt{2-\sqrt{3}}\right)^2-2\sqrt{\left(\sqrt{2-\sqrt{3}}\right)\left(\sqrt{2+\sqrt{3}}\right)}+\left(\sqrt{2+\sqrt{3}}\right)^2\)
\(=2-\sqrt{3}-2\sqrt{4-3}+2+\sqrt{3}\)
\(=4-2\)
\(=2\)
bằng 4.
dùng hđt như bình thường thôi