Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Đặt \(f\left(x\right)=\left(2m^2-5m+2\right)\left(x+2\right)^{2019}\left(x^{2020}-4\right)+5x-1\)
Do \(f\left(x\right)\) là hàm đa thức nên liên tục và xác định trên R
Ta có: \(f\left(-2\right)=-11< 0\)
Lại có: \(4>1\Rightarrow\sqrt[2020]{4}>1\Rightarrow5\sqrt[2020]{4}>1\)
\(\Rightarrow f\left(\sqrt[2020]{4}\right)=5\sqrt[2020]{4}-1>0\)
\(\Rightarrow f\left(-2\right).f\left(\sqrt[2020]{4}\right)< 0;\forall m\)
\(\Rightarrow f\left(x\right)\) luôn có ít nhất 1 nghiệm thuộc \(\left(-2;\sqrt[2020]{4}\right)\) hay pt đã cho luôn luôn có nghiệm với mọi m

6.
\(\Leftrightarrow\frac{1}{2}cos6x+\frac{1}{2}cos4x=\frac{1}{2}cos6x+\frac{1}{2}cos2x+\frac{3}{2}+\frac{3}{2}cos2x+1\)
\(\Leftrightarrow cos4x=4cos2x+5\)
\(\Leftrightarrow2cos^22x-1=4cos2x+5\)
\(\Leftrightarrow cos^22x-2cos2x-3=0\)
\(\Leftrightarrow\left[{}\begin{matrix}cos2x=-1\\cos2x=3>1\left(ktm\right)\end{matrix}\right.\)
\(\Leftrightarrow...\)
7.
Thay lần lượt 4 đáp án ta thấy chỉ có đáp án C thỏa mãn
8.
\(\Leftrightarrow\left[{}\begin{matrix}sinx=1\\sinx=\frac{1}{2}\end{matrix}\right.\) \(\Leftrightarrow\left[{}\begin{matrix}x=\frac{\pi}{2}+k2\pi\\x=\frac{\pi}{6}+k2\pi\\x=\frac{5\pi}{6}+k2\pi\end{matrix}\right.\)
\(\Rightarrow x=\left\{\frac{\pi}{6};\frac{\pi}{2}\right\}\)
9.
Đặt \(sinx+cosx=t\Rightarrow\left\{{}\begin{matrix}-1\le t\le1\\sinx.cosx=\frac{t^2-1}{2}\end{matrix}\right.\)
\(\Rightarrow mt+\frac{t^2-1}{2}+1=0\)
\(\Leftrightarrow t^2+2mt+1=0\)
Pt đã cho có đúng 1 nghiệm thuộc \(\left[-1;1\right]\) khi và chỉ khi: \(\left[{}\begin{matrix}m\ge1\\m\le-1\end{matrix}\right.\)
10.
\(\frac{\sqrt{3}}{2}cos5x-\frac{1}{2}sin5x=cos3x\)
\(\Leftrightarrow cos\left(5x-\frac{\pi}{6}\right)=cos3x\)
\(\Leftrightarrow\left[{}\begin{matrix}5x-\frac{\pi}{6}=3x+k2\pi\\5x-\frac{\pi}{6}=-3x+k2\pi\end{matrix}\right.\)

Nhận thấy \(x=0\) không phải là nghiệm của BPT đã cho, chia 2 vế cho \(x^2\):
\(\Leftrightarrow\frac{\left(x^2-2x+4\right)}{x}.\frac{\left(x^2+x+4\right)}{x}-a-2018\le0\)
\(\Leftrightarrow\left(x+\frac{4}{x}-2\right)\left(x+\frac{4}{x}+1\right)-a-2018\le0\)
Đặt \(x+\frac{4}{x}=t\) \(\left(\left|t\right|\ge4\right)\) BPT trở thành:
\(\left(t-2\right)\left(t+1\right)-a-2018\le0\)
\(\Leftrightarrow t^2-t-a-2020\le0\)
\(\Leftrightarrow t^2-t-2020\le a\)
Xét \(f\left(t\right)=t^2-t-2020\) với \(\left|t\right|\ge2\)
Để BPT đã cho có nghiệm thì \(a\ge\min\limits_{\left|t\right|\ge2}f\left(t\right)\)
\(f'\left(t\right)=2t-1=0\Rightarrow t=\frac{1}{2}\)
\(f\left(-2\right)=-2014\) ; \(f\left(2\right)=-2018\)
\(\Rightarrow\min\limits_{\left|t\right|\ge2}f\left(t\right)=f\left(2\right)=-2018\)
\(\Rightarrow a\ge-2018\)

(1−m2)(x+1)3+x2−x−3=0
f(x)=(1−m2) (x+1)3+x2−x−3 là hàm đa thức liên tục trên R. Do đó nó liên tục trên [-2; -1]
Ta có f(−1)=−1<0 và f(−2)=m2+2>0 nên f(−1) f(−2)<0 với mọi m.
Do đó, phương trình f(x)=0 luôn có ít nhất một nghiệm trong khoảng (-2; -1) với mọi m. Nghĩa là, phương trình (1−m2) (x+1)3+x2−x−3 luôn có nghiệm với mọi m.
Do hàm số \(\left(1-m^2\right)\left(x+1\right)^3+x^2-x-3\) là hàm đa thức nên nó liên tục trên R, nên liên tục trên \(\left[-2,-1\right]\)
\(f\left(-1\right)=-1< 0;f\left(-2\right)=m^2+2>0\)
\(\Rightarrow f\left(1\right).f\left(-2\right)< 0\)
Do đó phương trình luôn có nghiệm

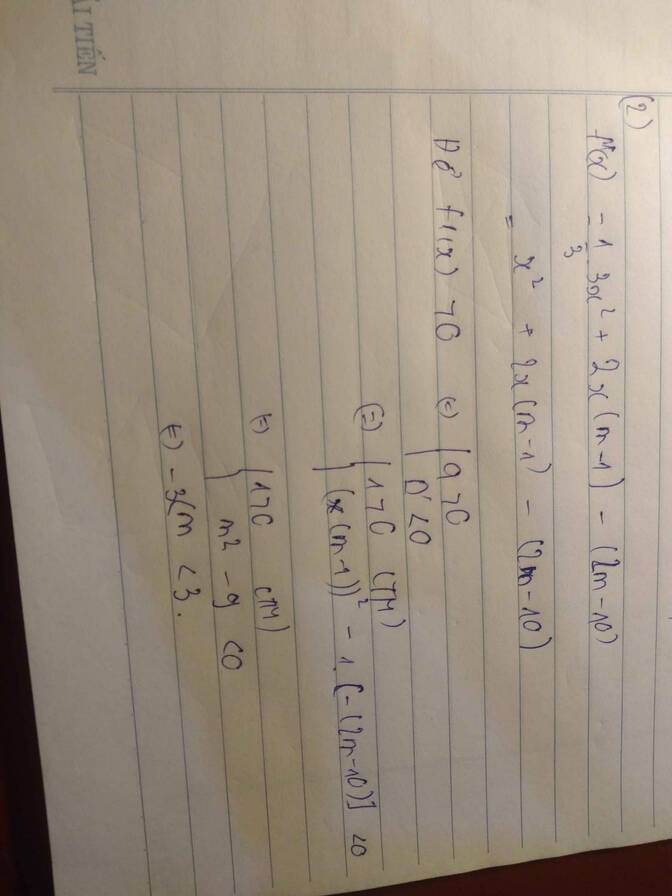
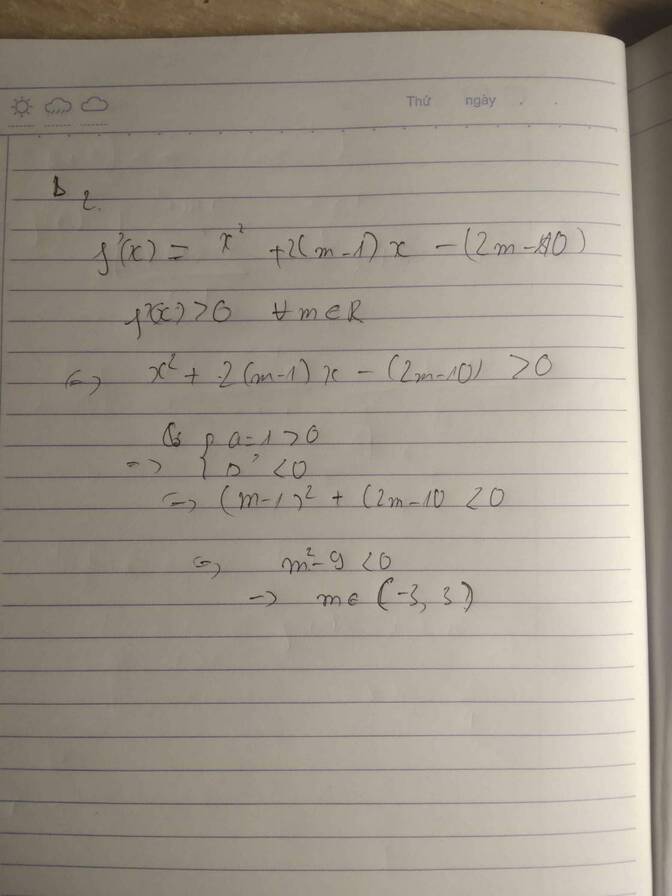
Nếu phương trình là \(\left(2m^2-5m+2\right)\left(x-1\right)^{2021}\left(x^{2020}-2\right)+2x^2-3=0\) thì còn có cơ hội giải quyết
Chứ đề đúng thế này thì e rằng không có cơ hội nào cả.