TQ
1
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
20 tháng 2 2020
\(PT\Leftrightarrow\left(\frac{x-5}{2020}-1\right)+\left(\frac{x-6}{2019}-1\right)-\left(\frac{x-7}{2018}-1\right)-\left(\frac{x-8}{2017}-1\right)=0\)
\(\Leftrightarrow\left(x-2025\right)\left(\frac{1}{2020}+\frac{1}{2019}-\frac{1}{2018}-\frac{1}{2017}\right)=0\)
Dễ thấy \(\left(\frac{1}{2020}+\frac{1}{2019}-\frac{1}{2018}-\frac{1}{2017}\right)< 0\)
\(\Rightarrow x=2025=5^2.3^4\)
Vậy các ước nguyên tố của nghieemh pt là 3,5
TN
1
NV
Nguyễn Việt Lâm
Giáo viên
14 tháng 2 2020
Nhận thấy \(x=\left\{2019;2020\right\}\) là 2 nghiệm của pt đã cho
- Với \(x>2020\Rightarrow\left\{{}\begin{matrix}\left|x-2019\right|^{2019}>1\\\left|x-2020\right|^{2020}>0\end{matrix}\right.\) \(\Rightarrow VT>1>VP\)
\(\Rightarrow\) pt vô nghiệm
- Với \(x< 2019\Rightarrow\left\{{}\begin{matrix}\left|x-2019\right|^{2019}>0\\\left|x-2020\right|^{2020}>1\end{matrix}\right.\) \(\Rightarrow VT>1>VP\)
Pt vô nghiệm
- Với \(2019< x< 2020\Rightarrow\left\{{}\begin{matrix}0< x-2019< 1\\0< 2020-x< 1\end{matrix}\right.\)
\(\Rightarrow\left\{{}\begin{matrix}\left|x-2019\right|^{2019}< x-2019\\\left|2020-x\right|^{2020}< 2020-x\end{matrix}\right.\)
\(\Rightarrow VT< x-2019+2020-x=1\Rightarrow VT< VP\)
Pt vô nghiệm
Vậy pt có đúng 2 nghiệm \(\left[{}\begin{matrix}x=2019\\x=2020\end{matrix}\right.\)
TT
1
20 tháng 3 2023
=>\(\left(\dfrac{x+1}{2021}+1\right)+\left(\dfrac{x+2}{2020}+1\right)+\left(\dfrac{x+3}{2019}+1\right)+\left(\dfrac{x+2028}{2}-3\right)=0\)
=>x+2022=0
=>x=-2022
NT
1
NC
17 tháng 3 2021
Ta có:\(1+x+x^2+x^3+...+x^{2020}=0\)
\(\Leftrightarrow1+\left(x+x^2\right)+\left(x^3+x^4\right)+...+\left(x^{2019}+x^{2020}\right)=0\)
Mà \(x+x^2\ge0\forall x\)
\(x^3+x^4\ge0\forall x\)
........
\(x^{2019}+x^{2020}\ge0\forall x\)
\(\Leftrightarrow1+\left(x+x^2\right)+\left(x^3+x^4\right)+...+\left(x^{2019}+x^{2020}\right)\ge1\forall x\)
Theo bài ra:\(1+\left(x+x^2\right)+\left(x^3+x^4\right)+...+\left(x^{2019}+x^{2020}\right)=0\)
\(\Rightarrow\)Vô nghiệm
P
0
S
4
20 tháng 4 2020
\(\frac{x-2}{2017}+\frac{x-3}{2018}=\frac{x-4}{2019}+\frac{x-5}{2020}\)
<=> \(\frac{x-2}{2017}+1+\frac{x-3}{2018}+1=\frac{x-4}{2019}+1+\frac{x-5}{2020}+1\)
<=> \(\frac{x+2015}{2017}+\frac{x+2015}{2018}-\frac{x+2015}{2019}-\frac{x+2015}{2020}=0\)
<=> \(\left(x+2015\right)\left(\frac{1}{2017}+\frac{1}{2018}-\frac{1}{2019}-\frac{1}{2020}\right)=0\)
<=> x + 2015 = 0 ( vì \(\frac{1}{2017}+\frac{1}{2018}-\frac{1}{2019}-\frac{1}{2020}\ne0\))
<=> x = - 2015
Vậy x = -2015.
20 tháng 4 2020
Giải phương trình :
\(\frac{x-2}{2017}+\frac{x-3}{2018}=\frac{x-4}{2019}+\frac{x-5}{2020}\)
\(\Rightarrow\frac{x-2}{2017}+1+\frac{x-3}{2018}+1=\frac{x-4}{2019}+1+\frac{x-5}{2020}+1\)
\(\Rightarrow\frac{x+2015}{2017}+\frac{x+2015}{2018}-\frac{x+2015}{2019}-\frac{x+2015}{2020}=0\)
\(\Rightarrow\left(x+2015\right)\left(\frac{1}{2017}+\frac{1}{2018}-\frac{1}{2019}-\frac{1}{2020}\right)=0\)
Mà \(\left(\frac{1}{2017}+\frac{1}{2018}-\frac{1}{2019}-\frac{1}{2020}\right)>0\)
\(\Rightarrow x+2015=0\)
\(\Rightarrow x=-2015\)
TQ
0
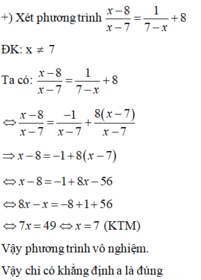
Ta có: (x-2019)(x+2020)=0
\(\Leftrightarrow\left[{}\begin{matrix}x-2019=0\\x+2020=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=2019\\x=-2020\end{matrix}\right.\)
Vậy: S={2019;-2020}