Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

a. \(\sqrt{4\left(a-3\right)^2}=2.|a-3|=2\left(a-3\right)\) (vì a \(\ge3\) nên a-3\(\ge\) 0. Do đó: \(|a-3|=a-3\))
b. \(\sqrt{9\left(b-2\right)^2}=3.|b-2|=3\left(2-b\right)\) (vì b < 2 nên b-2 < 0. Do đó : \(|b-2|=2-b\))
c. \(\sqrt{a^2\left(a+1\right)^2}=a\left(a+1\right)\) ( vì a > 0)
d. \(\sqrt{b^2\left(b-1\right)^2}=b\left(b-1\right)\) (vì b < 0)

b: \(=\left|b\cdot\left(b-1\right)\right|=b\cdot\left|b-1\right|\)
c: \(=\left|a\right|\cdot\left|a+1\right|=a\left(a+1\right)=a^2+a\)
d: \(=1-2a-4a=-6a+1\)

a) \(\sqrt{4\left(a-3\right)^2}=\sqrt{2^2\left(a-3\right)^2}=2\sqrt{\left(a-3\right)^2}=2.\left|a-3\right|=2\left(a-3\right)=2a-6\) (Vì \(a\ge3\) )
b) \(\sqrt{9\left(b-2\right)^2}=\sqrt{3^2\left(b-2\right)^2}=3\sqrt{\left(b-2\right)^2}=3\left|b-2\right|=3\left(2-b\right)\)
\(=6-3b\) (vì b < 2 )
b) \(\sqrt{27.48\left(1-a\right)^2}=\sqrt{27.3.16.\left(1-a\right)^2}=\sqrt{81.16.\left(1-a\right)^2}\)
\(=\sqrt{9^2.4^2.\left(1-a\right)^2}=9.4\sqrt{\left(1-a\right)^2}=36.\left|1-a\right|=36\left(1-a\right)=36-36a\) (vì a > 1)

\(A=\left(x-2\right)\cdot\sqrt{\dfrac{9}{\left(x-2\right)^2}}+3=\dfrac{3\left(x-2\right)}{\left|x-2\right|}+3=\dfrac{3\left(x-2\right)}{-\left(x-2\right)}=-3+3=0\)
\(B=\sqrt{\dfrac{a}{6}}+\sqrt{\dfrac{2a}{3}}+\sqrt{\dfrac{3a}{2}}=\dfrac{\sqrt{a}}{\sqrt{6}}+\dfrac{\sqrt{2a}}{\sqrt{3}}+\dfrac{\sqrt{3a}}{\sqrt{2}}=\dfrac{\sqrt{a}+2\sqrt{a}+3\sqrt{a}}{\sqrt{6}}=\dfrac{6\sqrt{a}}{\sqrt{6}}=\sqrt{6a}\)
\(E=\sqrt{9a^2}+\sqrt{4a^2}+\sqrt{\left(1-a\right)^2}+\sqrt{16a^2}=3\left|a\right|+2\left|a\right|+\left|1-a\right|+4\left|a\right|=9\left|a\right|+1-a=-9a+1-a=-10a+1\)
\(F=\left|x-2\right|\cdot\dfrac{\sqrt{x^2}}{x}=\left|x-2\right|\cdot\dfrac{\left|x\right|}{x}=\dfrac{x\left(x-2\right)}{x}=x-2\)
\(H=\dfrac{x^2+2\sqrt{3}\cdot x+3}{x^2-3}=\dfrac{\left(x+\sqrt{3}\right)^2}{\left(x-\sqrt{3}\right)\left(x+\sqrt{3}\right)}=\dfrac{x+\sqrt{3}}{x-\sqrt{3}}\)
\(I=\left|x-\sqrt{\left(x-1\right)^2}\right|-2x=\left|x-\left(-\left(x-1\right)\right)\right|-2x=\left|x+x-1\right|-2x=\left|2x-1\right|-2x=1-4x\)

a, \(\sqrt{\left(2-\sqrt{5}\right)^2}=\sqrt{5}-2\left(\sqrt{5}>2\right)\)
b, \(\sqrt{\left(3-\sqrt{2}\right)^2}=3-\sqrt{2}\left(3>\sqrt{2}\right)\)
c, Với a < 3
\(\sqrt{\left(a-3\right)^2}+\left(a-9\right)=3-a+a-9=-6\)
d, \(A=\sqrt{\left(2a+5\right)^2}-\left(2a-7\right)\)
\(=\left|2a+5\right|-2a+7\)
+) Xét \(x\ge\dfrac{-5}{2}\) có:
\(A=2a+5-2a+7=12\)
+) Xét \(x< \dfrac{-5}{2}\) có:
\(A=-2a-5-2a+7=-4a+2\)
Vậy...
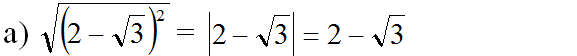
a) \(\sqrt{\left(2-\sqrt{3}\right)^2}\) = / 2 - \(\sqrt{3}\) / = 2 - \(\sqrt{3}\) ( vì 2 > \(\sqrt{3}\) )
b) \(\sqrt{\left(3-\sqrt{11}\right)^2}\) = / 3 - \(\sqrt{11}\) / = \(\sqrt{11}\) - 3 ( vì \(\sqrt{11}\) > 3 )
c) \(2\sqrt{a^2}\) = 2/a / = 2a (vì a> 0 )
d) \(3\sqrt{\left(a-2\right)^2}\) = 3 / a - 2 / = 3 ( 2 - a ) = 6 - 3a (vì a < 2 )