phân tích thành nhân tử\(4x^4+4x^3+5x^2+2x+1\)

phân tích thành nhân tử
\(4x^4+4x^3+5x^2+2x+1\)

K

Khách

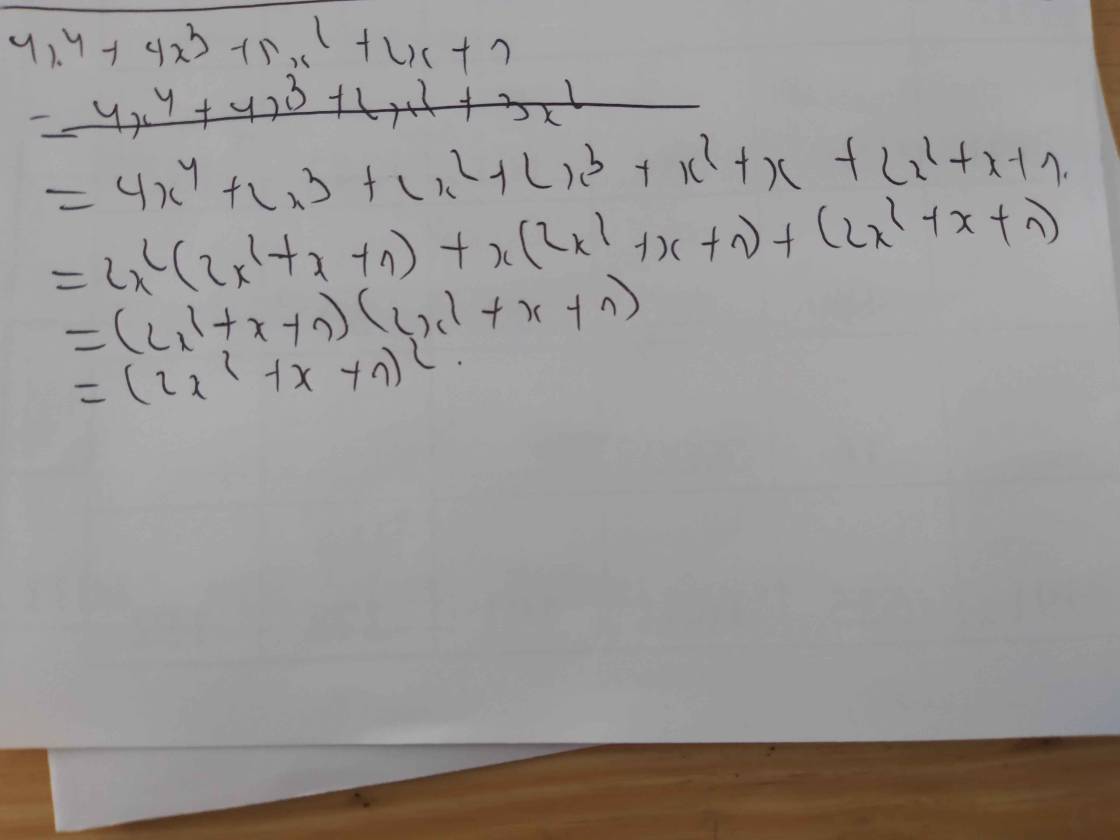
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Mua vip

HN

Học ngu lắm

18 tháng 10 2023

phân tích thành nhân tử
\(4x^4+4x^3+5x^2+2x+1\)

#Hỏi cộng đồng OLM #Toán lớp 8

1

NL

Nguyễn Lê Phước Thịnh

19 tháng 10 2023

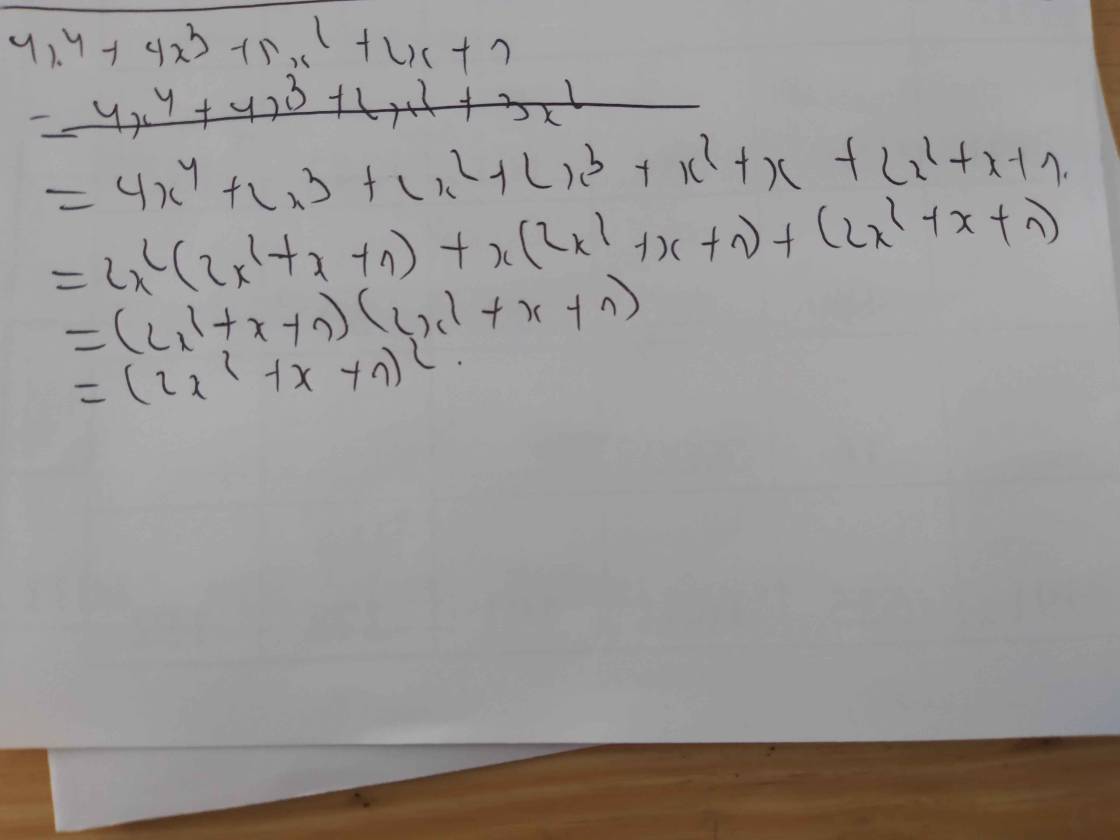
loading...

Các câu hỏi dưới đây có thể giống với câu hỏi trên

LA

Lan Anh Phạm

13 tháng 8 2017 - olm

Phân tích thành nhân tử

a)
\(x^3+2x^2y+xy^2-4x\)

b) \(x^4+4\)

#Hỏi cộng đồng OLM #Toán lớp 8

2

CN

Cô Nàng Bảo Bình

13 tháng 8 2017

a)x(x²+2xy+y²-4)

=x[(x+y)²-2² ]

=x(x+y-2)(x+y+2)

b)x⁴+4=x⁴+4x²+4-4x²=(x²+2)²-4x²

=(x²+2-2x)(x²+2+2x)

UN

uzumaki naruto

13 tháng 8 2017

\(x^3+2x^2y+xy^2-4x=x\)\(\left(x^2+2xy+y^2-4\right)\)

\(=x\left[\left(x+y\right)^2-4\right]\)

\(=x\left(x+y+2\right)\left(x+y-2\right)\)

\(x^4+4=x^4+4x^2+4-4x^2\)

\(=\left(x^2+2\right)^2-\left(2x\right)^2\)

\(=\left(x^2+2+2x\right)\left(x^2+2-2x\right)\)

NK

Nguyễn Khắc Quang

3 tháng 3 2021 - olm

Phân tích đa thức thành nhân tử:

a, \(4x^4+4x^3+5x^2+2x+1\)

b, \(3x^2+11x^3-7x^2-2x+1\)

#Hỏi cộng đồng OLM #Toán lớp 8

3

PT

Phạm Thành Đông

4 tháng 3 2021

a) \(4x^4+4x^3+5x^2+2x+1\)

= \(x^2\left(4x^2+4x+5+\frac{4}{x}+\frac{1}{x^2}\right)\)

=\(x^2\left[\left(4x^2+\frac{1}{x^2}\right)+2\left(2x+\frac{1}{x}\right)+5\right]\)(1)

Đặt \(2x+\frac{1}{x}=a\)thì \(\left(2x+\frac{1}{x}\right)^2=a^2\)\(\Rightarrow4x^2+\frac{1}{x^2}=a^2-4\)

Thay vào (1), ta có:

\(x^2\left(a^2-4+2a+5\right)\)

=\(x^2\left(a^2+2a+1\right)\)

=\(x^2\left(a+1\right)^2\)

=\(\left[x\left(a+1\right)\right]^2\)

=\(\left[x\left(2x+\frac{1}{x}+1\right)\right]^2\)

=\(\left(2x^2+1+x\right)^2\)

\(=\left(2x^2+x+1\right)^2\)

LD

l҉o҉n҉g҉ d҉z҉

3 tháng 3 2021

a) Đặt f(x) = 4x⁴ + 4x³ + 5x² + 2x + 1

Sau khi phân tích thì đa thức có dạng ( 2x² + ax + 1 )( 2x² + bx + 1 )

=> f(x) = ( 2x² + ax + 1 )( 2x² + bx + 1 )

<=> f(x) = 4x⁴ + 2bx³ + 2x² + 2ax³ + abx² + ax + 2x² + bx + 1

<=> f(x) = 4x⁴ + ( a + b )2x³ + ( ab + 4 )x² + ( a + b )x + 1

Đồng nhất hệ số ta có : \(\hept{\begin{cases}a+b=2\\ab=1\end{cases}\Leftrightarrow}a=b=1\)

Vậy f(x) = 4x⁴ + 4x³ + 5x² + 2x + 1 = ( 2x² + x + 1 )²

b) 3x⁴ + 11x³ - 7x² - 2x + 1

= 3x⁴ - x³ + 12x³ - 4x² - 3x² + x - 3x + 1

= x³( 3x - 1 ) + 4x²( 3x - 1 ) - x( 3x - 1 ) - ( 3x - 1 )

= ( 3x - 1 )( x³ + 4x² - x - 1 )

M

min_sone2003

19 tháng 8 2016 - olm

Phân tích thành nhân tử:
a) \(x^4+2x^3-4x-4\)

b) \(x^2.\left(1-x^2\right)-4-4x^2\)
c) \(\left(1+2x\right)\left(1-2x\right)-x\left(x+2\right)\left(x-2\right)\)
d) \(x^2+y^2-x^2y^2+xy-x-y\)
Giúp mình với nhé, mình cần gấp

#Hỏi cộng đồng OLM #Toán lớp 8

4

AN

alibaba nguyễn

19 tháng 8 2016

1/ (x² - 2)(x²+ 2x + 2)

AN

alibaba nguyễn

19 tháng 8 2016

2/ x²- (x²+ 2)²= (x - x²- 2)(x + x²+ 2)

DV

Đăng Văn Nghĩa

25 tháng 8 2021 - olm

Phân tích đa thức thành nhân tử dùng phương pháp nhóm các hạng tử:
1. \(x^2+x-y^2+y\)

2.\(4x^2-9y^2+4x-6y\)

3.\(x^2-9-5x-15\)

#Hỏi cộng đồng OLM #Toán lớp 8

1

DA

Diệu Anh

25 tháng 8 2021

1. x² + x - y² +y

= (x² -y²) + (x+y)

= (x-y)(x+y) + (x+y)

= (x+y)(x-y+1)

2. 4x² - 9y² + 4x -6y

= (2x)² -(3y)² + 2(2x - 3y)

= (2x -3y)(2x+3y) + 2(2x-3y)

= (2x-3y)(2x+3y+2)

3. x² - 9 - 5x - 15

= x² - 5x - 24

= x² - 8x + 3x -24

= x(x-8) + 3(x-8)

= (x-8)(x+3)

DV

Đăng Văn Nghĩa

26 tháng 8 2021 - olm

Phân tích đa thức thành nhân tử dùng phương pháp nhóm các hạng tử:
1.\(x^4+2x^3-4x-4\)

2.\(x^2-\left(a+b\right)x+ab\)

3.\(5xy^3-2xyz-15y^2+6z\)

#Hỏi cộng đồng OLM #Toán lớp 8

1

QA

Quỳnh Anh

26 tháng 8 2021

Trả lời:

1) sửa đề: \(x^4+x^3-4x-4=x^3\left(x+1\right)-4\left(x+1\right)=\left(x+1\right)\left(x^3-4\right)\)

2) \(x^2-\left(a+b\right)x+ab=x^2-ax-bx+ab=\left(x^2-ax\right)-\left(bx-ab\right)\)

\(=x\left(x-a\right)-b\left(x-a\right)=\left(x-a\right)\left(a-b\right)\)

3) \(5xy^3-2xyz-15y^2+6z=\left(5xy^3-15y^2\right)-\left(2xyz-6z\right)\)

\(=5y^2\left(xy-3\right)-2z\left(xy-3\right)=\left(xy-3\right)\left(5y^2-2z\right)\)

L

LIÊN

19 tháng 10 2016

1) phân tích đa thức thành nhân tử:
\(x^3-3x+1-3x^2\)

b) \(2x^2+4x+2-2y^2\)

#Hỏi cộng đồng OLM #Toán lớp 8

1

TV

Trần Việt Linh

19 tháng 10 2016

a) \(x^3-3x+1-3x^2=\left(x^3+1\right)-\left(3x^2+3x\right)=\left(x+1\right)\left(x^2-x+1\right)-3x\left(x+1\right)\)

\(=\left(x+1\right)\left(x^2-x+1-3x\right)=\left(x+1\right)\left(x^2-4x+1\right)\)

b) \(2x^2+4x+2-2y^2=2\left(x^2+2x+1-y^2\right)=2\left[\left(x+1\right)^2-y^2\right]=2\left(x+1+y\right)\left(x+1-y\right)\)

L

LIÊN

19 tháng 10 2016

bạn ơi giúp mink 1 câu nữa nhé

LP

Lê Phúc Thuận

8 tháng 10 2017 - olm

Phân tích đa thức thành nhân tử

\(x^4+4x^3+5x^2+2x+1\)

#Hỏi cộng đồng OLM #Toán lớp 8

3

TT

tuyett tuyet

8 tháng 10 2017

x⁴+4x³+5x²+2x+1 = x(x³+4x²+5x+2)+1

TT

tuyett tuyet

8 tháng 10 2017

Bút danh XXX

TT

Trang Thu

28 tháng 11 2016 - olm

Bài 1: Cho biểu thức A \(\frac{2}{2x-1}+\frac{4x^2+1}{4x^2-1}-\frac{1}{1+2x}\)= 1) Rút gọn biểu thức A2) Tính giá trị biểu thức A tại x = \(\frac{1}{2}\)3) Tìm x để A = 24) Tìm x nguyên để A nhận giá trị nguyên Bài 2: Phân tích đa thức thành nhân tử 1) \(x^2+4x-y^2+4\) 2)\(3x^2-5x-8\)3) \(x^3+x^2-4x+4\)4) \(16x^3+54y^3\)Bài 3: Cho hình chữ nhật ABCD có AB= 2AD. Vẽ BH vuông góc với AC. Gọi M,N,P lần lượt là...

#Hỏi cộng đồng OLM #Toán lớp 8

3

LM

Lionel Messi

7 tháng 12 2016

bài dễ như thế mà còn hỏi nữa

S

shitbo

1 tháng 1 2019

Câu 1:

\(Tacó\)

\(\frac{2}{2x-1}+\frac{4x^2+1}{4x^2-1}-\frac{1}{2x+1}=\frac{2}{2x-1}+\frac{4x^2+1}{\left(2x+1\right)\left(2x-1\right)}-\frac{1}{2x+1}\)

\(=\frac{4x+2}{\left(2x+1\right)\left(2x-1\right)}+\frac{4x^2+1}{\left(2x+1\right)\left(2x-1\right)}-\frac{2x-1}{\left(2x+1\right)\left(2x-1\right)}\)

\(=\frac{4x+2+4x^2+1-2x+1}{\left(2x+1\right)\left(2x-1\right)}=\frac{2x\left(2x+1\right)+4}{\left(2x+1\right)\left(2x-1\right)}=\frac{2x+4}{2x-1}\)

\(b,x=\frac{1}{2}\Rightarrow2x-1=0\left(loại\right)\)

..... 2 câu sau easy

N

NOOB

29 tháng 7 2020 - olm

Phân tích đa thức thành nhân tử
a) \(x^2-6x+4\)

b) \(x^2-4x+3\)

c) \(6x^2-5x+1\)

d) \(3x^2+13x-10\)

#Hỏi cộng đồng OLM #Toán lớp 8

1

NM

Nguyễn Minh Đăng

29 tháng 7 2020

Bài làm:

a) \(x^2-6x+4=\left(x^2-6x+9\right)-5=\left(x-3\right)^2-\left(\sqrt{5}\right)^2\)

\(=\left(x-3-\sqrt{5}\right)\left(x-3+\sqrt{5}\right)\)

b) \(x^2-4x+3=x^2-x-3x+3=\left(x-1\right)\left(x-3\right)\)

c) \(6x^2-5x+1=6x^2-3x-2x+1=\left(2x-1\right)\left(3x-1\right)\)

d) \(3x^2+13x-10=3x^2+15x-2x-10=\left(x-5\right)\left(3x-2\right)\)

NH

Nguyễn Hải Anh Jmg

5 tháng 7 2016

Phân tích các đa thức sau thành nhân tử bằng phương pháp dùng hằng đẳng thức:
a,\(x^2\)+4x-3
b,\(x^2\)+8x-9
c,\(3x^2\)+6x-9
d,\(2x^2\)+x-3

#Hỏi cộng đồng OLM #Toán lớp 8

2

HL

Hoàng Lê Bảo Ngọc

5 tháng 7 2016

a) \(x^2+4x+3=\left(x^2+4x+4\right)-1=\left(x+2\right)^2-1^2=\left(x+1\right)\left(x+3\right)\) (mình sửa lại)

b) \(x^2+8x-9=\left(x^2+8x+16\right)-25=\left(x+4\right)^2-5^2=\left(x-1\right)\left(x+9\right)\)

c) \(3x^2+6x-9=3\left[\left(x^2+2x+1\right)-4\right]=3\left[\left(x+1\right)^2-2^2\right]=3\left(x-1\right)\left(x+3\right)\)

d) \(2x^2+x-3=2x^2-4x+2+5x-5=2\left(x^2-2x+1\right)+5\left(x-1\right)=2\left(x-1\right)^2+5\left(x-1\right)=\left(x-1\right)\left(2x+3\right)\)

TT

Thiên Thảo

6 tháng 7 2016

tik nhé Toán lớp 8