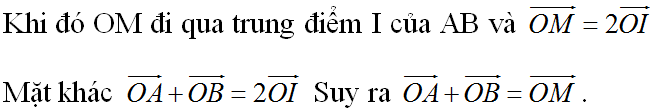Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

a: \(\overrightarrow{AM}+\overrightarrow{BN}=\dfrac{1}{2}\overrightarrow{AB}+\dfrac{1}{2}\overrightarrow{BC}=\dfrac{1}{2}\overrightarrow{AC}\)
b: \(=\dfrac{1}{2}\overrightarrow{AC}+\dfrac{1}{2}\overrightarrow{AC}+\dfrac{1}{2}\overrightarrow{BA}\)
\(=\overrightarrow{AC}+\dfrac{1}{2}\overrightarrow{BA}\)
c: \(\overrightarrow{AM}+\overrightarrow{BN}+\overrightarrow{CP}\)
\(=\dfrac{1}{2}\overrightarrow{AB}+\dfrac{1}{2}\overrightarrow{BC}+\dfrac{1}{2}\overrightarrow{CA}\)
\(=\dfrac{1}{2}\left(\overrightarrow{AC}+\overrightarrow{CA}\right)=\overrightarrow{0}\)

\(3\overrightarrow{AC}=2\overrightarrow{CB}\leftrightarrow3\overrightarrow{AO}+3\overrightarrow{OC}=2\overrightarrow{CO}+2\overrightarrow{OB}\)
\(\rightarrow5\overrightarrow{OC}=3\overrightarrow{OA}+2\overrightarrow{OB}\)
\(\rightarrow\overrightarrow{OC}=\dfrac{3}{5}\overrightarrow{OA}+\dfrac{2}{5}\overrightarrow{OB}\)

Gọi G là trọng tâm của \(\Delta\)ABC. Khi đó, với mỗi điểm O ta luôn có:
\(\overrightarrow{OA}+\overrightarrow{OB}+\overrightarrow{OC}=3\overrightarrow{OG}\). Suy ra \(3\overrightarrow{OG}=2\overrightarrow{CB}\)
Do G xác định nên ta có thể dựng điểm O sao cho: OG = 2/3.BC và \(\overrightarrow{OG}\uparrow\uparrow\overrightarrow{CB}\)như hình vẽ:
A B C G O

Ta có:
\(\overrightarrow{AM}=\dfrac{1}{2}\left(\overrightarrow{AO}+\overrightarrow{AB}\right)\)
\(\Leftrightarrow\overrightarrow{AM}=\dfrac{1}{2}\left(\overrightarrow{AO}+\overrightarrow{AO}+\overrightarrow{OB}\right)\)
\(\Leftrightarrow\overrightarrow{AM}=\dfrac{1}{2}\left(2\overrightarrow{AO}+\overrightarrow{OB}\right)\)
\(\Leftrightarrow\overrightarrow{AM}=\overrightarrow{AO}+\dfrac{1}{2}\overrightarrow{OB}\)
\(\Leftrightarrow\overrightarrow{AM}=\dfrac{1}{2}\overrightarrow{OB}-\overrightarrow{OA}\)
\(\RightarrowĐPCM\)
Câu b ) Bạn làm tương tự câu a , ta có vecto BN = 1/2 (BO +BC ) , rồi là như câu a
chúc bạn hok tốt