
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


Đặt `t=x-\sqrt3=>x=t+sqrt3( t\inZZ)`
Khi đó: `x^2+2sqrt3=(t+sqrt3)^2+2sqrt3=t^2+3+2sqrt3(t+1)\inZZ`
`=>t+1=0<=>t=-1`
`=>x=-1+sqrt3,` thử lại ta đều thấy `(sqrtx-3;x^2+2\sqrt3;x-2/x)\inZZ`
Vậy `x=-1+sqrt3`

a.
\(a+2b+3c=14\Rightarrow2a+4b+6c=28\)
\(P-28=a^2+b^2+c^2-2a-4b-6c\)
\(P-28=\left(a-1\right)^2+\left(b-2\right)^2+\left(c-3\right)^2-14\ge-14\)
\(\Rightarrow P\ge28-14=14\)
\(P_{min}=14\) khi \(\left(a;b;c\right)=\left(1;2;3\right)\)
b.
\(P^2=\left(a+b+c\right)^2=\left(1.a+\dfrac{1}{2}.2b+\dfrac{1}{3}.3c\right)^2\)
\(P^2\le\left(1+\dfrac{1}{4}+\dfrac{1}{9}\right)\left(a^2+4b^2+9c^2\right)=\dfrac{49}{36}.2015\)
\(\Rightarrow P\le\dfrac{7\sqrt{2015}}{6}\)
Dấu "=" xảy ra khi \(\left(a;b;c\right)=\left(\dfrac{6\sqrt{2015}}{7};\dfrac{3\sqrt{2015}}{4};\dfrac{2\sqrt{2015}}{21}\right)\)


a) Xét tứ giác ADHE có
\(\widehat{ADH}\) và \(\widehat{AEH}\) là hai góc đối
\(\widehat{ADH}+\widehat{AEH}=180^0\left(90^0+90^0=180^0\right)\)
Do đó: ADHE là tứ giác nội tiếp(Dấu hiệu nhận biết tứ giác nội tiếp)


BĐt phụ : \(\frac{a^2-ab+b^2}{a^2+ab+b^2}\ge\frac{1}{3}\)
c/m :\(3a^2-3ab+3b^2\ge a^2+ab+b^2\)
↔\(2a^2-4ab+2b^2\ge0\)
↔\(2\left(a-b\right)^2\ge0\)(luôn đúng)
Giải ;
ta có:\(\frac{a^3-b^3}{a^2+ab+b^2}+\frac{b^3-c^3}{b^2+bc+c^2}+\frac{c^3-a^3}{c^2+ac+a^2}=\left(a-b\right)+\left(b-c\right)+\left(c-a\right)=0\)
→\(\frac{a^3}{a^2+ab+b^2}+\frac{b^3}{b^2+bc+c^2}+\frac{c^3}{c^2+ca+a^2}=\frac{b^3}{a^2+ab+b^2}+\frac{c^3}{b^2+bc+c^2}+\frac{a^3}{c^2+ac+a^2}\)(1)
mà \(\frac{a^2-ab+b^2}{a^2+ab+b^2}\ge\frac{1}{3}\Leftrightarrow\frac{\left(a+b\right)\left(a^2-ab+b^2\right)}{a^2+ab+b^2}\ge\frac{1}{3}\left(a+b\right)\)
↔\(\frac{a^3+b^3}{a^2+ab+b^2}\ge\frac{1}{3}\left(a+b\right)\)
tương tự ta có:\(\frac{b^3+c^3}{b^2+bc+c^2}\ge\frac{1}{3}\left(b+c\right)\);\(\frac{c^3+a^3}{c^2+ca+a^2}\ge\frac{1}{3}\left(a+c\right)\)
cộng vế vs vế ta có:
\(\frac{a^3}{a^2+ab+b^2}+\frac{b^3}{a^2+ab+b^2}+\frac{b^3}{b^2+bc+c^2}+\frac{c^3}{b^2+bc+c^2}+\frac{c^3}{c^2+ca+a^2}+\frac{a^3}{c^2+ac+a^2}\ge\frac{2}{3}\left(a+b+c\right)\)
từ (1)→\(2\left(\frac{a^3}{a^2+ab+b^2}+\frac{b^3}{b^2+bc+c^2}+\frac{c^3}{c^2+ca+a^2}\right)\ge\frac{2}{3}\left(a+b+c\right)\)
↔ \(S\ge\frac{1}{3}\left(a+b+c\right)=1\)(đặt S luôn cho tiện)
dấu = xảy ra khi BĐt ở đầu đúng :\(\begin{cases}a=b\\b=c\\c=a\end{cases}\)mà a+b+c=3↔a=b=c=1

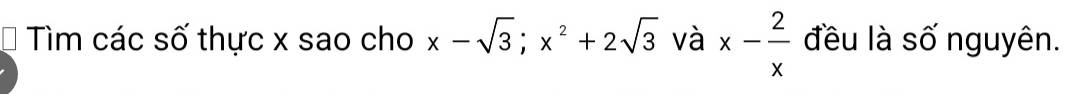 nhờ mọi người giúp vs ạ.
nhờ mọi người giúp vs ạ.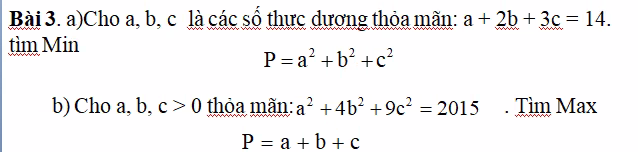





 Mọi người giúp e làm câu 4c và bài 5 ạ
Mọi người giúp e làm câu 4c và bài 5 ạ
