K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
AH
Akai Haruma
Giáo viên
7 tháng 10 2023
Bài 1:
Vận tốc cano khi dòng nước lặng là: $25-2=23$ (km/h)
AH
Akai Haruma
Giáo viên
7 tháng 10 2023
Bài 2:
Đổi 1 giờ 48 phút = 1,8 giờ
Độ dài quãng đường AB: $1,8\times 25=45$ (km)
Vận tốc ngược dòng là: $25-2,5-2,5=20$ (km/h)
Cano ngược dòng từ B về A hết:
$45:20=2,25$ giờ = 2 giờ 15 phút.
AH
Akai Haruma
Giáo viên
4 tháng 9 2021
Bài 1:
a.
$a^3-a^2c+a^2b-abc=a^2(a-c)+ab(a-c)$
$=(a-c)(a^2+ab)=(a-c)a(a+b)=a(a-c)(a+b)$
b.
$(x^2+1)^2-4x^2=(x^2+1)^2-(2x)^2=(x^2+1-2x)(x^2+1+2x)$
$=(x-1)^2(x+1)^2$
c.
$x^2-10x-9y^2+25=(x^2-10x+25)-9y^2$
$=(x-5)^2-(3y)^2=(x-5-3y)(x-5+3y)$
d.
$4x^2-36x+56=4(x^2-9x+14)=4(x^2-2x-7x+14)$
$=4[x(x-2)-7(x-2)]=4(x-2)(x-7)$
AH
Akai Haruma
Giáo viên
4 tháng 9 2021
Bài 2:
a. $(3x+4)^2-(3x-1)(3x+1)=49$
$\Leftrightarrow (3x+4)^2-[(3x)^2-1]=49$
$\Leftrightarrow (3x+4)^2-(3x)^2=48$
$\Leftrightarrow (3x+4-3x)(3x+4+3x)=48$
$\Leftrightarrow 4(6x+4)=48$
$\Leftrightarrow 6x+4=12$
$\Leftrightarrow 6x=8$
$\Leftrightarrow x=\frac{4}{3}$
b. $x^2-4x+4=9(x-2)$
$\Leftrightarrow (x-2)^2=9(x-2)$
$\Leftrightarrow (x-2)(x-2-9)=0$
$\Leftrightarrow (x-2)(x-11)=0$
$\Leftrightarrow x-2=0$ hoặc $x-11=0$
$\Leftrightarrow x=2$ hoặc $x=11$
c.
$x^2-25=3x-15$
$\Leftrightarrow (x-5)(x+5)=3(x-5)$
$\Leftrightarrow (x-5)(x+5-3)=0$
$\Leftrightarrow (x-5)(x+2)=0$
$\Leftrightarrow x-5=0$ hoặc $x+2=0$
$\Leftrightarrow x=5$ hoặc $x=-2$
HV
14 tháng 11 2017
Áp dụng phương pháp hệ số bất định ta có
x4-6x3+12x2-14x+3
= (x2+ax+b)(x2+cx+d)
= x4 + (a+c)x3+(ac+b+d)x2+(ad+bc)x+bd
Đồng nhất đa thức trên với đề bài ta có
\(\left[{}\begin{matrix}a+c=-6\\ac+b+d=12\\ad+bc=-14\\bd=3\end{matrix}\right.\)
\(\Rightarrow\left[{}\begin{matrix}a=-2\\b=3\\c=-4\\d=1\end{matrix}\right.\)
Thế a,b,c,d ta được
x4-6x3+12x2-14x+3
= (x2+ax+b)(x2+cx+d)
= (x2-2x+3)(x2-4x+1)
HR
14 tháng 11 2017
Bài 2
1/ \(\dfrac{x-342}{15}+\dfrac{x-323}{17}+\dfrac{x-300}{19}+\dfrac{x-273}{21}=10\)
\(\Leftrightarrow\dfrac{x-342}{15}-1+\dfrac{x-323}{17}-2+\dfrac{x-300}{19}-3+\dfrac{x-273}{21}-4=0\)\(\Leftrightarrow\dfrac{x-357}{15}+\dfrac{x-357}{17}+\dfrac{x-357}{19}+\dfrac{x-357}{21}=0\)
\(\Leftrightarrow\left(x-357\right)\left(\dfrac{1}{15}+\dfrac{1}{17}+\dfrac{1}{19}+\dfrac{1}{21}\right)=0\)
Mà \(\dfrac{1}{15}+\dfrac{1}{17}+\dfrac{1}{19}+\dfrac{1}{21}>0\)
\(\Rightarrow x-357=0\Leftrightarrow x=357\)
2/ Ta có: \(\left(x-y\right)^2+\left(y-z\right)^2+\left(z-x\right)^2\ge0\)
\(\Leftrightarrow x^2+y^2+z^2-xy-yz-zx\ge0\)
\(\Leftrightarrow\left(x+y+z\right)^2\ge3\left(xy+yz+zx\right)\)
\(\Leftrightarrow\dfrac{\left(x+y+z\right)^2}{3}\ge xy+yz+zx\)
\(\Leftrightarrow xy+yz+zx\le3\)
\(\Rightarrow\) GTLN của B là 3
Dấu ''='' xảy ra khi và chỉ khi \(x=y=z=1\)
NC
29 tháng 10 2017
a.) \\(\\left(a+b+c\\right)^3-a^3-b^3-c^3\\)
\\(=a^3+b^3+c^3+3a^2b+3ab^2+3a^2c+3ac^2+3b^2c+3bc^2+6abc-a^3-b^3-c^3\\)\\(=3\\left(3a^2b+3ab^2+3a^2c+3ac^2+3b^2c+3bc^2+6abc\\right)\\)
\\(=3\\left(abc+a^2b+a^2c+ac^2+b^2c+ab^2+abc+bc^2\\right)\\)
\\(=3\\left[ab\\left(a+c\\right)+ac\\left(a+c\\right)+b^2\\left(a+c\\right)+bc\\left(a+c\\right)\\right]\\)
\\(=3\\left(a+c\\right)\\left(ab+ac+bc+b^2\\right)\\)
\\(=3\\left(a+c\\right)\\left[a\\left(b+c\\right)+b\\left(b+c\\right)\\right]\\)
\\(=3\\left(a+c\\right)\\left(a+b\\right)\\left(b+c\\right)\\)
b) 4a2b2-(a2 +b2-c2)2
=（2ab+a2+b2-c2)(2ab-a2-b2+c2）
=[(a+b)2-c2][c2-(a-b)2]
=(a+b+c)(a+b-c)(c+a-b)(c-a+b)
AN
30 tháng 10 2017
a) \(\left(a+b+c\right)^3-a^3-b^3-c^3\)
\(=a^3+b^3+c^3+3ab\left(a+b\right)+3bc\left(b+c\right)+3ca\left(c+a\right)+6abc-a^3-b^3-c^3\)
\(=3ab\left(a+b\right)+3bc\left(b+c\right)+3ca\left(c+a\right)+6abc\)
\(=3\left(ab\left(a+b\right)+bc\left(b+c\right)+ca\left(c+a\right)+2abc\right)\)
\(=3\left(ab\left(a+b\right)+b^2c+abc+bc^2+c^2a+ca^2+abc\right)\)
\(=3\left(ab\left(a+b\right)+bc\left(a+b\right)+c^2\left(a+b\right)+ac\left(a+b\right)\right)\)
\(=3\left(a+b\right)\left(ab+bc+c^2+ac\right)\)
\(=3\left(a+b\right)\left[b\left(a+c\right)+c\left(a+c\right)\right]\)
\(=3\left(a+b\right)\left(a+c\right)\left(b+c\right)\)
2 tháng 6 2022
Câu 4:
a: ĐKXĐ: \(x\notin\left\{0;-5\right\}\)
b: \(A=\dfrac{x^2+2x}{2\left(x+5\right)}+\dfrac{x-5}{x}+\dfrac{50-5x}{2x\left(x+5\right)}\)
\(=\dfrac{x^3+2x^2}{2x\left(x+5\right)}+\dfrac{2\left(x^2-25\right)}{2x\left(x+5\right)}+\dfrac{50-5x}{2x\left(x+5\right)}\)
\(=\dfrac{x^3+2x^2+2x^2-50+50-5x}{2x\left(x+5\right)}\)
\(=\dfrac{x^3+4x^2-5x}{2x\left(x+5\right)}=\dfrac{x\left(x^2+4x-5\right)}{2x\left(x+5\right)}\)
\(=\dfrac{x\left(x+5\right)\left(x-1\right)}{2x\left(x+5\right)}=\dfrac{x-1}{2}\)
c: Để A=-3 thì x-1=-6
hay x=-5(loại)
giúp mk nha
14 tháng 9 2017
Điều kiện:
\(x-1\ne0\Rightarrow x\ne1\)
\(x^3+x\ne0\Leftrightarrow x\ne0\)
NC
22 tháng 10 2017
Đặt tính \(2n^2-n+2\) : \(2n+1\) sẽ bằng n - 1 dư 3
Để chia hết thì 3 phải chia hết cho 2n + 1 hay 2n + 1 là ước của 3
Ư(3) = {\(\pm\) 3; \(\pm\) 1}
\(2n+1=1\Leftrightarrow2n=0\Leftrightarrow n=0\)
\(2n+1=-1\Leftrightarrow2n=-2\Leftrightarrow n=-1\)
\(2n+1=3\Leftrightarrow2n=2\Leftrightarrow n=1\)
\(2n+1=-3\Leftrightarrow2n=-4\Leftrightarrow n=-2\)
Vậy \(n=\left\{0;-2;\pm1\right\}\)
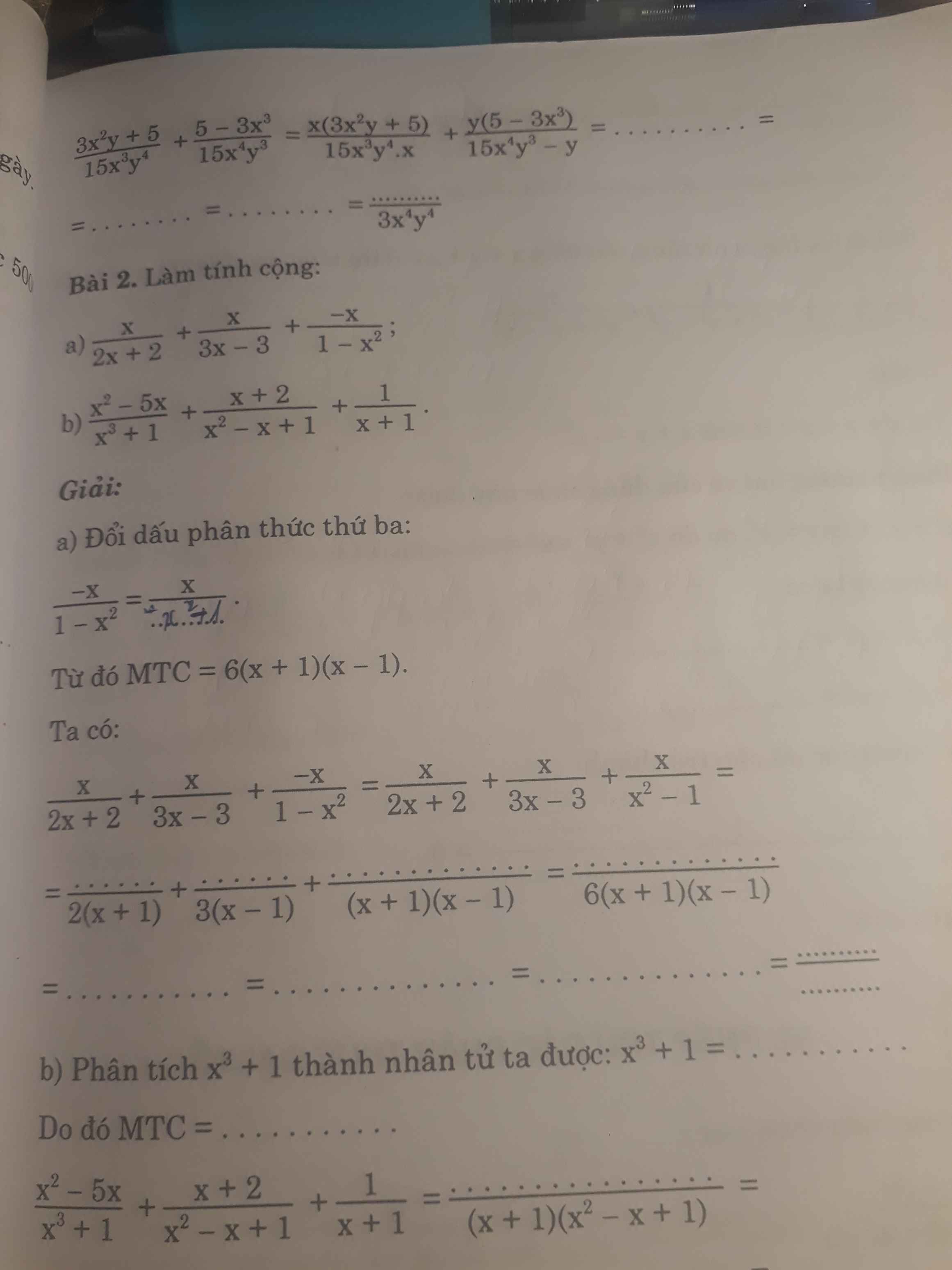
2:
a)x / 2x+2 + x / 3x-3 + -x / 1-x2
= x / 2(x+1) + x / 3(x-1) + x / x2-1
= x(x-1) / 2(x+1)(x-1) + x(x+1) / 3(x-1)(x+1) + x / (x-1)(x+1)
= 3x(x-1) / 6(x+1)(x-1) + 2x(x+1) / 6(x+1)(x-1) + 6x / 6(x+1)(x-1)
= 3x(x-1) + 2x(x+1) + 6x / 6(x+1)(x-1)
= 3(x2-x) + 2(x2+x) + 6x / 6(x+1)(x-1)
= 3x2 - 3x + 2x2 + 2x + 6x / 6(x+1)(x-1)
= 5x2 + 5x / 6(x+1)(x-1)
= 5x(x+1) / 6(x+1)(x-1)
= 5x / 6(x-1) = 5(x-1) + 5 / 6(x-1)
= 5(x-1) / 6(x-1) + 5 / 6(x-1)
= 5/6 + 5 / 6(x-1)
b)x2-5x / x3+1 + x+2 / x2-x+1 + 1 / x+1
= x2-5x / x3+1 + (x+2)(x+1)+x2-x+1 / (x2-x+1)(x+1)
= x2-5x / x3+1 + x2+3x+2+x2-x+1 / x3+1
= x2-5x+x2+3x+2+x2-x+1 / x3+1
= 3x2-3x+3 / (x2-x+1)(x+1)
= 3(x2-x+1) / (x2-x+1)(x+1) = 3 / x+1