HB
3
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
H
2
3 tháng 4 2018
a) \(|2x+1|=|x-3|\)
\(\Leftrightarrow|2x+1|-|x-3|=0\)
Lập bảng xét dấu :
| x | \(\frac{-1}{2}\) | 3 | |||
| 2x+1 | - | 0 | + | \(|\) | + |
| x-3 | - | \(|\) | - | 0 | + |
Nếu \(x< \frac{-1}{2}\) thì \(|2x+1|=-2x-1\)
\(|x-3|=3-x\)
\(pt\Leftrightarrow\left(-2x-1\right)-\left(3-x\right)=0\)
\(\Leftrightarrow-2x-1-3+x=0\)
\(\Leftrightarrow-x=4\)
\(\Leftrightarrow x=-4\left(tm\right)\)
Nếu \(\frac{-1}{2}\le x\le3\) thì \(|2x+1|=2x+1\)
\(|x-3|=3-x\)
\(pt\Leftrightarrow\left(2x+1\right)-\left(3-x\right)=0\)
\(\Leftrightarrow2x+1-3+x=0\)
\(\Leftrightarrow3x-2=0\)
\(x=\frac{2}{3}\left(tm\right)\)
Nếu \(x>3\) thì \(|2x+1|=2x+1\)
\(|x-3|=x-3\)
\(pt\Leftrightarrow\left(2x+1\right)-\left(x-3\right)=0\)
\(\Leftrightarrow2x+1-x+3=0\)
\(\Leftrightarrow x=-4\) ( loại )
3 tháng 4 2018
\(x^4+x^2+6x-8=0\)
\(\Leftrightarrow\left(x^4+2x^2+1\right)-\left(x^2-6x+9\right)=0\)
\(\Leftrightarrow\left(x^2+1\right)^2-\left(x-3\right)^2=0\)
Mà \(\left(x^2+1\right)^2\ge0\forall x\)
\(\left(x-3\right)^2\ge0\forall x\)
Dấu bằng xảy ra khi :
\(\hept{\begin{cases}x^2+1=0\\x-3=0\end{cases}}\Leftrightarrow\hept{\begin{cases}x^2=-1\\x=3\end{cases}}\)
Lại có \(x^2\ge0\forall x\)
\(\Leftrightarrow x^2=-1\) ( vô lí )
Vậy phương trình có tập nghiệm \(S=\left\{3\right\}\)
19 tháng 5 2017
chẳng ai giải, thôi mình giải vậy!
a) Đặt \(y=x^2+4x+8\),phương trình có dạng:
\(t^2+3x\cdot t+2x^2=0\)
\(\Leftrightarrow t^2+xt+2xt+2x^2=0\)
\(\Leftrightarrow t\left(t+x\right)+2x\left(t+x\right)=0\)
\(\Leftrightarrow\left(2x+t\right)\left(t+x\right)=0\)
\(\Leftrightarrow\left(2x+x^2+4x+8\right)\left(x^2+4x+8+x\right)=0\)
\(\Leftrightarrow\orbr{\begin{cases}x=-2\\x=-4\end{cases}}\)vậy tập nghiệm của phương trình là:S={-2;-4}
b) nhân 2 vế của phương trình với 12 ta được:
\(\left(6x+7\right)^2\left(6x+8\right)\left(6x+6\right)=72\)
Đặt y=6x+7, ta được:\(y^2\left(y+1\right)\left(y-1\right)=72\)
giải tiếp ra ta sẽ được S={-2/3;-5/3}
c) \(\left(x-2\right)^4+\left(x-6\right)^4=82\)
S={3;5}
d)s={1}
e) S={1;-2;-1/2}
f) phương trình vô nghiệm
23 tháng 1 2020
1) 2x4 - 9x3 + 14x2 - 9x + 2 = 0
<=> (2x4 - 4x3) - (5x3 - 10x2) + (4x2 - 8x) - (x - 2) = 0
<=> 2x3(x - 2) - 5x2(x - 2) + 4x(x - 2) - (x - 2) = 0
<=> (2x3 - 5x2 + 4x - 1)(x - 2) = 0
<=> [(2x3 - 2x2) - (3x2 - 3x) + (x - 1)](x - 2) = 0
<=> [2x2(x - 1) - 3x(x - 1) + (x - 1)](x - 2) = 0
<=> (2x2 - 2x - x + 1)(x - 1)(x - 2) = 0
<=> (2x - 1)(x - 1)2(x - 2) = 0
<=> 2x - 1=0
hoặc x - 1 = 0
hoặc x - 2 = 0
<=> x = 1/2
hoặc x = 1
hoặc x = 2
Vậy S = {1/2; 1; 2}
3 tháng 4 2020
a) Ta thấy x - 1 \(\ne\)0 vì x = 1 không nghiệm đúng phương trình
Nhân hai vế của phương trình với x - 1 \(\ne\)0 ta được x5 -1 = 0 hay x = 1 ,không thỏa mãn điều kiện trên .
Vậy phương trình vô nghiệm .
b) Ta có : \(\frac{\left(x-2\right)^2}{3}-\frac{\left(2.x-3\right).\left(2.x+3\right)}{8}+\frac{\left(x-4\right)^2}{6}\)
<=> 3.(x-2)2 - 3. ( 2.x - 3 ) . ( 2.x + 3 )+ 4. ( x-4 )2 = 0
<=> 3. ( x - 4.x + 4 ) - 3. ( 4.x2 -9 ) + 4. ( x2 -8.x + 16 ) = 0
<=> -5.x2 -44.x + 103 = 0
<=> \(\orbr{\begin{cases}x=\frac{-22+3\sqrt{111}}{5}\\x=\frac{-22-3\sqrt{111}}{5}\end{cases}}\)
Vậy \(\orbr{\begin{cases}x=\frac{-22+3\sqrt{111}}{5}\\x=\frac{-22-3\sqrt{111}}{5}\end{cases}}\)
3 tháng 4 2020
a) Ta thấy x - 1 \(\ne\)0 vì x = 1 không nghiệm đúng phương trình
Nhân hai vế của phương trình với x - 1 \(\ne\)0 ta được x5 -1 = 0 hay x = 1 ,không thỏa mãn điều kiện trên .
Vậy phương trình vô nghiệm .
b) Ta có : \(\frac{\left(x-2\right)^2}{3}-\frac{\left(2.x-3\right).\left(2.x+3\right)}{8}+\frac{\left(x-4\right)^2}{6}\)
<=> 3.(x-2)2 - 3. ( 2.x - 3 ) . ( 2.x + 3 )+ 4. ( x-4 )2 = 0
<=> 3. ( x - 4.x + 4 ) - 3. ( 4.x2 -9 ) + 4. ( x2 -8.x + 16 ) = 0
<=> -5.x2 -44.x + 103 = 0
<=> \(\orbr{\begin{cases}x=\frac{-22+3\sqrt{111}}{5}\\x=\frac{-22-3\sqrt{111}}{5}\end{cases}}\)
Vậy \(\orbr{\begin{cases}x=\frac{-22+3\sqrt{111}}{5}\\x=\frac{-22-3\sqrt{111}}{5}\end{cases}}\)
20 tháng 4 2018
a/ Đặt \(\hept{\begin{cases}\frac{x+1}{x-2}=a\\\frac{x+1}{x-4}=b\end{cases}}\) thì có
\(a^2+b-\frac{12b^2}{a^2}=0\)
\(\Leftrightarrow\left(a^2-3b\right)\left(a^2+4b\right)=0\)
b/ \(2x^2+3xy-2y^2=7\)
\(\Leftrightarrow\left(2x-y\right)\left(x+2y\right)=7\)
21 tháng 3 2021
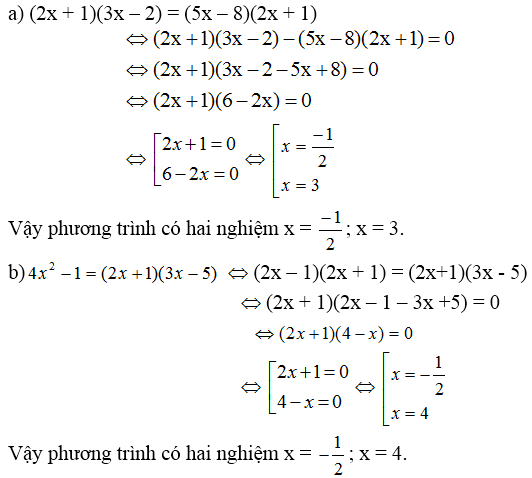
a)(2x+1)(3x-2)=(5x-8)(2x+1)
⇔(2x+1)(3x-2)-(5x-8)(2x+1)=0
⇔(2x+1)(3x-2-5x+8)=0
⇔(2x+1)(-2x+6)=0
⇔2x+1=0 hoặc -2x+6=0
1.2x+1=0⇔2x=-1⇔x=-1/2
2.-2x+6=0⇔-2x=-6⇔x=3
phương trình có 2 nghiệm x=-1/2 và x=3
31 tháng 12 2019
a) Ta có: x4 - x3 + 2x2 - x + 1 = 0
=> (x4 + 2x2 + 1) - x(x2 + 1) = 0
=> (x2 + 1)2 - x(x2 + 1) = 0
=> (x2 + 1)(x2 - x + 1) = 0
=> (x2 + 1)[(x2 - x + 1/4) + 3/4] = 0
=> (x2+ 1 )[(x - 1/2)2 + 3/4] = 0
=> pt vô nghiệm (vì x2 + 1 > 0; (x - 1/2)2 + 3/4 > 0)
b) Ta có: x3 + 2x2 - 7x + 4 = 0
=> (x3 - x) + (2x2 - 6x + 4) = 0
=> x(x2 - 1) + 2(x2 - 3x + 2) = 0
=> x(x - 1)(x + 1) + 2(x2 - 2x - x + 2) = 0
=> x(x - 1)(x + 1) + 2(x - 2)(x - 1) = 0
=> (x - 1)(x2 + x + 2x - 4) = 0
=> (x - 1)(x2 + 3x - 4) = 0
=> (x - 1)(x2 + 4x - x - 4) = 0
=> (x - 1)(x + 4)(x - 1) = 0
=> (x - 1)2(x + 4) = 0
=> \(\orbr{\begin{cases}x-1=0\\x+4=0\end{cases}}\)
=> \(\orbr{\begin{cases}x=1\\x=-4\end{cases}}\)
A
1 tháng 1 2020
a) \(x^4-x^3+2x^2-x+1=0\)
\(\Leftrightarrow\left(x^4+2x^2+1\right)-x\left(x^2+1\right)=0\)
\(\Leftrightarrow\left(x^2+1\right)^2-x\left(x^2+1\right)=0\)
\(\Leftrightarrow\left(x^2+1\right)\left(x^2+1-x\right)=0\)
\(\Leftrightarrow\left(x^2+1\right)\left[\left(x^2-x+\frac{1}{4}\right)+\frac{3}{4}\right]=0\)
\(\Leftrightarrow\left(x^2+1\right)\left[\left(x-\frac{1}{2}\right)^2+\frac{3}{4}\right]=0\)
Ta có: \(\hept{\begin{cases}x^2+1>0\forall x\\\left(x-\frac{1}{2}\right)^2+\frac{3}{4}>0\forall x\end{cases}}\)
\(\Rightarrow\)Phương trình vô nghiệm
Vậy không có giá trị x thỏa mãn đề bài
b) \(x^3+2x^2-7x+4=0\)
\(\Leftrightarrow\left(x^3-x\right)+\left(2x^2-6x+4\right)=0\)
\(\Leftrightarrow x\left(x^2-1\right)+2\left(x^2-3x+2\right)=0\)
\(\Leftrightarrow x\left(x-1\right)\left(x+1\right)+2\left(x^2-x-2x+2\right)=0\)
\(\Leftrightarrow x\left(x-1\right)\left(x+1\right)+2\left[x\left(x-1\right)-2\left(x-1\right)\right]=0\)
\(\Leftrightarrow x\left(x-1\right)\left(x+1\right)+2\left(x-2\right)\left(x-1\right)=0\)
\(\Leftrightarrow\left(x-1\right)\left[x\left(x+1\right)+2\left(x-2\right)\right]=0\)
\(\Leftrightarrow\left(x-1\right)\left[x^2+x+2x-4\right]=0\)
\(\Leftrightarrow\left(x-1\right)\left[x^2+3x-4\right]=0\)
\(\Leftrightarrow\left(x-1\right)\left[x^2+4x-x-4\right]=0\)
\(\Leftrightarrow\left(x-1\right)\left[x\left(x+4\right)-\left(x+4\right)\right]=0\)
\(\Leftrightarrow\left(x-1\right)^2\left(x+4\right)=0\)
\(\Leftrightarrow\orbr{\begin{cases}\left(x-1\right)^2=0\\x+4=0\end{cases}\Leftrightarrow\orbr{\begin{cases}x-1=0\\x+4=0\end{cases}\Leftrightarrow}\orbr{\begin{cases}x=1\\x=-4\end{cases}}}\)
Vậy x=1; x=-4
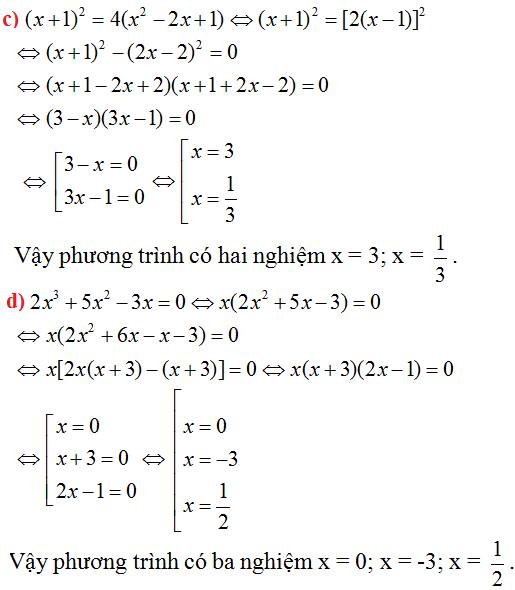
<=> (x^8-2x^4+1)+(x^2-2x+1)=0
<=>(x^4-1)^2+(x-1)^2=0
<=>\(\hept{\begin{cases}x^4-1=0\\x-1=0\end{cases}}\) <=> x=1
Chúc bạn học tốt :">
\(x^8-2x^4+x^2-2x+2=0\)
\(\left[\left(x^4\right)^2-2.x^4.1+1^2\right]+\left(x^2-2x+1\right)=0\)
\(\left(x^4-1\right)^2+\left(x-1\right)^2=0\)
Ta có: \(\hept{\begin{cases}\left(x^4-1\right)^2\ge0\forall x\\\left(x-1\right)^2\ge0\forall x\end{cases}}\Rightarrow\left(x^4-1\right)^2+\left(x-1\right)^2\ge0\forall x\)
Mà \(\left(x^4-1\right)^2+\left(x-1\right)^2=0\)
\(\Rightarrow\hept{\begin{cases}\left(x^4-1\right)^2=0\\\left(x-1\right)^2=0\end{cases}}\Leftrightarrow\hept{\begin{cases}x^4-1=0\\x-1=0\end{cases}\Leftrightarrow\hept{\begin{cases}x=\pm1\\x=1\end{cases}\Rightarrow}x=1}\)
Vậy \(x=1\)