
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


b:
ĐKXĐ: \(\left\{{}\begin{matrix}cosx< >0\\sinx< >0\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}x< >\dfrac{\Omega}{2}+k\Omega\\x\ne k\Omega\end{matrix}\right.\)
=>\(x\ne\dfrac{\Omega}{2}+\dfrac{k\Omega}{2}\)
\(\dfrac{1}{cosx}+\dfrac{\sqrt{3}}{sinx}=2\cdot sin\left(x+\dfrac{\Omega}{3}\right)\)
=>\(\dfrac{sinx+\sqrt{3}\cdot cosx}{cosx\cdot sinx}=2\cdot sin\left(x+\dfrac{\Omega}{3}\right)\)
=>\(\dfrac{sinx+\sqrt{3}\cdot cosx}{cosx\cdot sinx}=2\cdot\left[sinx\cdot\cos\dfrac{\Omega}{3}+sin\left(\dfrac{\Omega}{3}\right)\cdot cosx\right]\)
=>\(\dfrac{sinx+\sqrt{3}\cdot cosx}{cosx\cdot sinx}=2\cdot\left(\dfrac{1}{2}\cdot sinx+\dfrac{\sqrt{3}}{2}\cdot cosx\right)\)
=>\(\left(sinx+\sqrt{3}\cdot cosx\right)\left(\dfrac{1}{cosx\cdot sinx}-1\right)=0\)
=>\(2\cdot\left(sinx\cdot\dfrac{1}{2}+\dfrac{\sqrt{3}}{2}\cdot cosx\right)\cdot\left(\dfrac{2}{2\cdot sinx\cdot cosx}-1\right)=0\)
=>\(2\cdot sin\left(x+\dfrac{\Omega}{3}\right)\cdot\left(\dfrac{2}{sin2x}-1\right)=0\)
=>\(\left[{}\begin{matrix}sin\left(x+\dfrac{\Omega}{3}\right)=0\\\dfrac{2}{sin2x}-1=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x+\dfrac{\Omega}{3}=k\Omega\\sin2x=2\left(loại\right)\end{matrix}\right.\)
=>\(x=-\dfrac{\Omega}{3}+k\Omega\)

\(sin^2x-2m.sinx.cosx-sinx.cosx+2mcos^2x=0\)
\(\Leftrightarrow sinx\left(sinx-cosx\right)-2mcosx\left(sinx-cosx\right)=0\)
\(\Leftrightarrow\left(sinx-cosx\right)\left(sinx-2m.cosx\right)=0\)
\(\Rightarrow\left[{}\begin{matrix}sinx=cosx\\sinx=2m.cosx\end{matrix}\right.\)
\(\Rightarrow\left[{}\begin{matrix}tanx=1\\tanx=2m\end{matrix}\right.\)
Do \(tanx=1\) ko có nghiệm đã cho nên \(tanx=2m\) phải có nghiệm trên khoảng đã cho
\(\Rightarrow tan\left(\dfrac{\pi}{4}\right)< 2m< tan\left(\dfrac{\pi}{3}\right)\)
\(\Rightarrow1< 2m< \sqrt[]{3}\)
\(\Rightarrow m\in\left(\dfrac{1}{2};\dfrac{\sqrt{3}}{2}\right)\) (hoặc có thể 1 đáp án là tập con của tập này cũng được)

Điều kiện:
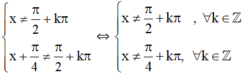
⇔ tan x.(1 - tanx) + tanx + 1 = 1 – tan x.
⇔ tan x - tan2x + 2.tan x = 0
⇔ tan2x - 3tanx = 0
⇔ tanx(tanx - 3) = 0
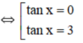
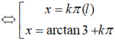
Vậy phương trình đã cho có tập nghiệm là:
{arctan 3+kπ; k ∈ Z }

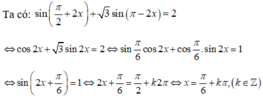

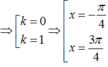
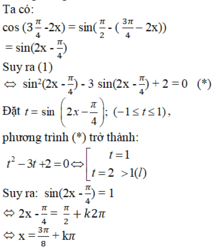

\(2sinx-1=0\Leftrightarrow sinx=\dfrac{1}{2}\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{\pi}{6}+k2\pi\\x=\dfrac{5\pi}{6}+k2\pi\end{matrix}\right.\)
Do \(x\in\left(-\dfrac{\pi}{2};\pi\right)\Rightarrow x=\left\{\dfrac{\pi}{6};\dfrac{5\pi}{6}\right\}\)