K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
NT
1
HP
4 tháng 2 2021
ĐK: \(x\ge-1\)
\(\sqrt{2x+7}-\sqrt{x+1}=2\)
\(\Leftrightarrow\sqrt{2x+7}=2+\sqrt{x+1}\)
\(\Leftrightarrow3x+7=4+4\sqrt{x+1}+x+1\)
\(\Leftrightarrow x+1=2\sqrt{x+1}\)
\(\Leftrightarrow\left\{{}\begin{matrix}\left(x+1\right)^2=4\left(x+1\right)\\x+1\ge0\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x^2-2x-3=0\\x\ge-1\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=3\left(tm\right)\\x=-1\left(tm\right)\end{matrix}\right.\)
15 tháng 7 2023
1) \(\sqrt[]{3x+7}-5< 0\)
\(\Leftrightarrow\sqrt[]{3x+7}< 5\)
\(\Leftrightarrow3x+7\ge0\cap3x+7< 25\)
\(\Leftrightarrow x\ge-\dfrac{7}{3}\cap x< 6\)
\(\Leftrightarrow-\dfrac{7}{3}\le x< 6\)
HT
1
7 tháng 5 2016
Viết lại phương trình dưới dạng :
\(4^{x^2-3x+2}+4^{2x^2+6x+5}=4^{x^2-3x+2}.4^{2x^2+6x+5}+1\)
Đặt \(\begin{cases}u=4^{x^2-3x+2}\\v=4^{2x^2+6x+5}\end{cases}\)\(;u,v>0\)
Khi đó phương trình tương đương với :
\(u+v=uv+1\Leftrightarrow\left(u-1\right)\left(1-v\right)=0\)
\(\Leftrightarrow\left[\begin{array}{nghiempt}u=1\\v=1\end{array}\right.\)\(\Leftrightarrow\left[\begin{array}{nghiempt}4^{x^2-3x+2}=1\\4^{2x^2+6x+5}=1\end{array}\right.\)\(\Leftrightarrow\left[\begin{array}{nghiempt}x^2-3x+2=0\\2x^2+6x+5=0\end{array}\right.\)\(\Leftrightarrow\left[\begin{array}{nghiempt}x=1\\x=2\\x=-1\\x=-5\end{array}\right.\)
HQ
Hà Quang Minh
Giáo viên
30 tháng 9 2023
a) \(\sqrt {3{x^2} - 6x + 1} = \sqrt { - 2{x^2} - 9x + 1} \)
Bình phương hai vế của phương trình \(\sqrt {3{x^2} - 6x + 1} = \sqrt { - 2{x^2} - 9x + 1} \) ta được
\(3{x^2} - 6x + 1 = - 2{x^2} - 9x + 1\)
\( \Leftrightarrow 5{x^2} + 3x = 0\)
\( \Leftrightarrow x\left( {5x + 3} \right) = 0\)
\( \Leftrightarrow x = 0\) hoặc \(x = \frac{{ - 3}}{5}\)
Thay lần lượt hai giá trị này của x vào phương trình đã cho, ta thấy cả hai giá trị x = 0 và \(x = \frac{{ - 3}}{5}\) đều thỏa mãn.
Vậy tập nghiệm của phương trình đã cho là \(S = \left\{ {0;\frac{{ - 3}}{5}} \right\}\)
b) \(\sqrt {2{x^2} - 3x - 5} = \sqrt {{x^2} - 7} \)
Bình phương hai vế của phương trình \(\sqrt {2{x^2} - 3x - 5} = \sqrt {{x^2} - 7} \) , ta được
\(2{x^2} - 3x - 5 = {x^2} - 7\)
\( \Leftrightarrow {x^2} - 3x + 2 = 0\)
\( \Leftrightarrow x = 1\) hoặc \(\)\(x = 2\)
Thay lần lượt giá trị của x vào phương trình đã cho, ta thấy không có giá trị nào của x thỏa mãn.
Vậy phương trình đã cho vô nghiệm.
PT
1
CM
29 tháng 12 2018
Điều kiện của phương trình x 2 + 3 x + 7 > 0
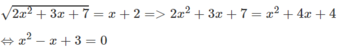
Phương trình cuối vô nghiệm, do đó phương trình đã cho vô nghiệm.
T
1
=>\(\dfrac{3x+7-x-1}{\sqrt{3x+7}+\sqrt{x+1}}=2\)
=>\(\sqrt{3x+7}+\sqrt{x+1}=x+3\)
=>3x+7+x+1+2 căn 3x^2+10x+7=x^2+6x+9
=>căn 12x^2+40x+28=x^2+2x
=>x^4+4x^3+4x^2-12x^2-40x-28=0
=>x^4+4x^3-8x^2-40x-28=0
=>\(x\in\left\{-0.94;3.21\right\}\)