Giải các hệ phương trình sau: 4...

Khách

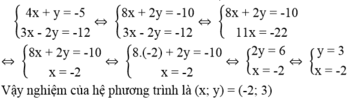
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Mua vip

Pham Trong Bach

21 tháng 6 2019

Giải các hệ phương trình sau: 4 x + y = - 5 3 x - 2 y = - 12

#Hỏi cộng đồng OLM #Toán lớp 9

Cao Minh Tâm

21 tháng 6 2019

Đúng(0)

Các câu hỏi dưới đây có thể giống với câu hỏi trên

░▒▓█►─═S͎óI͎❖T͎R͎ắN͎G͎❖G͎A͎M͎I͎N͎G͎...

28 tháng 1 2020 - olm

Giải các hệ phương trình sau:

a) \(\hept{\begin{cases}x^3+x^3y^3+y^3=17\\x+y+xy=5\end{cases}}\)

b) \(\hept{\begin{cases}x^4+x^2y^2+y^4=481\\x^2+xy+y^2=37\end{cases}}\)

#Hỏi cộng đồng OLM #Toán lớp 9

Nguyễn Văn Tuấn Anh

28 tháng 1 2020

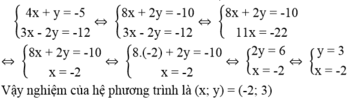
Câu dễ làm trước !

b) \(\hept{\begin{cases}x^4+x^2y^2+y^4=481\\x^2+xy+y^2=37\end{cases}}\)

\(\Leftrightarrow\hept{\begin{cases}\left(x^2+y^2\right)-x^2y^2=481\\x^2+xy+y^2=37\end{cases}}\)

\(\Leftrightarrow\hept{\begin{cases}\left(x^2-xy+y^2\right)=13\\x^2+xy+y^2=37\end{cases}}\)

\(\Leftrightarrow\hept{\begin{cases}xy=12\\x^2+y^2=25\end{cases}}\)

\(\Rightarrow\hept{\begin{cases}\left(x^2+2xy+y^2\right)-xy=37\\\left(x^2-2xy+y^2\right)+xy=13\end{cases}}\)

\(\Leftrightarrow\hept{\begin{cases}\left(x+y\right)^2=49\\\left(x-y\right)^2=1\end{cases}}\) (thay xy=12)

\(\Leftrightarrow\hept{\begin{cases}x=4\\y=3\end{cases}}\) hoặc \(\hept{\begin{cases}x=-4\\y=-3\end{cases}}\)

\(\Leftrightarrow\orbr{\begin{cases}\hept{\begin{cases}x+y=7\\x-y=1\end{cases}}\\\hept{\begin{cases}x+y=-7\\x-y=-1\end{cases}}\end{cases}}\)

Đúng(0)

░▒▓█►─═S͎óI͎❖T͎R͎ắN͎G͎❖G͎A͎M͎I͎N͎G͎...

29 tháng 1 2020

thanks you♥

Đúng(0)

Đặng Minh Triều

16 tháng 5 2016

Giải các hệ phương trình sau:

a)\(\begin{cases}x^3+y^3=1\\x^5+y^5=x^2+y^2\end{cases}\)

b)\(\begin{cases}3xy=4\left(x+y\right)\\5yz=6\left(y+z\right)\\7zx=8\left(z+x\right)\end{cases}\)

#Hỏi cộng đồng OLM #Toán lớp 9

Minh Triều

16 tháng 5 2016 - olm

Giải các hệ phương trình sau:

a)\(\int^{x^3+y^3=1}_{x^5+y^5=x^2+y^2}\)

b)\(\int^{3xy=4\left(x+y\right)}_{^{5yz=6\left(y+z\right)}_{7zx=8.\left(z+x\right)}}\)

#Hỏi cộng đồng OLM #Toán lớp 9

Thắng Nguyễn

16 tháng 5 2016

ê cu bài phần a nè

(2)<=>X²(1-X³)+y²(1-y³)=0 (3)

từ (1) => 1-x³=y³;1-y³=x³

thay vào (3)ta được :x².y³+y².x³=0

<=>x².y².(x+y)=0 (tới đây tự lo liệu)

Đúng(0)

ngô ngọc huyền

1 tháng 2 2018 - olm

GIẢI HỆ PHƯƠNG TRÌNH

\(\frac{2x+1}{4}-\frac{y-2}{3}=\frac{1}{12}\)

\(\frac{x+5}{2}=\frac{y+7}{3}-4\)

giúp mik giải nhé ( bên trên là hệ phương trình đó do mik ko bik ghi dấu ngoặc ở đầu nên để vậy)

#Hỏi cộng đồng OLM #Toán lớp 9

lan anh le

1 tháng 2 2018

\(\frac{2x+1}{4}\)-\(\frac{y-2}{3}\)=\(\frac{1}{12}\)

=\(\frac{3.\left[2x+1\right]}{12}\)-\(\frac{4.\left[y-2\right]}{12}\)=\(\frac{1}{12}\)

=6x+3-4y-6=1

=6x-3-4y=1

=6x-4y=4

=2[3x-2y]=4

MK MỚI HỌC LỚP 8 ,CHÚA SẼ CHUYỂN HỆ PHƯƠNG TRÌNH CUỐI CÙNG ,BẠN GIẢI NỐT NHA

Đúng(0)

Cố gắng hơn nữa

10 tháng 5 2018 - olm

giải hệ phương trình sau : \(\hept{\begin{cases}x^3-3x^2+2=y\sqrt{y+3}\\3\sqrt{x-2}+\sqrt{y-1}+x^2=12\end{cases}}\)

#Hỏi cộng đồng OLM #Toán lớp 9

Anh Dương Ngọc

2 tháng 11 2018 - olm

giải hệ phương trình \(\hept{\begin{cases}\left(x+y\right)^2\\\left(x-y\right)^2-2\left(x-y\right)=3\end{cases}-4\left(x+y\right)=12}\)12

#Hỏi cộng đồng OLM #Toán lớp 9

Anh Dương Ngọc

2 tháng 11 2018

trên là 12 thôi ạ

Đúng(0)

Phạm Gia Hiếu

29 tháng 7 2018 - olm

a) giải phương trình \(\sqrt{5-3x}\) + \(\sqrt{x+1}\)= \(\sqrt{3x^2-4x+4}\)

b)giải hệ phương trình :\(\hept{\begin{cases}6x+\frac{3}{x+y}=13\\12\left(x^2+xy+y^2\right)+\frac{9}{\left(x+y\right)^2}=85\end{cases}}\)

#Hỏi cộng đồng OLM #Toán lớp 9

nguyen tien phuc

25 tháng 3 2020 - olm

Giải hệ phương trình \(\hept{\begin{cases}5\left(x-y\right)-3\left(x+2y\right)=12\\3\left(x-y\right)-4\left(x+y2\right)=5\end{cases}}\)

#Hỏi cộng đồng OLM #Toán lớp 9

ɦσʂɦĭмĭүα☆ĭ¢ɦĭɠσ•

25 tháng 3 2020

Rút gọn từng phương trình ta có hệ :

\(\hept{\begin{cases}5\left(x-y\right)-3\left(x+2y\right)=12\\3\left(x-y\right)-4\left(x+2y\right)=5\end{cases}\Leftrightarrow\hept{\begin{cases}2x-11y=12\\-x-11y=5\end{cases}}}\)

Cộng từng vế của hai phương trình, ta được :

\(\Leftrightarrow\hept{\begin{cases}3x=7\\-x-11y=5\end{cases}\Leftrightarrow\hept{\begin{cases}x=\frac{7}{3}\\-\frac{7}{3}-11y=5\end{cases}\Leftrightarrow}\hept{\begin{cases}x=\frac{3}{7}\\y=-\frac{2}{3}\end{cases}}}\)

Suy ra \(\left(\frac{7}{3};-\frac{2}{3}\right)\)là nghiệm của hệ phương trình

Đúng(0)

New_New

1 tháng 11 2016 - olm

giải các hệ phương trình

\(\hept{\begin{cases}x+y+xy=5\\\left(x+1\right)^5+\left(y+1\right)^5=35\end{cases}}\)
\(\hept{\begin{cases}x+x^2+x^3+x^4=y+y^2+y^3+y^4\\x^2+y^2=1\end{cases}}\)

#Hỏi cộng đồng OLM #Toán lớp 9

alibaba nguyễn

1 tháng 11 2016

Ta có

x + x² + x³ + x⁴ = y + y² + y³ + y⁴

<=> (x - y) + (x² - y²) + (x³ - y²) + (x⁴ - y⁴) = 0

<=> (x - y)[1 + x + y + x² + xy + y² + (x² + y²)(x + y)]

<=> (x - y)(2 + 2x + 2y + xy)

\(\Leftrightarrow\orbr{\begin{cases}x-y=0\\2+2x+2y+xy=0\end{cases}}\)

Tới đây bạn tự giải tiếp nhé. Tính không giải đâu mà thấy bạn nhờ nên mới giải tiếp

Đúng(0)

alibaba nguyễn

1 tháng 11 2016

1/ \(\hept{\begin{cases}x+y+xy=5\\\left(x+1\right)^5+\left(y+1\right)^5=35\end{cases}}\)

\(\Leftrightarrow\hept{\begin{cases}\left(x+1\right)\left(y+1\right)=6\\\left(x+1\right)^5+\left(y+1\right)^5=35\end{cases}}\)

Đặt \(\hept{\begin{cases}x+1=a\\y+1=b\end{cases}}\)thì hệ thành

\(\hept{\begin{cases}ab=6\\a^5+B^5=35\end{cases}}\)

\(\Rightarrow a^5+\frac{6^5}{a^5}=35\)

PT này vô nghiệm vậy pt ban đầu vô nghiệm

Đúng(0)

nguyễn thùy linh

5 tháng 9 2019 - olm

bài 1: giải các phương trình sau :

a) x^3-5x=0 b) căn bậc 2 của x-1=3

bài 2 :

cho hệ phương trình : {2x+my;3x-y=0 (I)

a) giải hệ phương trình khi m=0

b) tìm giá trị của m để hệ (I) có nghiệm (x;y) thỏa mãn hệ thức :

x-y+m+1/m-2=-4

bài 3:giải các phương trình sau

a)5x-2/3=5x-3/2 b) 10x+3/12=1+6x+8/9 c) 2(x+3/5)=5-(13/5+x) d) 7/8x-5(x-9)=20x+1,5/6

#Hỏi cộng đồng OLM #Toán lớp 9

Chúng tôi đề xuất

Tài nguyên

Ứng dụng mobile

Bảng xếp hạng

Các khóa học có thể bạn quan tâm

Yêu cầu VIP