
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


a) =
=
.
b) =
=
.
c) =
=
.
d) y' =\(\dfrac{\left(x^2+7x+3\right)'\left(x^2-3x\right)-\left(x^2+7x+3\right)\left(x^2-3x\right)'}{\left(x^2-3x\right)^2}\)=\(\dfrac{\left(2x+7\right)\left(x^2-3x\right)-\left(x^2+7x+3\right)\left(2x-3\right)}{\left(x^2-3x\right)^2}\)=\(\dfrac{-2x^2-6x+9}{\left(x^2-3x\right)^2}\)

y ' = ( 4 x + 1 ) ' . x 2 + 2 − ( 4 x + 1 ) . ( x 2 + 2 ) ' x 2 + 2 = 4. x 2 + 2 − ( 4 x + 1 ) . x x 2 + 2 x 2 + 2 = 4 ( x 2 + 2 ) − ( 4 x + 1 ) . x ( x 2 + 2 ) . x 2 + 2 = − x + 8 ( x 2 + 2 ) . x 2 + 2
ĐÁP ÁN D


tham khảo:
a)y′=2\(^{3x-x^2}\).ln2.(3−2x)
b) y′\(\dfrac{4}{ln3}\).\(\dfrac{1}{4x+1}\).4=\(\dfrac{4}{\left(4x+1\right)ln3}\)

a: \(y'=\left(x^2\right)'+\left(3x\right)'-\left(6x^6\right)'+\left(\dfrac{2x-3}{x-1}\right)'\)
\(=2x+3-6\cdot6x^5+\dfrac{\left(2x-3\right)'\left(x-1\right)-\left(2x-3\right)\left(x-1\right)'}{\left(x-1\right)^2}\)
\(=-36x^5+2x+3+\dfrac{2\left(x-1\right)-2x+3}{\left(x-1\right)^2}\)
\(=-36x^5+2x+3+\dfrac{1}{\left(x-1\right)^2}\)
b: \(\left(\sqrt{2x^2-3x+1}\right)'=\dfrac{\left(2x^2-3x+1\right)'}{2\sqrt{2x^2-3x+1}}\)
\(=\dfrac{4x-3}{2\sqrt{2x^2-3x+1}}\)
\(y'=3\cdot2x-4+\dfrac{4x-3}{2\sqrt{2x^2-3x+1}}\)
\(=6x-4+\dfrac{4x-3}{2\sqrt{2x^2-3x+1}}\)
c: \(\left(\sqrt{4x^2-3x+1}\right)'=\dfrac{\left(4x^2-3x+1\right)'}{2\sqrt{4x^2-3x+1}}\)
\(=\dfrac{8x-3}{2\sqrt{4x^2-3x+1}}\)
\(y'=\left(\sqrt{4x^2-3x+1}\right)'-4'=\dfrac{8x-3}{2\sqrt{4x^2-3x+1}}\)

\(\sqrt[n]{y}=4x+1\)
\(y^{\dfrac{1}{n}}=4x+1\)
đạo cấp 1
\(\dfrac{1}{n}y^{\left(\dfrac{1}{n}-1\right)}=\dfrac{1}{n}\sqrt[n]{y^{\left(1-n\right)}}=4\)
thay y=(4x+1)^n vào
\(\dfrac{1}{n}\sqrt[n]{\left(4x+1\right)^{n\left(1-n\right)}}=\dfrac{1}{n}\left(4x+1\right)^{\left(1-n\right)}\)
từ đó: \(y'=\dfrac{4}{\dfrac{1}{n}\left(4x+1\right)^{\left(1-n\right)}}=4.n\left(4x+1\right)^{n-1}\)
Có đúng không: cấp n có thể phải làm lấy vài cái--> quy luật nào đó
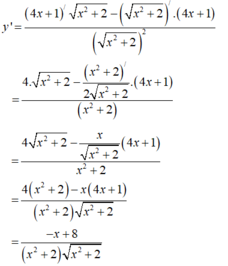
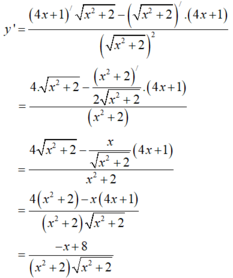
Đây là lấy vị chia