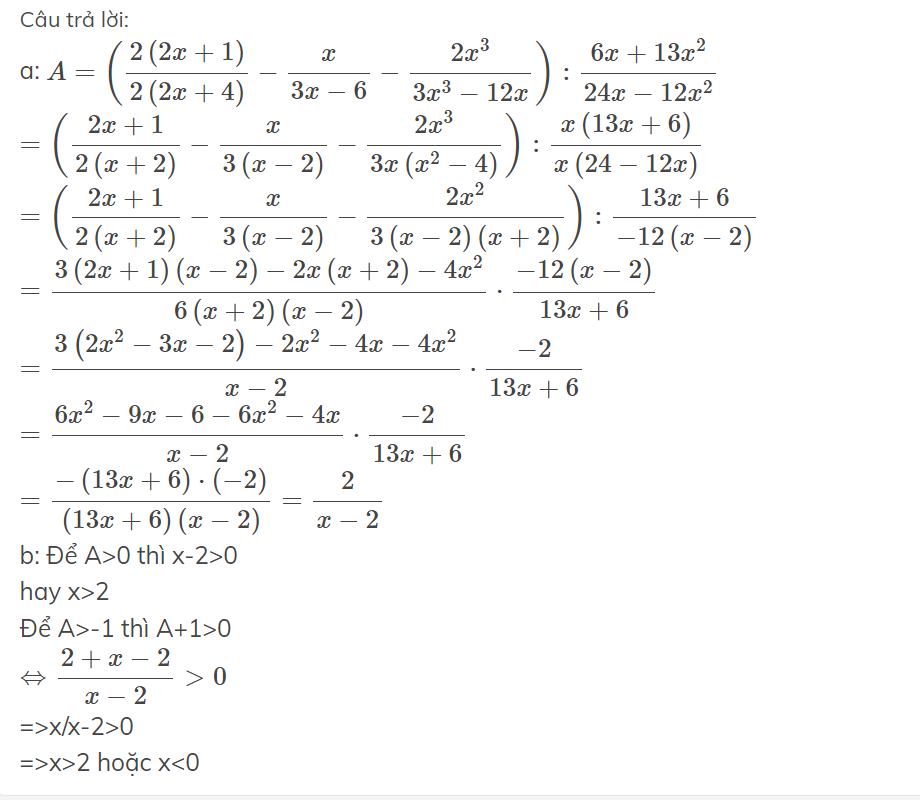Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

A) \(A^2+B^2\ge2AB\Leftrightarrow\left(A-B\right)^2\ge0\)(luôn đúng)
B)\(A^2B=A\cdot A\cdot B;AB^2=A\cdot B\cdot B\)
áp dụng BĐT AM-GM
\(A\cdot A\cdot B\le\dfrac{A^3+A^3+B^3}{3};A\cdot B\cdot B\le\dfrac{A^3+B^3+B^3}{3}\)
cộng 2 vế của BĐT cho nhau
\(\Rightarrow A^2B+AB^2\le A^3+B^3\left(đpcm\right)\)
C)tương tự câu B) ta có
\(A^3B\le\dfrac{A^4+A^4+A^4+B}{4};AB^3\le\dfrac{A^4+B^4+B^4+B^{\text{4}}}{4}\)
cộng từng vế của BĐT ta có đpcm

Ta có : 4x + y = 1 => y = 1 - 4x
=> 4x^2 + y^2 = 4x^2 + ( 1 - 4x )^2 = 20x^2 - 8x + 1 = 4 ( 5x^2 - 2x ) + 1 = 4/5 ( 25x^2 - 10x + 1 ) + 1/5 = 4/5 ( 5x-1 )^2 +1/5
Ta có : ( 5x-1)^2 >= 0
=> 4/5 ( 5x-1)^2 +1/5 >= 0 + 1/5 = 1/5
Vậy 4x^2 + y^2 >= 1/5. Dấu "=" xảy ra <=> x= 1/5
Áp dụng BĐT Bunhiacopxki ta có:
\(\left[\left(2x\right)^2+y^2\right].\left(2^2+1\right)\ge\left(4x+y\right)^2=1\)
\(\Leftrightarrow4x^2+y^2\ge\frac{1}{5}\)
Dấu " = " xảy ra <=> \(\frac{2x}{2}=y\Leftrightarrow x=y=0,2\)

\(M=\dfrac{yz\sqrt{x-1}+xz\sqrt{y-2}+xy\sqrt{z-3}}{xyz}\)
\(=\dfrac{yz\sqrt{x-1}}{xyz}+\dfrac{xz\sqrt{y-2}}{xyz}+\dfrac{xy\sqrt{z-3}}{xyz}\)
\(=\dfrac{\sqrt{x-1}}{x}+\dfrac{\sqrt{y-2}}{y}+\dfrac{\sqrt{z-3}}{z}\)
Áp dụng BĐT AM-GM ta có:
\(\sqrt{x-1}\le\dfrac{1+x-1}{2}=\dfrac{x}{2}\)\(\Rightarrow\dfrac{\sqrt{x-1}}{x}\le\dfrac{x}{2}\cdot\dfrac{1}{x}=\dfrac{1}{2}\)
\(\sqrt{y-2}=\dfrac{\sqrt{2\left(y-2\right)}}{\sqrt{2}}\le\dfrac{y}{2\sqrt{2}}\)\(\Rightarrow\dfrac{\sqrt{y-2}}{y}\le\dfrac{y}{2\sqrt{2}}\cdot\dfrac{1}{y}=\dfrac{1}{2\sqrt{2}}\)
\(\sqrt{z-3}=\dfrac{\sqrt{3\left(z-3\right)}}{\sqrt{3}}\le\dfrac{z}{2\sqrt{3}}\)\(\Rightarrow\dfrac{\sqrt{z-3}}{z}\le\dfrac{z}{2\sqrt{3}}\cdot\dfrac{1}{z}=\dfrac{1}{2\sqrt{3}}\)
Cộng theo vế 3 BĐT trên ta có:
\(M\le\dfrac{1}{2}\left(1+\dfrac{1}{\sqrt{2}}+\dfrac{1}{\sqrt{3}}\right)\) (ĐPCM)

a)\(\frac{3xy+6}{6xy+12}=\frac{1}{2}\Leftrightarrow\left(3xy+6\right)\cdot2=\left(6xy+12\right)\cdot1\)
\(\Leftrightarrow6xy+12=6xy+12\)
Vậy.......
b)\(\frac{x^2-xy}{5y^2-5xy}=\frac{x}{5y}\Leftrightarrow\left(x^2-xy\right)\cdot5y=\left(5y^2-5xy\right)\cdot x\)
\(\Leftrightarrow5x^2y-5xy^2=5xy^2-5x^2y\)
Vậy.....

\(4x^2+3xy-11y^2=5x^2-x^2-2xy+5xy-10y^2-y^2\)
\(=5\left(x^2+xy+2y^2\right)-\left(x^2+2xy+y^2\right)=5\left(x^2+xy+2y^2\right)-\left(x+y\right)^2\)
Ta có \(4x^2+3xy-11y^2\) chia hết cho 5
=> \(\left(x+y\right)^2\) chia hết cho 5
Mà 5 là số nguyên tố
=> x+y chia hết cho 5
Mặt khác
\(x^4-y^4=\left(x^2-y^2\right)\left(x^2+y^2\right)=\left(x+y\right)\left(x-y\right)\left(x^2+y^2\right)\)
=> \(x^4-y^4\) chia hết cho 5 (đpcm)