Cho ba số thực a,b,c dương chứng minh rằng:\(\sqrt{\dfrac{a^3}{a^3+\left(b+c\right)^3}}+\...

\(\sqrt{\dfrac{a^3}{a^3+\left(b+c\right)^3}}+\...">

K

Khách

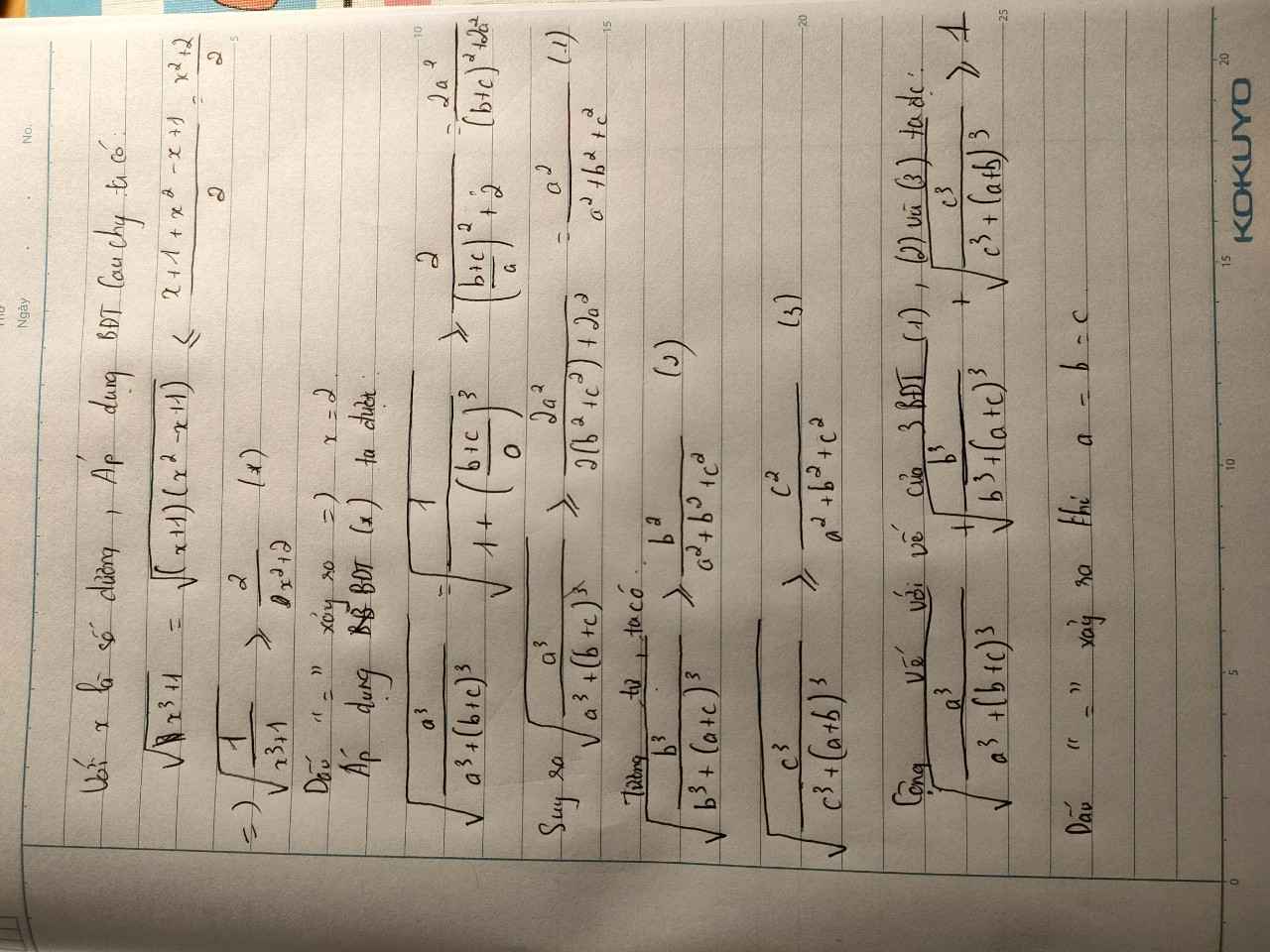
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Mua vip

HA

Hoàng Anh Thắng

13 tháng 3 2022

Cho ba số thực a,b,c dương chứng minh rằng:

\(\sqrt{\dfrac{a^3}{a^3+\left(b+c\right)^3}}+\sqrt{\dfrac{b^3}{b^3+\left(c+a\right)^3}}+\sqrt{\dfrac{c^3}{c^3+\left(a+b\right)^3}}\ge1\)

#Hỏi cộng đồng OLM #Toán lớp 9

2

C

Corona

13 tháng 3 2022

DT

Đào Tùng Dương

13 tháng 3 2022

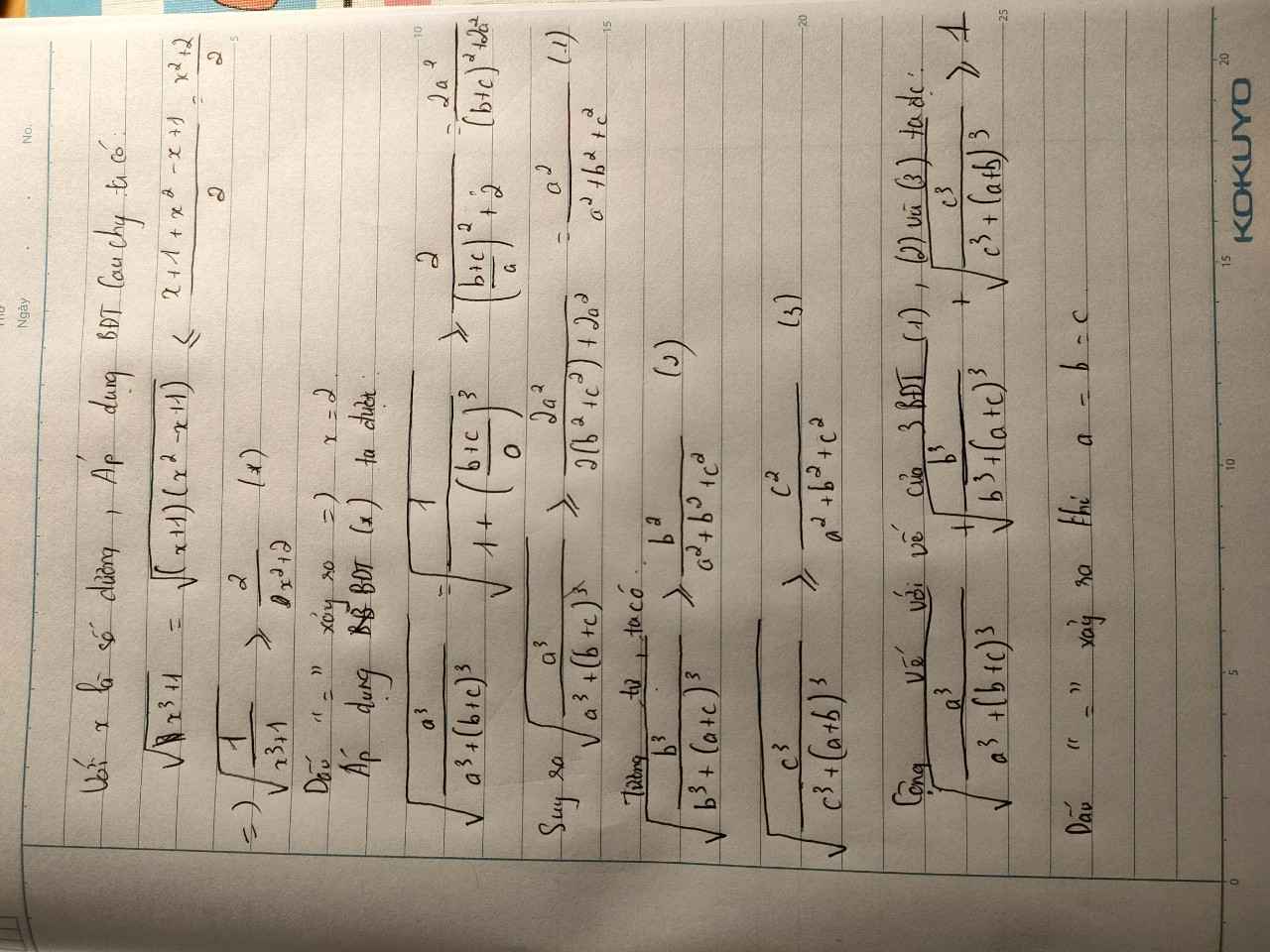
undefined

Các câu hỏi dưới đây có thể giống với câu hỏi trên

PA

Pham Anh Tuan

5 tháng 9 2018

Cho a,b,c là các số thực dương . Chứng minh rằng : \(\sqrt{\dfrac{a^3}{a^3+\left(b+c\right)^3}}\)+ \(\sqrt{\dfrac{b^3}{b^3+\left(c+a\right)^3}}\)+\(\sqrt{\dfrac{c^3}{c^3+\left(a+b\right)^3}}\)≥ 1

#Hỏi cộng đồng OLM #Toán lớp 9

0

VD

Võ Đông Anh Tuấn

24 tháng 11 2017

Cho 3 số thực a,b,c dương . CMR :

\(\sqrt{\dfrac{a^3}{a^3+\left(b+c\right)^3}}+\sqrt{\dfrac{b^3}{b^3+\left(c+a\right)^3}}+\sqrt{\dfrac{c^3}{c^3+\left(a+b\right)^3}}\)

#Hỏi cộng đồng OLM #Toán lớp 9

2

VD

Võ Đông Anh Tuấn

24 tháng 11 2017

Lớn hơn hoặc = 1

BN

Bích Ngọc Huỳnh

25 tháng 11 2017

mạng có đó tương tự như z mà làm theo !!

TH

Trần Hoàng Đạt

5 tháng 1 2019

\(\sqrt{\dfrac{a^3}{a^3+\left(b+c\right)^3}}+\sqrt{\dfrac{b^3}{b^3+\left(a+c\right)^3}}+\sqrt{\dfrac{c^3}{c^3+\left(a+b\right)^3}}\ge1\)

#Hỏi cộng đồng OLM #Toán lớp 9

0

2

๖²⁴ʱ乂ų✌й๏✌ρɾ๏༉

25 tháng 6 2023

cho ba số thực a,b,c dương . chứng minh rằng :

\(\sqrt{\dfrac{a^3}{a^3+\left(b+c\right)^3}}\)+ \(\sqrt{\dfrac{b^3}{b^3+\left(c+a\right)^3}}\)+\(\sqrt{\dfrac{c^3}{c^3+\left(b+a\right)^3}}\)≥1

#Hỏi cộng đồng OLM #Toán lớp 9

1

MP

Minh Phương

25 tháng 6 2023

bn tham khảo nha

https://hoc24.vn/cau-hoi/cho-ba-so-thuc-abc-duong-chung-minh-rangsqrtdfraca3a3leftbcright3sqrtdfracb3b3leftcaright3sqrtdfracc3c.5222680437292

PP

Phạm Phương Anh

5 tháng 9 2018

Cho 3 số thực dương a, b, c. Tìm giá trị nhỏ nhất của biểu thức:

\(A=\sqrt{\dfrac{a^3}{a^3+\left(b+c\right)^3}}+\sqrt{\dfrac{b^3}{b^3+\left(c+a\right)^3}}+\sqrt{\dfrac{c^3}{c^3+\left(a+b\right)^3}}\)

#Hỏi cộng đồng OLM #Toán lớp 9

0

DT

Đinh Thuận

19 tháng 1 2019

Cho ba số dương a,b,c.Chứng minh rằng:\(\dfrac{a^3}{b\left(2c+a\right)}+\dfrac{b^3}{c\left(2a+b\right)}+\dfrac{c^3}{a\left(2b+c\right)}\ge1\)

#Hỏi cộng đồng OLM #Toán lớp 9

1

NV

Nguyễn Việt Lâm

Giáo viên

20 tháng 1 2019

Thử với \(a=b=c=0.1\), BĐT trở thành \(\dfrac{1}{10}\ge1\Rightarrow\) đề sai

N

noname

3 tháng 12 2017

cho a,b,c là các số dương thỏa mãn: a+b+c=5 và \(\sqrt{a}+\sqrt{b}+\sqrt{c}=3\)

chứng minh rằng: \(\dfrac{\sqrt{a}}{a+2}+\dfrac{\sqrt{b}}{b+2}+\dfrac{\sqrt{c}}{c+2}=\dfrac{4}{\sqrt{\left(a+2\right)\left(b+2\right)\left(c+2\right)}}\)

#Hỏi cộng đồng OLM #Toán lớp 9

1

CC

Chí Cường

4 tháng 12 2017

Ta có:

\(\left(\sqrt{a}+\sqrt{b}+\sqrt{c}\right)^2=9\\ \Leftrightarrow a+b+c+2\sqrt{ab}+2\sqrt{bc}+2\sqrt{ac}=9\\ \Leftrightarrow\sqrt{ab}+\sqrt{bc}+\sqrt{ac}=2\)

\(\Rightarrow\dfrac{\sqrt{a}}{a+2}+\dfrac{\sqrt{b}}{b+2}+\dfrac{\sqrt{c}}{c+2}=\dfrac{\sqrt{a}}{a+\sqrt{ab}+\sqrt{bc}+\sqrt{ac}}+\dfrac{\sqrt{b}}{b+\sqrt{ab}+\sqrt{bc}+\sqrt{ac}}+\dfrac{\sqrt{c}}{c+\sqrt{ab}+\sqrt{bc}+\sqrt{ac}}\\ =\dfrac{\sqrt{a}}{\left(\sqrt{a}+\sqrt{b}\right)\left(\sqrt{a}+\sqrt{c}\right)}+\dfrac{\sqrt{b}}{\left(\sqrt{a}+\sqrt{b}\right)\left(\sqrt{b}+\sqrt{c}\right)}+\dfrac{\sqrt{c}}{\left(\sqrt{b}+\sqrt{c}\right)\left(\sqrt{a}+\sqrt{c}\right)}\\ =\dfrac{\sqrt{a}\left(\sqrt{b}+\sqrt{c}\right)+\sqrt{b}\left(\sqrt{a}+\sqrt{c}\right)+\sqrt{c}\left(\sqrt{a}+\sqrt{b}\right)}{\left(\sqrt{a}+\sqrt{b}\right)\left(\sqrt{b}+\sqrt{c}\right)\left(\sqrt{a}+\sqrt{c}\right)}\\ =\dfrac{2\left(\sqrt{ab}+\sqrt{bc}+\sqrt{ac}\right)}{\left(\sqrt{a}+\sqrt{b}\right)\left(\sqrt{b}+\sqrt{c}\right)\left(\sqrt{a}+\sqrt{c}\right)}\\ =\dfrac{4}{\sqrt{\left(\sqrt{a}+\sqrt{b}\right)^2\left(\sqrt{b}+\sqrt{c}\right)^2\left(\sqrt{a}+\sqrt{c}\right)^2}}\)\(=\dfrac{4}{\sqrt{\left(a+\sqrt{ab}+\sqrt{bc}+\sqrt{ac}\right)\left(b+\sqrt{ab}+\sqrt{bc}+\sqrt{ac}\right)\left(c+\sqrt{ab}+\sqrt{bc}+\sqrt{ac}\right)}}\\ =\dfrac{4}{\sqrt{\left(a+2\right)\left(b+2\right)\left(c+2\right)}}\)

AA

Admin (a@olm.vn)

Admin VIP

22 tháng 3 2021 - olm

Cho \(a,b,c\) là ba số dương thỏa mãn \(abc\ge1\). Chứng minh rằng

\(\dfrac{a^3}{\left(1+b\right)\left(1+c\right)}+\dfrac{b^3}{\left(1+c\right)\left(1+a\right)}+\dfrac{c^3}{\left(1+a\right)\left(1+b\right)}\ge\dfrac{3}{4}\).

#Hỏi cộng đồng OLM #Toán lớp 9

3

PT

Phạm Thành Đông

22 tháng 3 2021

Đặt \(A=\frac{a^3}{\left(1+b\right)\left(1+c\right)}+\frac{b^3}{\left(1+c\right)\left(1+a\right)}+\frac{c^3}{\left(1+a\right)\left(1+b\right)}\)

Vì \(a,b,c>0\)nên áp dụng bất đẳng thức Cô-si cho 3 số dương, ta được:

\(\frac{a^3}{\left(1+b\right)\left(1+c\right)}+\frac{1+b}{8}+\frac{1+c}{8}\ge3\sqrt[3]{\frac{a^3\left(1+b\right)\left(1+c\right)}{\left(1+b\right)\left(1+c\right).64}}\)\(=3\sqrt[3]{\frac{a^3}{64}}=\frac{3a}{4}\left(1\right)\)

Chứng minh tương tự, ta được:

\(\frac{b^3}{\left(1+c\right)\left(1+a\right)}+\frac{1+c}{8}+\frac{1+a}{8}\ge\frac{3b}{4}\left(2\right)\)

\(\frac{c^3}{\left(1+a\right)\left(1+b\right)}+\frac{1+a}{8}+\frac{1+b}{8}\ge\frac{3a}{4}\left(3\right)\)

Từ (1), (2), (3), ta được:

\(\frac{a^3}{\left(1+b\right)\left(1+c\right)}+\frac{b^3}{\left(1+c\right)\left(1+a\right)}+\frac{c^3}{\left(1+a\right)\left(1+b\right)}\)\(+\frac{1+a}{8}+\frac{1+b}{8}+\frac{1+c}{8}+\frac{1+a}{8}+\frac{1+b}{8}+\frac{1+c}{8}\)\(\ge\frac{3a}{4}+\frac{3b}{4}+\frac{3c}{4}\)

\(\Leftrightarrow A+\frac{1+a}{4}+\frac{1+b}{4}+\frac{1+c}{4}\ge\frac{3a}{4}+\frac{3b}{4}+\frac{3c}{4}\)

\(\Leftrightarrow A+\frac{1+a+1+b+1+c}{4}\ge\frac{3a+3b+3c}{4}\)

\(\Leftrightarrow A+\frac{3+a+b+c}{4}\ge\frac{3\left(a+b+c\right)}{4}\)

\(\Leftrightarrow A\ge\frac{3\left(a+b+c\right)}{4}-\frac{3-a-b-c}{4}\)

\(\Leftrightarrow A\ge\frac{3\left(a+b+c\right)-\left(a+b+c\right)}{4}-\frac{3}{4}\)

\(\Leftrightarrow A\ge\frac{2\left(a+b+c\right)}{4}-\frac{3}{4}\left(4\right)\)

Mặt khác, vì \(a,b,c>0\)nên áp dụng bất đẳng thức Cô-si cho 3 số dương, ta được:

\(a+b+c\ge3\sqrt[3]{abc}\)

Mà \(abc\ge1\Leftrightarrow\sqrt[3]{abc}\ge1\Leftrightarrow3\sqrt[3]{abc}\ge3\)

Do đó:

\(a+b+c\ge3\)

\(\Leftrightarrow2\left(a+b+c\right)\ge6\)

\(\Leftrightarrow\frac{2\left(a+b+c\right)}{4}\ge\frac{6}{4}=\frac{3}{2}\)

\(\Leftrightarrow\frac{2\left(a+b+c\right)}{4}-\frac{3}{4}\ge\frac{3}{2}-\frac{3}{4}=\frac{3}{4}\left(5\right)\)

Từ (4) và (5), ta được:

\(A\ge\frac{3}{4}\)(điều phải chứng minh)

Dấu bằng xảy ra.

\(\Leftrightarrow\hept{\begin{cases}a=b=c>0\\abc=1\end{cases}}\Leftrightarrow a=b=c=1\)

Vậy \(\frac{a^3}{\left(1+b\right)\left(1+c\right)}+\frac{b^3}{\left(1+c\right)\left(1+a\right)}+\frac{c^3}{\left(1+a\right)\left(1+b\right)}\ge\frac{3}{4}\)với \(a,b,c>0\)và \(abc\ge1\)

PB

Phạm Bá Huy

13 tháng 7 2021

Với $x, y > 0$ đã cho, áp dụng bất đẳng thức Cô si ta có

$\dfrac{a^3}{\left(1+b\right)\left(1+c\right)}+\dfrac{1+b}{x}+\dfrac{1+c}{y}\ge\dfrac{3a}{\sqrt[3]{xy}}$

Kỳ vọng rằng bất đẳng thức cần chứng minh trở thành đẳng thức khi $a = b = c = 1$ , ta chọn $x > 0$ sao cho $\dfrac{a^3}{\left(1+b\right)\left(1+c\right)}=\dfrac{1+b}{x}=\dfrac{1+c}{y}$ xảy ra khi $a = b = c = 1$ , tức là $\dfrac{1}{4}=\dfrac{2}{x}=\dfrac{2}{y}\Leftrightarrow x=y=8$ . Vì vậy

$\dfrac{a^3}{\left(1+b\right)\left(1+c\right)}+\dfrac{1+b}{8}+\dfrac{1+c}{8}\ge\dfrac{3a}{4}$

Viết hai bất đẳng thức tương tự rồi cộng theo vế ba bất đẳng thức này ta có

$\dfrac{a^3}{\left(1+b\right)\left(1+c\right)}+\dfrac{b^3}{\left(1+c\right)\left(1+a\right)}+\dfrac{c^3}{\left(1+a\right)\left(1+b\right)}+\dfrac{3}{4}+\dfrac{a+b+c}{4}\ge$

$\dfrac{3}{4}\left(a+b+c\right)$

Hay $\dfrac{a^3}{\left(1+b\right)\left(1+c\right)}+\dfrac{b^3}{\left(1+c\right)\left(1+a\right)}+\dfrac{c^3}{\left(1+a\right)\left(1+b\right)}\ge\dfrac{1}{2}\left(a+b+c\right)-\dfrac{3}{4}$

Mà $a+b+c\ge3\sqrt[3]{abc}\ge3$ . Suy ra

$\dfrac{a^3}{\left(1+b\right)\left(1+c\right)}+\dfrac{b^3}{\left(1+c\right)\left(1+a\right)}+\dfrac{c^3}{\left(1+a\right)\left(1+b\right)}\ge\dfrac{3}{4}$

2

๖²⁴ʱ乂ų✌й๏✌ρɾ๏༉

25 tháng 6 2023

Cho a,b,c là các số dương . Chứng minh rằng :

\(\sqrt{\dfrac{a^3}{a^3+\left(b+c\right)^3}}\)+ \(\sqrt{\dfrac{b^3}{b^3+\left(c+a\right)^3}}\) + \(\sqrt{\dfrac{c^3}{c^3+\left(a+b\right)^3}}\)

#Hỏi cộng đồng OLM #Toán lớp 9

1

GH

Gia Huy

25 tháng 6 2023

Xem lại câu hỏi

2

๖²⁴ʱ乂ų✌й๏✌ρɾ๏༉

25 tháng 6 2023

đúng r ạ !!