Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

S A B C D H E K F
Ta có
\(SH\perp\left(ABCD\right);SH\in\left(SBD\right)\Rightarrow\left(SBD\right)\perp\left(ABCD\right)\)
Trong mp (ABCD) từ C dựng đường thẳng vuông góc với BD cắt BD tại F ta có
\(SH\perp\left(ABCD\right);CF\in ABCD\Rightarrow SH\perp CF\)
Mà \(CF\perp BD\)
Ta có \(BD\in\left(SBD\right);SH\in\left(SBD\right)\)
\(\Rightarrow CF\perp\left(SBD\right)\) => CF là khoảng cách từ C đến (SBD)
Trong mp (ABCD) nối CH cắt AD tại E
Ta có BC//AD \(\Rightarrow\dfrac{BC}{ED}=\dfrac{HB}{HD}=\dfrac{HC}{HE}=1\Rightarrow ED=BC=\dfrac{3a}{2}\)
\(\Rightarrow EA=AD-ED=3a-\dfrac{3a}{2}=\dfrac{3a}{2}=BC\)
Mà BC//AE và \(\widehat{ABC}=90^o\)
=> ABCE là hình chữ nhật
Trong mp (ABCD) từ H dựng đường thẳng vuông góc với CD cắt CD tại K
Xét tg vuông CDE có
\(CD=\sqrt{CE^2+ED^2}=\sqrt{4a^2+\dfrac{9a^2}{4}}=\dfrac{5a}{2}\)
Xét tg vuông ABD có
\(BD=\sqrt{AB^2+AD^2}=\sqrt{4a^2+9a^2}=a\sqrt{13}\)
\(\Rightarrow HB=HD=\dfrac{BD}{2}=\dfrac{a\sqrt{13}}{2}\)
Xét tg vuông CKH và tg vuông CED có \(\widehat{ECD}\) chung
=> tg CKH đồng dạng với tg CED (g.g.g)
\(\Rightarrow\dfrac{CK}{CE}=\dfrac{HC}{CD}\Rightarrow CK=\dfrac{CE.HC}{CD}=\dfrac{2a.a}{\dfrac{5a}{2}}=\dfrac{4a}{5}\)
Xét tg vuông CKH có
\(HK=\sqrt{HC^2-CK^2}=\sqrt{a^2-\dfrac{16a^2}{25}}=\dfrac{3a}{5}\)
Xét tg vuông DKH và tg vuông DFC có \(\widehat{BDC}\) chung
=> tg DKH đồng dạng với tg DFC (g.g.g)
\(\Rightarrow\dfrac{HK}{CF}=\dfrac{HD}{CD}\Rightarrow CF=\dfrac{HK.CD}{HD}=\dfrac{\dfrac{3a}{5}.\dfrac{5a}{2}}{\dfrac{a\sqrt{13}}{2}}=\dfrac{3a\sqrt{13}}{13}\)


Ta có \(\frac{d\left(A,\left(SCD\right)\right)}{d\left(M,\left(SCD\right)\right)}=2\Rightarrow d=\left(m,\left(SCD\right)\right)=\frac{1}{2}d\left(A,\left(SCD\right)\right)\)
Dễ thấy AC _|_ CD, SA _|_ CD dựng AH _|_ SA => AH _|_ (SCD)
Vậy d(A,(SCD))=AH
Xét tam giác vuông SAC (A=1v) có \(\frac{1}{AH^2}=\frac{1}{AC^2}+\frac{1}{AS^2}\Rightarrow AH=\frac{a\sqrt{6}}{3}\)
Vậy suy ra \(d\left(M,\left(SCD\right)\right)=\frac{a\sqrt{6}}{3}\)
.jpg)
E=AB∩CD,G=EN∩SB⇒GE=AB∩CD,G=EN∩SB⇒G là trọng tâm tam giác SAE.
d(M,(NCD))=GMGBd(B,(NCD))=12d(B,(NCD))=12.12d(A,(NCD))=14d(A,(NCD))=14hd(M,(NCD))=GMGBd(B,(NCD))=12d(B,(NCD))=12.12d(A,(NCD))=14d(A,(NCD))=14h
Tứ diện AEND vuông tại đỉnh A nên 1h2=1AN2+1AE2+1AD2=116a2⇒h=a√66111h2=1AN2+1AE2+1AD2=116a2⇒h=a6611
Vậy d(M,(NCD))=a√6644.d(M,(NCD))=a6644.

S A B C D H O K I L T
a) SA vuông góc với (ABCD) => SA vuông góc AD; hình thang ABCD vuông tại A => AD vuông góc AB
=> AD vuông góc (SAB), mà AD nằm trong (SAD) nên (SAB) vuông góc (SAD).
b) AD vuông góc (SAB), BC || AD => BC vuông góc (SAB) => B là hc vuông góc của C trên (SAB)
=> (SC,SAB) = ^CAB
\(SB=\sqrt{AS^2+AB^2}=\sqrt{2a^2+a^2}\)\(=a\sqrt{3}\)
\(\tan\widehat{CAB}=\frac{BC}{SB}=\frac{a}{a\sqrt{3}}=\frac{\sqrt{3}}{3}\)=> (SC,SAB) = ^CAB = 300.
c) T là trung điểm của AD, K thuộc ST sao cho AK vuông góc ST, BT cắt AC tại O, HK cắt AO tại I, AI cắt SC tại L.
BC vuông góc (SAB) => BC vuông góc AH, vì AH vuông góc SB nên AH vuông góc SC. Tương tự AK vuông góc SC
=> SC vuông góc (HAK) => SC vuông góc AI,AL. Lập luận tương tự thì AL,AI vuông góc (SCD).
Dễ thấy \(\Delta\)SAB = \(\Delta\)SAT, chúng có đường cao tương ứng AH và AK => \(\frac{HS}{HB}=\frac{KS}{KT}\)=> HK || BT || CD
=> d(H,SCD) = d(I,SCD) = IL (vì A,I,L vuông góc (SCD)) = \(\frac{IL}{AL}.AL=\frac{CO}{CA}.\frac{SI}{SO}.AL=\frac{1}{2}.\frac{SH}{SB}.\frac{AS.AC}{\sqrt{AS^2+AC^2}}\)
\(=\frac{1}{2}.\frac{SA^2}{SA^2+SB^2}.\frac{AS.AC}{\sqrt{AS^2+AC^2}}=\frac{1}{2}.\frac{2a^2}{2a^2+a^2}.\frac{a\sqrt{2}.a\sqrt{2}}{\sqrt{2a^2+2a^2}}=\frac{a}{3}\)

Cho mình hỏi, cái chỗ tính HI không dùng cách này được hả bạn \(\dfrac{SH.HC}{\sqrt{SH^2+HC^2}}\)
Nếu không dùng được, bạn lí giải giùm mình với

Đáp án C
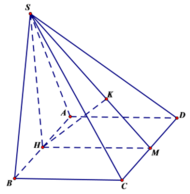
Gọi M là trung điểm của CD. Kẻ HK vuông góc với SM.
Ta có:

Mặt khác ta có HK ⊥ SM
Suy ra HK ⊥ (SCD)
Vậy ![]()
Xét tam giác BHC vuông tại B, ta có:
![]()
![]()
Xét tam giác SHM vuông tại H, ta có:
![]()


Tuy nhiên đề cho giá trị cạnh AC với BC bị sai. Cạnh huyền AC (\(a\sqrt{3}\)) sao lại có giá trị nhỏ hơn cạnh góc vuông BC (2a) nhỉ?



2. Câu 29 (Toán lớp 11)
2.1. Lập hệ trục, xác định tọa độ
\(A B = 2 a , B C = 2 a , A D = 3 a , (\text{v} \overset{ˋ}{\text{a}} \&\text{nbsp}; B C \parallel A D ).\)
\(A = \left(\right. 0 , \textrm{ } 0 , \textrm{ } 0 \left.\right) , B = \left(\right. 0 , \textrm{ } 2 a , \textrm{ } 0 \left.\right) .\)
\(D = \left(\right. 3 a , \textrm{ } 0 , \textrm{ } 0 \left.\right) , C = \left(\right. 2 a , \textrm{ } 2 a , \textrm{ } 0 \left.\right) .\)
\(A = \left(\right. 0 , 0 , 0 \left.\right) , C = \left(\right. 2 a , 2 a , 0 \left.\right) \textrm{ }\textrm{ } \rightarrow \textrm{ }\textrm{ } H = \left(\right. \frac{0 + 2 a}{2} , \textrm{ } \frac{0 + 2 a}{2} , \textrm{ } 0 \left.\right) = \left(\right. \textrm{ } a , \textrm{ } a , \textrm{ } 0 \textrm{ } \left.\right) .\)
Tính nhanh:
⇒ \(S\) phải có \(x = a , \textrm{ }\textrm{ } y = a\), và một giá trị \(z = h > 0.\)
\(\overset{\rightarrow}{B C} \textrm{ }\textrm{ } = \textrm{ }\textrm{ } C - B = \left(\right. 2 a , \textrm{ } 2 a , \textrm{ } 0 \left.\right) - \left(\right. 0 , \textrm{ } 2 a , \textrm{ } 0 \left.\right) = \left(\right. 2 a , \textrm{ } 0 , \textrm{ } 0 \left.\right) ,\) \(\overset{\rightarrow}{B S} \textrm{ }\textrm{ } = \textrm{ }\textrm{ } S - B = \left(\right. \textrm{ } a , \textrm{ } a , \textrm{ } h \left.\right) - \left(\right. 0 , \textrm{ } 2 a , \textrm{ } 0 \left.\right) = \left(\right. \textrm{ } a , \textrm{ } - a , \textrm{ } h \textrm{ } \left.\right) .\)
\(\mathbf{n}_{1} \textrm{ }\textrm{ } = \textrm{ }\textrm{ } \overset{\rightarrow}{B C} \times \overset{\rightarrow}{B S} = \left(\right. \textrm{ } 2 a , \textrm{ } 0 , \textrm{ } 0 \textrm{ } \left.\right) \times \left(\right. \textrm{ } a , \textrm{ } - a , \textrm{ } h \textrm{ } \left.\right) .\)
Tính trực tiếp:
\(\mathbf{n}_{1} = \mid \mathbf{i} & \mathbf{j} & \mathbf{k} \\ 2 a & 0 & 0 \\ a & - a & h \mid = \left(\right. 0 \cdot h \textrm{ }\textrm{ } - \textrm{ }\textrm{ } 0 \cdot \left(\right. - a \left.\right) \left.\right) \mathbf{i} \textrm{ }\textrm{ } - \textrm{ }\textrm{ } \left(\right. \left(\right. 2 a \left.\right) \cdot h - 0 \cdot a \left.\right) \mathbf{j} \textrm{ }\textrm{ } + \textrm{ }\textrm{ } \left(\right. \left(\right. 2 a \left.\right) \cdot \left(\right. - a \left.\right) - 0 \cdot a \left.\right) \mathbf{k} .\)
Suy ra
\(\mathbf{n}_{1} = \left(\right. 0 , \textrm{ } - 2 a h , \textrm{ } - 2 a^{2} \left.\right) .\)
\(cos \varphi \textrm{ }\textrm{ } = \textrm{ }\textrm{ } \frac{\mid \mathbf{n}_{1} \cdot \mathbf{n}_{2} \mid}{\mid \mathbf{n}_{1} \mid \textrm{ } \mid \mathbf{n}_{2} \mid} \textrm{ }\textrm{ } = \textrm{ }\textrm{ } \frac{\mid \left(\right. 0 , \textrm{ } - 2 a h , \textrm{ } - 2 a^{2} \left.\right) \cdot \left(\right. 0 , 0 , 1 \left.\right) \mid}{\left(\right. 2 a \sqrt{\textrm{ } h^{2} + a^{2} \textrm{ }} \left.\right) \times 1} \textrm{ }\textrm{ } = \textrm{ }\textrm{ } \frac{\textrm{ } 2 a^{2} \textrm{ }}{\textrm{ } 2 a \textrm{ } \sqrt{\textrm{ } h^{2} + a^{2} \textrm{ }} \textrm{ }} \textrm{ }\textrm{ } = \textrm{ }\textrm{ } \frac{a}{\sqrt{\textrm{ } h^{2} + a^{2} \textrm{ }}} \textrm{ } .\)
Theo đề: \(\varphi = 60^{\circ}\). Do đó
\(cos 60^{\circ} = \frac{1}{2} = \frac{\textrm{ } a \textrm{ }}{\sqrt{\textrm{ } h^{2} + a^{2} \textrm{ }}} \textrm{ }\textrm{ } \Longrightarrow \textrm{ }\textrm{ } \sqrt{\textrm{ } h^{2} + a^{2} \textrm{ }} \textrm{ }\textrm{ } = \textrm{ }\textrm{ } 2 a \textrm{ }\textrm{ } \Longrightarrow \textrm{ }\textrm{ } h^{2} + a^{2} = 4 a^{2} \textrm{ }\textrm{ } \Longrightarrow \textrm{ }\textrm{ } h = a \sqrt{3} \left(\right. \text{l} \overset{ˊ}{\hat{\text{a}}} \text{y}\&\text{nbsp}; h > 0 \left.\right) .\)
\(S = \left(\right. a , \textrm{ } a , \textrm{ } a \sqrt{3} \left.\right) .\)
2.2. Tính khoảng cách từ \(H = \left(\right. a , a , 0 \left.\right)\) đến ba mặt phẳng
(a) Khoảng cách từ \(H\) đến mặt phẳng \(\left(\right. S A B \left.\right)\)
\(A = \left(\right. 0 , \textrm{ } 0 , \textrm{ } 0 \left.\right) , B = \left(\right. 0 , \textrm{ } 2 a , \textrm{ } 0 \left.\right) , S = \left(\right. a , \textrm{ } a , \textrm{ } a \sqrt{3} \left.\right) .\)
\(\overset{\rightarrow}{A B} = B - A = \left(\right. 0 , \textrm{ } 2 a , \textrm{ } 0 \left.\right) , \overset{\rightarrow}{A S} = S - A = \left(\right. a , \textrm{ } a , \textrm{ } a \sqrt{3} \left.\right) .\)
\(\mathbf{n}_{\textrm{ } S A B} = \overset{\rightarrow}{A B} \times \overset{\rightarrow}{A S} :\)
\(\mathbf{n}_{\textrm{ } S A B} = \mid \mathbf{i} & \mathbf{j} & \mathbf{k} \\ 0 & 2 a & 0 \\ a & a & a \sqrt{3} \mid = \left(\right. \textrm{ } 2 a \cdot a \sqrt{3} - 0 \cdot a \textrm{ } \left.\right) \mathbf{i} \textrm{ }\textrm{ } - \textrm{ }\textrm{ } \left(\right. \textrm{ } 0 \cdot a \sqrt{3} - 0 \cdot a \textrm{ } \left.\right) \mathbf{j} \textrm{ }\textrm{ } + \textrm{ }\textrm{ } \left(\right. \textrm{ } 0 \cdot a - 2 a \cdot a \textrm{ } \left.\right) \mathbf{k} .\)
Suy ra
\(\mathbf{n}_{\textrm{ } S A B} \textrm{ }\textrm{ } = \textrm{ }\textrm{ } \left(\right. \textrm{ } 2 a^{2} \sqrt{3} , \textrm{ } 0 , \textrm{ } - 2 a^{2} \textrm{ } \left.\right) .\)
\(\mathbf{n}_{\textrm{ } S A B} \cdot \left(\right. x , y , z - 0 \left.\right) \textrm{ }\textrm{ } = \textrm{ }\textrm{ } 0 \textrm{ }\textrm{ } \Longrightarrow \textrm{ }\textrm{ } \left(\right. 2 a^{2} \sqrt{3} \left.\right) \textrm{ } x \textrm{ }\textrm{ } + \textrm{ }\textrm{ } 0 \cdot y \textrm{ }\textrm{ } + \textrm{ }\textrm{ } \left(\right. - 2 a^{2} \left.\right) \textrm{ } z \textrm{ }\textrm{ } = \textrm{ }\textrm{ } 0 ,\)
hay rút gọn chia cho \(2 a^{2}\):
\(\sqrt{3} \textrm{ } x \textrm{ }\textrm{ } - \textrm{ }\textrm{ } z \textrm{ }\textrm{ } = \textrm{ }\textrm{ } 0 \Longleftrightarrow z = \sqrt{3} \textrm{ } x .\)
\(d = \frac{\mid A x_{0} + B y_{0} + C z_{0} + D \mid}{\sqrt{\textrm{ } A^{2} + B^{2} + C^{2} \textrm{ }}} .\)
\(\mid \sqrt{3} \cdot a \textrm{ }\textrm{ } + \textrm{ }\textrm{ } 0 \cdot a \textrm{ }\textrm{ } - \textrm{ }\textrm{ } 1 \cdot 0 + 0 \mid = \mid \textrm{ } a \sqrt{3} \mid = a \sqrt{3} .\)
\(d \left(\right. H , \textrm{ } \left(\right. S A B \left.\right) \left.\right) = \frac{\textrm{ } a \sqrt{3} \textrm{ }}{2} \textrm{ } .\)
Kết quả (a):
\(\boxed{d \left(\right. H , \left(\right. S A B \left.\right) \left.\right) \textrm{ }\textrm{ } = \textrm{ }\textrm{ } \frac{a \sqrt{3}}{2} .}\)
(b) Khoảng cách từ \(H\) đến mặt phẳng \(\left(\right. S C D \left.\right)\)
\(C = \left(\right. \textrm{ } 2 a , \textrm{ } 2 a , \textrm{ } 0 \left.\right) , D = \left(\right. \textrm{ } 3 a , \textrm{ } 0 , \textrm{ } 0 \left.\right) , S = \left(\right. \textrm{ } a , \textrm{ } a , \textrm{ } a \sqrt{3} \left.\right) .\)
\(\mathbf{n}_{\textrm{ } S C D} = \overset{\rightarrow}{C D} \textrm{ } \times \textrm{ } \overset{\rightarrow}{C S} .\)
Tính nhanh:
\(\mathbf{n}_{\textrm{ } S C D} = \mid \mathbf{i} & \mathbf{j} & \mathbf{k} \\ a & - 2 a & 0 \\ - a & - a & a \sqrt{3} \mid = \left(\right. \textrm{ } \left(\right. - 2 a \left.\right) \cdot \left(\right. a \sqrt{3} \left.\right) - 0 \cdot \left(\right. - a \left.\right) \left.\right) \mathbf{i} \textrm{ }\textrm{ } - \textrm{ }\textrm{ } \left(\right. a \cdot a \sqrt{3} - 0 \cdot \left(\right. - a \left.\right) \left.\right) \mathbf{j} \textrm{ }\textrm{ } + \textrm{ }\textrm{ } \left(\right. a \cdot \left(\right. - a \left.\right) - \left(\right. - 2 a \left.\right) \cdot \left(\right. - a \left.\right) \left.\right) \mathbf{k} .\)
Suy ra
\(\mathbf{n}_{\textrm{ } S C D} = \left(\right. \textrm{ } - 2 a^{2} \sqrt{3} , \textrm{ }\textrm{ } - \textrm{ } a^{2} \sqrt{3} , \textrm{ }\textrm{ } \left(\right. - \textrm{ } a^{2} \textrm{ }\textrm{ } - \textrm{ }\textrm{ } 2 a^{2} \left.\right) \left.\right) = \left(\right. \textrm{ } - 2 \sqrt{3} \textrm{ } a^{2} , \textrm{ } - \sqrt{3} \textrm{ } a^{2} , \textrm{ } - 3 a^{2} \left.\right) .\)
Ta có thể rút gọn \(a^{2}\) chung; nhưng chỉ cần tỉ lệ để tính khoảng cách.
\(\mathbf{n}_{\textrm{ } S C D} \cdot \left(\right. \left(\right. x , y , z \left.\right) - C \left.\right) \textrm{ }\textrm{ } = \textrm{ }\textrm{ } 0.\)
\(- 2 \sqrt{3} \textrm{ } a^{2} \textrm{ } \left(\right. x - 2 a \left.\right) \textrm{ }\textrm{ } - \textrm{ }\textrm{ } \sqrt{3} \textrm{ } a^{2} \textrm{ } \left(\right. y - 2 a \left.\right) \textrm{ }\textrm{ } - \textrm{ }\textrm{ } 3 a^{2} \textrm{ } \left(\right. z - 0 \left.\right) \textrm{ }\textrm{ } = \textrm{ }\textrm{ } 0.\)
\(2 \sqrt{3} \textrm{ } \left(\right. x - 2 a \left.\right) \textrm{ }\textrm{ } + \textrm{ }\textrm{ } \sqrt{3} \textrm{ } \left(\right. y - 2 a \left.\right) \textrm{ }\textrm{ } + \textrm{ }\textrm{ } 3 \textrm{ } z = 0 ,\)
hay
\(2 \sqrt{3} \textrm{ } x \textrm{ }\textrm{ } + \textrm{ }\textrm{ } \sqrt{3} \textrm{ } y \textrm{ }\textrm{ } + \textrm{ }\textrm{ } 3 \textrm{ } z \textrm{ }\textrm{ } - \textrm{ }\textrm{ } 6 a \sqrt{3} = 0.\)
Đó là phương trình rút gọn của plane \(\left(\right. S C D \left.\right)\).
\(2 \sqrt{3} \cdot a \textrm{ }\textrm{ } + \textrm{ }\textrm{ } \sqrt{3} \cdot a \textrm{ }\textrm{ } + \textrm{ }\textrm{ } 3 \cdot 0 \textrm{ }\textrm{ } - \textrm{ }\textrm{ } 6 a \sqrt{3} = \left(\right. 2 a \sqrt{3} + a \sqrt{3} - 6 a \sqrt{3} \left.\right) = - \textrm{ } 3 a \sqrt{3} .\)
Giá trị tuyệt đối \(\mid - 3 a \sqrt{3} \mid = 3 a \sqrt{3} .\)
\(\sqrt{\textrm{ } \left(\right. 2 \sqrt{3} \left.\right)^{2} + \left(\right. \sqrt{3} \left.\right)^{2} + 3^{2} \textrm{ }} = \sqrt{\textrm{ } 4 \cdot 3 \textrm{ }\textrm{ } + \textrm{ }\textrm{ } 3 \textrm{ }\textrm{ } + \textrm{ }\textrm{ } 9 \textrm{ }} = \sqrt{\textrm{ } 12 + 3 + 9 \textrm{ }} = \sqrt{24} = 2 \sqrt{6} .\)
\(d \left(\right. H , \textrm{ } \left(\right. S C D \left.\right) \left.\right) = \frac{\textrm{ } 3 a \sqrt{3} \textrm{ }}{\textrm{ } 2 \sqrt{6} \textrm{ }} = \frac{3 a \sqrt{3}}{2 \sqrt{6}} = \frac{3 a \sqrt{3} \textrm{ } \sqrt{6}}{2 \cdot 6} = \frac{3 a \sqrt{18}}{12} = \frac{\textrm{ } 3 a \textrm{ } \left(\right. 3 \sqrt{2} \left.\right) \textrm{ }}{12} = \frac{3 a \sqrt{2}}{4} .\)
Kết quả (b):
\(\boxed{d \left(\right. H , \left(\right. S C D \left.\right) \left.\right) \textrm{ }\textrm{ } = \textrm{ }\textrm{ } \frac{3 a \sqrt{2}}{4} .}\)
(c) Khoảng cách từ \(H\) đến mặt phẳng \(\left(\right. S B D \left.\right)\)
\(B = \left(\right. 0 , \textrm{ } 2 a , \textrm{ } 0 \left.\right) , D = \left(\right. 3 a , \textrm{ } 0 , \textrm{ } 0 \left.\right) , S = \left(\right. a , \textrm{ } a , \textrm{ } a \sqrt{3} \left.\right) .\)
\(\mathbf{n}_{\textrm{ } S B D} = \mid \mathbf{i} & \mathbf{j} & \mathbf{k} \\ 3 a & - 2 a & 0 \\ a & - a & a \sqrt{3} \mid = \left(\right. \textrm{ } \left(\right. - 2 a \left.\right) \cdot \left(\right. a \sqrt{3} \left.\right) \textrm{ }\textrm{ } - \textrm{ }\textrm{ } 0 \cdot \left(\right. - a \left.\right) \left.\right) \mathbf{i} \textrm{ }\textrm{ } - \textrm{ }\textrm{ } \left(\right. \textrm{ } \left(\right. 3 a \left.\right) \cdot \left(\right. a \sqrt{3} \left.\right) \textrm{ }\textrm{ } - \textrm{ }\textrm{ } 0 \cdot a \left.\right) \mathbf{j} \textrm{ }\textrm{ } + \textrm{ }\textrm{ } \left(\right. \textrm{ } \left(\right. 3 a \left.\right) \cdot \left(\right. - a \left.\right) \textrm{ }\textrm{ } - \textrm{ }\textrm{ } \left(\right. - 2 a \left.\right) \cdot a \left.\right) \mathbf{k} .\)
Suy ra
\(\mathbf{n}_{\textrm{ } S B D} = \left(\right. \textrm{ } - 2 a^{2} \sqrt{3} , \textrm{ } - 3 a^{2} \sqrt{3} , \textrm{ } \left(\right. - 3 a^{2} + 2 a^{2} \left.\right) \left.\right) = \left(\right. \textrm{ } - 2 \sqrt{3} \textrm{ } a^{2} , \textrm{ } - 3 \sqrt{3} \textrm{ } a^{2} , \textrm{ } - a^{2} \left.\right) .\)
\(\mathbf{n}_{\textrm{ } S B D} \cdot \left(\right. \left(\right. x , y , z \left.\right) - B \left.\right) = 0 \Longleftrightarrow - 2 \sqrt{3} a^{2} \textrm{ } \left(\right. x - 0 \left.\right) \textrm{ }\textrm{ } - \textrm{ }\textrm{ } 3 \sqrt{3} a^{2} \textrm{ } \left(\right. y - 2 a \left.\right) \textrm{ }\textrm{ } - \textrm{ }\textrm{ } a^{2} \textrm{ } \left(\right. z - 0 \left.\right) \textrm{ }\textrm{ } = \textrm{ }\textrm{ } 0.\)
\(2 \sqrt{3} \textrm{ } x \textrm{ }\textrm{ } + \textrm{ }\textrm{ } 3 \sqrt{3} \textrm{ } \left(\right. y - 2 a \left.\right) \textrm{ }\textrm{ } + \textrm{ }\textrm{ } z = 0 \textrm{ }\textrm{ } \Longleftrightarrow \textrm{ }\textrm{ } 2 \sqrt{3} \textrm{ } x \textrm{ }\textrm{ } + \textrm{ }\textrm{ } 3 \sqrt{3} \textrm{ } y \textrm{ }\textrm{ } + \textrm{ }\textrm{ } z \textrm{ }\textrm{ } - \textrm{ }\textrm{ } 6 a \sqrt{3} = 0.\)
\(2 \sqrt{3} \textrm{ } a \textrm{ }\textrm{ } + \textrm{ }\textrm{ } 3 \sqrt{3} \textrm{ } a \textrm{ }\textrm{ } + \textrm{ }\textrm{ } 0 \textrm{ }\textrm{ } - \textrm{ }\textrm{ } 6 a \sqrt{3} = \left(\right. 2 a \sqrt{3} + 3 a \sqrt{3} - 6 a \sqrt{3} \left.\right) = - \textrm{ } a \sqrt{3} .\)
Giá trị tuyệt đối: \(\mid - \textrm{ } a \sqrt{3} \mid = a \sqrt{3} .\)
\(\sqrt{\textrm{ } \left(\right. 2 \sqrt{3} \left.\right)^{2} + \left(\right. 3 \sqrt{3} \left.\right)^{2} + 1^{2} \textrm{ }} = \sqrt{\textrm{ } 12 + 27 + 1 \textrm{ }} = \sqrt{40} = 2 \sqrt{10} .\)
\(d \left(\right. H , \textrm{ } \left(\right. S B D \left.\right) \left.\right) = \frac{\textrm{ } a \sqrt{3} \textrm{ }}{\textrm{ } 2 \sqrt{10} \textrm{ }} = \frac{a \sqrt{3}}{2 \sqrt{10}} = \frac{a \sqrt{30}}{20} \left(\right. \text{v} \overset{ˋ}{\imath} \&\text{nbsp}; \sqrt{3} / \sqrt{10} = \sqrt{30} / 10 \left.\right) .\)
Kết quả (c):
\(\boxed{d \left(\right. H , \left(\right. S B D \left.\right) \left.\right) \textrm{ }\textrm{ } = \textrm{ }\textrm{ } \frac{a \sqrt{30}}{20} .}\)
Tóm tắt Câu 29: