Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

\(a,=x^2+x+4x+4=\left(x+1\right)\left(x+4\right)\\ b,=x^2+2x-3x-6=\left(x-3\right)\left(x+2\right)\\ c,=x^2-2x-3x+6=\left(x-2\right)\left(x-3\right)\\ d,=3\left(x^2-2x+5x-10\right)=3\left(x-2\right)\left(x+5\right)\\ e,=-3x^2+6x-x+2=\left(x-2\right)\left(1-3x\right)\\ f,=x^2-x-6x+6=\left(x-1\right)\left(x-6\right)\\ h,=4\left(x^2-3x-6x+18\right)=4\left(x-3\right)\left(x-6\right)\\ i,=3\left(3x^2-3x-8x+5\right)=3\left(x-1\right)\left(3x-8\right)\\ k,=-\left(2x^2+x+4x+2\right)=-\left(2x+1\right)\left(x+2\right)\\ l,=x^2-2xy-5xy+10y^2=\left(x-2y\right)\left(x-5y\right)\\ m,=x^2-xy-2xy+2y^2=\left(x-y\right)\left(x-2y\right)\\ n,=x^2+xy-3xy-3y^2=\left(x+y\right)\left(x-3y\right)\)

Bài 6
\(a,ĐK:x\ne\pm5\\ b,P=\dfrac{x-5+2x+10-2x-10}{\left(x-5\right)\left(x+5\right)}=\dfrac{x-5}{\left(x-5\right)\left(x+5\right)}=\dfrac{1}{x+5}\\ c,P=-3\Leftrightarrow\dfrac{1}{x+5}=-3\Leftrightarrow-3\left(x+5\right)=1\Leftrightarrow x=-\dfrac{16}{3}\\ \Leftrightarrow Q=\left(3x-7\right)^2=\left[3\cdot\left(-\dfrac{16}{3}\right)-7\right]^2=529\)
Bài 7:
\(a,ĐK:x\ne\pm3\\ b,P=\dfrac{3x-9+x+3+18}{\left(x-3\right)\left(x+3\right)}=\dfrac{4\left(x+3\right)}{\left(x-3\right)\left(x+3\right)}=\dfrac{4}{x-3}\\ b,P=4\Leftrightarrow4\left(x-3\right)=4\Leftrightarrow x=4\)

Bài 6:
a: Xét ΔHAD vuông tại H và ΔHBA vuông tại H có
\(\widehat{HAD}=\widehat{HBA}\)
Do đó: ΔHAD\(\sim\)ΔHBA
b: Ta có: ΔHAD\(\sim\)ΔHBA
nên HA/HB=HD/HA
hay \(HA^2=HB\cdot HD\)

23.27. \(x^2-y^2-2x+1\)
\(=\left(x-1\right)^2-y^2\)
\(=\left(x-1-y\right)\left(x-1+y\right)\)
23.25.
\(\left(x^2-4x\right)^2+\left(x-2\right)^2-10\)
\(=\left(x^2-4x\right)^2-4+\left(x-2\right)^2-6\)
\(=\left(x^2-4x+4\right)\left(x^2-4x-4\right)+x^2-4x+4-6\)
\(=\left(x^2-4x+4\right)\left(x^2-4x-10\right)\)
23.23
\(x^3-2x^2-6x+27\)
\(=\left(x^3+27\right)-2x\left(x+3\right)\)
\(=\left(x+3\right)\left(x^2-3x+9-2x\right)\)
\(=\left(x+3\right)\left(x^2-5x+9\right)\)

a) ĐKXĐ: \(\left\{{}\begin{matrix}2x+3\ne0\\2x+1\ne0\\\left(2x+3\right)\left(2x+1\right)\ne0\end{matrix}\right.\)\(\Leftrightarrow\left\{{}\begin{matrix}x\ne-\dfrac{3}{2}\\x\ne-\dfrac{1}{2}\\\left(2x+3\right)\left(2x+1\right)\ne0\end{matrix}\right.\)\(\Leftrightarrow\left\{{}\begin{matrix}x\ne-\dfrac{3}{2}\\x\ne-\dfrac{1}{2}\end{matrix}\right.\)
b) \(\Rightarrow P=\dfrac{2\left(2x+1\right)+3\left(2x+3\right)-6x-5}{\left(2x+3\right)\left(2x+1\right)}\)
\(\Rightarrow P=\dfrac{4x+2+6x+9-6x-5}{\left(2x+3\right)\left(2x+1\right)}\)
\(\Rightarrow P=\dfrac{4x+6}{\left(2x+3\right)\left(2x+1\right)}\)
\(\Rightarrow P=\dfrac{2\left(2x+3\right)}{\left(2x+3\right)\left(2x+1\right)}\)
\(\Rightarrow P=\dfrac{2}{2x+1}\)
c) \(P=-1\Rightarrow\dfrac{2}{2x+1}=-1\\ \Rightarrow2=-2x-1\\ \Rightarrow2x=-3\\ \Rightarrow x=-\dfrac{3}{2}\)


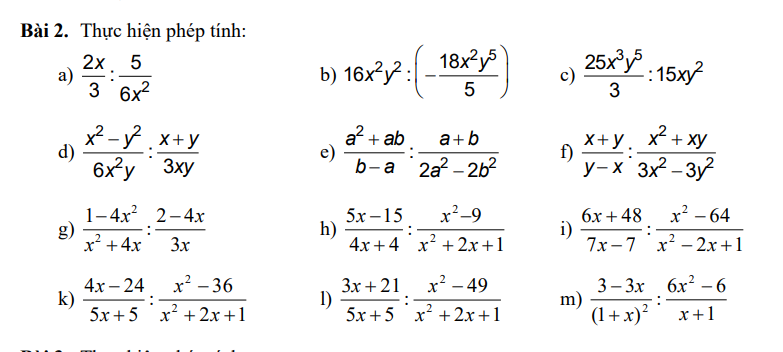
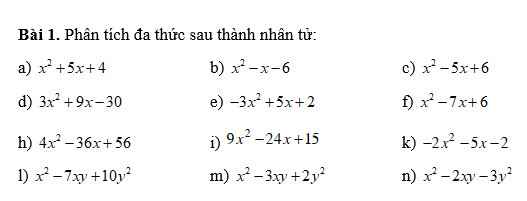





 Giúp vs æ! Cho like ạ! Trong hình là đề ạ! Giúp vs cần gấp lắm! Mơn anh chị!
Giúp vs æ! Cho like ạ! Trong hình là đề ạ! Giúp vs cần gấp lắm! Mơn anh chị!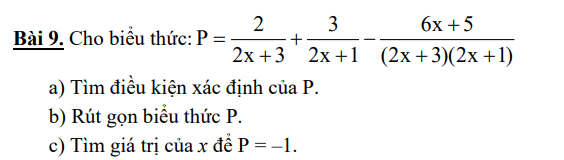

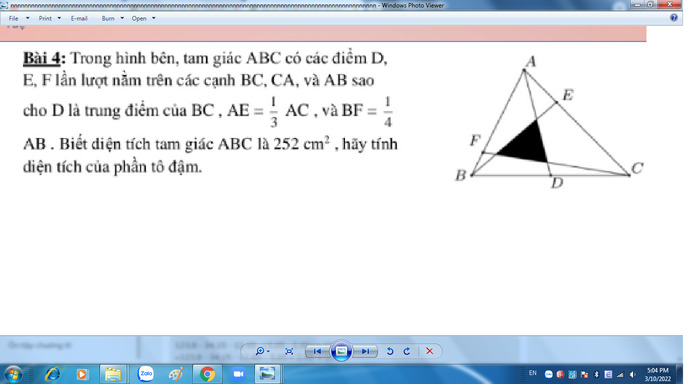


 mọi người giải giúp em với ạ em đang cần gấp lắm ạ
mọi người giải giúp em với ạ em đang cần gấp lắm ạ