K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
10 tháng 10 2021
\(1,\\ a,\Leftrightarrow\left(x-5\right)\left(x-2\right)=0\Leftrightarrow\left[{}\begin{matrix}x=5\\x=2\end{matrix}\right.\\ b,\Leftrightarrow\left(x-4\right)\left(3x-1\right)=0\Leftrightarrow\left[{}\begin{matrix}x=4\\x=\dfrac{1}{3}\end{matrix}\right.\\ c,\Leftrightarrow\left(x-7\right)\left(x+2\right)=0\Leftrightarrow\left[{}\begin{matrix}x=7\\x=-2\end{matrix}\right.\\ d,\Leftrightarrow\left(2x+3\right)\left(2x-1\right)=0\Leftrightarrow\left[{}\begin{matrix}x=-\dfrac{3}{2}\\x=\dfrac{1}{2}\end{matrix}\right.\\ 2,\\ a,\Leftrightarrow\left(x+5\right)^2=0\Leftrightarrow x=-5\\ b,\Leftrightarrow\left(x-\dfrac{1}{2}\right)^2=0\Leftrightarrow x=\dfrac{1}{2}\\ c,\Leftrightarrow\left(x-9\right)^2=0\Leftrightarrow x=9\\ d,\Leftrightarrow\left(x-3\right)^3=0\Leftrightarrow x=3\\ e,\Leftrightarrow3x\left(x^2-2x+3\right)=0\\ \Leftrightarrow3x\left(x^2-2x+1+2\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}x=0\\\left(x-1\right)^2+2=0\left(vô.nghiệm\right)\end{matrix}\right.\\ \Leftrightarrow x=0\)
\(f,\Leftrightarrow3x\left(x^2-4x+4\right)=0\Leftrightarrow\left[{}\begin{matrix}x=0\\x=2\end{matrix}\right.\)
10 tháng 10 2021
Bài 1:
a) \(\Rightarrow\left(x-5\right)\left(x-2\right)=0\)
\(\Rightarrow\left[{}\begin{matrix}x=5\\x=2\end{matrix}\right.\)
b) \(\Rightarrow3x\left(x-4\right)-\left(x-4\right)=0\)
\(\Rightarrow\left(x-4\right)\left(3x-1\right)=0\)
\(\Rightarrow\left[{}\begin{matrix}x=4\\x=\dfrac{1}{3}\end{matrix}\right.\)
c) \(\Rightarrow\left(x-7\right)\left(x+2\right)=0\)
\(\Rightarrow\left[{}\begin{matrix}x=7\\x=-2\end{matrix}\right.\)
d) \(\Rightarrow\left(2x+3\right)\left(2x-1\right)=0\)
\(\Rightarrow\left[{}\begin{matrix}x=-\dfrac{3}{2}\\x=\dfrac{1}{2}\end{matrix}\right.\)
Bài 2:
a) \(\Rightarrow\left(x+5\right)^2=0\Rightarrow x=-5\)
b) \(\Rightarrow\left(x-\dfrac{1}{2}\right)^2=0\Rightarrow x=\dfrac{1}{2}\)
c) \(\Rightarrow\left(x-9\right)^2=0\Rightarrow x=9\)
d) \(\Rightarrow\left(x-3\right)^3=0\Rightarrow x=3\)
e) \(\Rightarrow3x\left(x^2-6x+9\right)=0\)
\(\Rightarrow3x\left(x-3\right)^2=0\)
\(\Rightarrow\left[{}\begin{matrix}x=0\\x=3\end{matrix}\right.\)
f) \(\Rightarrow3x\left(x^2-4x+4\right)=0\)
\(\Rightarrow3x\left(x-2\right)^2=0\)
\(\Rightarrow\left[{}\begin{matrix}x=0\\x=2\end{matrix}\right.\)
12 tháng 4 2017
<=> 7x-1>0 và 3-2x<0 hoặc 7x-1<0 và 3-2x>0
<=> x>1/7 và x>3/2 hoặc x<1/7 và x<3/2
<=> x>3/2 hoặc x<1/7
Vậy với x>3/2 hoặc x<1/7 thì thỏa mãn đề bài
(những chữ "hoặc" và chữ "và" bạn có thể thay bằng dấu ngoặc vuông và ngoặc nhọn nhé!!!)
Have a good day!!!
5 tháng 3 2022
tất cả các ý mình cm thành cụm luôn nhé
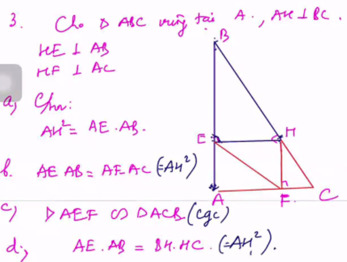
Xét tam giác AHE và tam giác ABH ta có
^A _ chung
^AHE = ABH = 900
Vậy tam giác AHE ~ tam giác ABH (g.g)
\(\dfrac{AH}{AB}=\dfrac{AE}{AH}\Rightarrow AH^2=AE.AB\)(1)
Xét tam giác AHC và tam giác AFH có
^A _ chung
^AHC = ^AFH = 900
Vậy tam giác AHC ~ tam giác AFH (g.g)
\(\dfrac{AH}{AF}=\dfrac{AC}{AH}\Rightarrow AH^2=AF.AC\)(2)
Từ (1) ; (2) suy ra \(\dfrac{AE}{AC}=\dfrac{AF}{AB}\)(*)
Xét tam giác AEF và tam giác ABC có
^A _ chung ; có (*)
Vậy tam giác AEF ~ tam giác ABC (c.g.c)
6 tháng 11 2021
Bài 15:
\(P=\dfrac{x+y-1}{x\left(x+y\right)}+\dfrac{x-y}{2xy}\cdot\dfrac{xy+y^2+xy-y^2}{x\left(x-y\right)\left(x+y\right)}\)
\(=\dfrac{1}{x}\)
T
1
12 tháng 12 2021
\(\dfrac{x+1}{2x^2-x^4}=\dfrac{x+1}{x^2\left(2-x^2\right)}=\dfrac{-\left(x+1\right)\left(x^4+2x^2+4\right)}{x^2\left(x^2-2\right)\left(x^4+2x^2+4\right)}\\ \dfrac{x}{x^4+2x^2+4}=\dfrac{x^3\left(x^2-2\right)}{x^2\left(x^2-2\right)\left(x^4+2x^2+4\right)}\\ \dfrac{2x-1}{x^7-8x}=\dfrac{2x-1}{x\left(x^6-8\right)}=\dfrac{x\left(2x-1\right)}{x^2\left(x^2-2\right)\left(x^4+2x^2+4\right)}\)
14 tháng 12 2016
Chỉ khi x + y + z = 0 mới như vậy.
Cụ thể :
Ta có :
\(x^3+y^3+z^3-3xyz\)
\(=\left(x+y\right)^3+z^3-3xy^2-3x^2y-3xyz\)
\(=\left(x+y+z\right)\left[\left(x+y\right)^2+z^2-\left(x+y\right)z\right]-3xy\left(x+y+z\right)\)
\(=\left(x+y+z\right)\left[x^2+y^2+2xy+z^2-xz-yz-3xy\right]\)
\(=0\) là BS xyz
EG
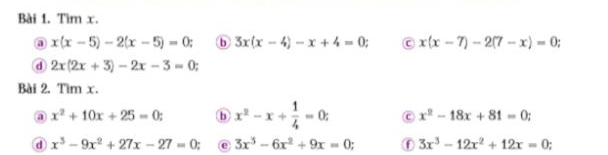
cd
4