K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
7 tháng 1 2018
a.\(\hept{\begin{cases}3x-2y=1\\2x+4y=3\end{cases}}\)
<=>\(\hept{\begin{cases}6x-4y=2\\2x+4y=3\end{cases}}\)
<=>\(\hept{\begin{cases}8x=5\\2x+4y=3\end{cases}}\)
<=>\(\hept{\begin{cases}x=\frac{5}{8}\\2\cdot\frac{5}{8}+4y=3\end{cases}}\)
<=>\(\hept{\begin{cases}x=\frac{5}{8}\\4y=\frac{7}{4}\end{cases}}\)
<=>\(\hept{\begin{cases}x=\frac{5}{8}\\y=\frac{7}{16}\end{cases}}\)
7 tháng 1 2018
a) \(\hept{\begin{cases}3x-2y=1\\2x+4y=3\end{cases}}\Rightarrow\hept{\begin{cases}6x-4y=2\\2x+4y=3\end{cases}}\)
\(\Leftrightarrow\hept{\begin{cases}8x=5\\2x+4y=3\end{cases}}\Leftrightarrow\hept{\begin{cases}x=\frac{5}{8}\\\frac{5}{4}+4y=3\end{cases}}\)
\(\Leftrightarrow\hept{\begin{cases}x=\frac{5}{8}\\4y=\frac{7}{4}\end{cases}}\Leftrightarrow\hept{\begin{cases}x=\frac{5}{8}\\y=\frac{7}{16}\end{cases}}\)
vậy hpt có nghiệm duy nhất \(\left(x;y\right)=\left(\frac{5}{8};\frac{7}{16}\right)\)
b) \(\hept{\begin{cases}4x-3y=1\\-x+2y=1\end{cases}}\Leftrightarrow\hept{\begin{cases}8x-6y=2\\-3x+6y=3\end{cases}}\)
\(\Leftrightarrow\hept{\begin{cases}5x=5\\-3x+6y=3\end{cases}}\Leftrightarrow\hept{\begin{cases}x=1\\-3+6y=3\end{cases}}\)
\(\Leftrightarrow\hept{\begin{cases}x=1\\y=1\end{cases}}\)
vậy hpt có nghiệm duy nhất \(\left(x;y\right)=\left(1;1\right)\)
CM
10 tháng 2 2018
(II):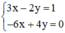 
Xét: (d): 3x – 2y = 1 hay (d):
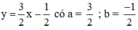
(d’): -6x + 4y = 0 hay (d’):
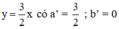
Ta có: a = a’ ; b ≠ b’ ⇒ (d) // (d’)
⇒ Hệ (II) vô nghiệm.
12 tháng 3 2020
\(\hept{\begin{cases}2x^2+y^2-4x+2y=1\\3x^2-2y^2-6x-4y=5\end{cases}}\)
\(\Leftrightarrow\hept{\begin{cases}2\left(x^2-2x+1\right)+\left(y^2+2y+1\right)=4\\3\left(x^2-2x+1\right)-2\left(y^2+2y+1\right)=6\end{cases}}\)
\(\Leftrightarrow\hept{\begin{cases}2\left(x-1\right)^2+\left(y+1\right)^2=4\\3\left(x-1\right)^2-2\left(y+1\right)^2=6\end{cases}}\)
Đặt \(\hept{\begin{cases}\left(x-1\right)^2=a\left(\ge0\right)\\\left(y+1\right)^2=b\left(\ge0\right)\end{cases}}\)
=> hệ phương trình \(\Leftrightarrow\hept{\begin{cases}2a+b=4\\3a-2b=6\end{cases}}\)
Tự giải tiếp nhé
24 tháng 7 2019
Hệ phương trình tương đương \(\hept{\begin{cases}4x^2+2y^2-8x+4y=2\\3x^2-2y^2-6x-4y=5\end{cases}}\)
Cộng vế theo vế ta có phương trình:
\(7x^2-14x=7\Leftrightarrow7x^2-14x-7=0\)
Y
24 tháng 7 2019
\(\left\{{}\begin{matrix}2x^2+y^2-4x+2y=1\\3x^2-2y^2-6x-4y=5\end{matrix}\right.\\ \Leftrightarrow\left\{{}\begin{matrix}4x^2+2y^2-8x+4y=2\left(1\right)\\3x^2-2y^2-6x-4y=5\left(2\right)\end{matrix}\right.\)
Lấy (1) cộng (2) ta được: \(7x^2-14x=7\Leftrightarrow x^2-2x=1\Leftrightarrow x^2-2x-1=0\Leftrightarrow\left\{{}\begin{matrix}x_1=1+\sqrt{2}\\x_2=1-\sqrt{2}\end{matrix}\right.\)
+) T/h 1: \(x=1+\sqrt{2}\) thay vào (1) ta có:
\(\left(1\right)\Leftrightarrow2\left(1+\sqrt{2}\right)^2+y^2-4\left(1+\sqrt{2}\right)+2y=1\\ \Leftrightarrow2\left(1+2\sqrt{2}+2\right)-4\left(1+\sqrt{2}\right)+y^2+2y-1=0\\ \Leftrightarrow2+4\sqrt{2}+4-4-4\sqrt{2}+y^2+2y-1=0\\ \Leftrightarrow y^2+2y+1=0\\ \Leftrightarrow\left(y+1\right)^2=0\Leftrightarrow y=-1\)
+) T/h 2 : \(x=1-\sqrt{2}\) thay vào (1) ta có:
\(\left(1\right)\Leftrightarrow2\left(1-\sqrt{2}\right)^2+y^2-4\left(1-\sqrt{2}\right)+2y=1\\ \Leftrightarrow2\left(1-2\sqrt{2}+2\right)-4\left(1-\sqrt{2}\right)+y^2+2y-1=0\\ \Leftrightarrow2-4\sqrt{2}+4-4+4\sqrt{2}+y^2+2y-1=0\\ \Leftrightarrow y^2+2y+1=0\\ \Leftrightarrow\left(y+1\right)^2=0\Leftrightarrow y=-1\)
Vậy hệ phương trình có nghiệm là: \(\left(x;y\right)=\left(1+\sqrt{2};-1\right);\left(1-\sqrt{2};-1\right)\)
CM
21 tháng 4 2018
a) (I):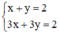 
Xét (d): x + y = 2 hay (d): y = -x + 2 có a = -1; b = 2.
(d’) 3x + 3y = 2 hay (d’): y = -x +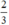 
có a’ = -1 ; b’ =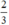 
Ta có: a = a’ ; b ≠ b’ ⇒ (d) // (d’)
⇒ Hệ (I) vô nghiệm.
b) (II):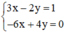 
Xét: (d): 3x – 2y = 1 hay (d):
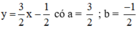
(d’): -6x + 4y = 0 hay (d’):
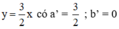
Ta có: a = a’ ; b ≠ b’ ⇒ (d) // (d’)
⇒ Hệ (II) vô nghiệm.
Kiến thức áp dụng
+ Xét hệ (I): 
Gọi (d): ax + by = c và (d’): a’x + b’y = c’.
Số nghiệm của hệ (I) phụ thuộc vào vị trí tương đối của (d) và (d’).
(d) cắt (d’) ⇒ hệ (I) có nghiệm duy nhất.
(d) // (d’) ⇒ hệ (I) vô nghiệm
(d) ≡ (d’) ⇒ hệ (I) có vô số nghiệm.
+ Cho đường thẳng (d): y = ax + b và (d’): y = a’x + b’.
(d) cắt (d’) ⇔ a ≠ a’
(d) // (d’) ⇔ a = a’ và b ≠ b’
(d) trùng (d’) ⇔ a = a’ và b = b’.
23 tháng 10 2021
a) \(\hept{\begin{cases}x+y=2\\3x+3y=2\end{cases}}\)
\(\Leftrightarrow\hept{\begin{cases}3x+3y=6\\3x+3y=2\end{cases}}\)
Dễ thấy điều trên là vô lí nên hệ phương trình không có nghiệm
TN
13 tháng 5 2018
Từ \(\left(6x+4y-1\right)\sqrt{x+y+1}=\left(2x+2y+1\right)\sqrt{3x+21y}\)
\(\Leftrightarrow\left(6x+4y-1\right)^2\left(x+y+1\right)=\left(2x+2y+1\right)^2\left(3x+2y\right)\)
\(\Leftrightarrow\left(2x+y-1\right)\left(12x^2+20xy+12x+8y^2+8y-1\right)=0\)
\(\Leftrightarrow x=\frac{-y+1}{2}\) thay vào pt(1)
\(\frac{y^2+2y-35}{4}=0\Leftrightarrow\left(y-5\right)\left(y+7\right)=0\Leftrightarrow\orbr{\begin{cases}y=5\\y=-7\end{cases}}\)
\(\Leftrightarrow\orbr{\begin{cases}y=5\Leftrightarrow x=-2\\y=-7\Leftrightarrow x=4\end{cases}}\)
\(\left\{{}\begin{matrix}3x+2y=4\\6x+4y=1\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}6x+4y=8\\6x+4y=1\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}3x+2y=4\\\text{0=7(vô lí)}\end{matrix}\right.\) ( \(\left(6x-6x\right)+\left(4y-4y\right)=8-1\))
Vậy không có các cặp x;y thỏa mãn