ND
2
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
NC
2
9 tháng 11 2021
Bài 1:
\(a,A=6\sqrt{2}-6\sqrt{2}+2\sqrt{5}=2\sqrt{5}\\ b,B=\dfrac{\sqrt{3}\left(\sqrt{3}-1\right)}{\sqrt{3}-1}+\dfrac{\sqrt{2}\left(\sqrt{2}-1\right)}{\sqrt{2}-1}=\sqrt{3}+\sqrt{2}\\ c,=2\sqrt{3}-6\sqrt{3}+15\sqrt{3}-4\sqrt{3}=7\sqrt{3}\\ d,=1+6\sqrt{3}-\sqrt{3}-1=5\sqrt{3}\\ e,=4\sqrt{2}+\sqrt{2}-6\sqrt{2}+3\sqrt{2}=2\sqrt{2}\)
Bài 2:
\(a,ĐK:x\ge\dfrac{3}{2}\\ PT\Leftrightarrow\sqrt{2x-3}=5\Leftrightarrow2x-3=25\Leftrightarrow x=14\\ b,PT\Leftrightarrow x^2=\sqrt{\dfrac{98}{2}}=\sqrt{49}=7\Leftrightarrow\left[{}\begin{matrix}x=\sqrt{7}\\x=-\sqrt{7}\end{matrix}\right.\\ c,ĐK:x\ge3\\ PT\Leftrightarrow\sqrt{x-3}\left(\sqrt{x+3}+1\right)=0\\ \Leftrightarrow\sqrt{x-3}=0\left(\sqrt{x+3}+1>0\right)\\ \Leftrightarrow x=3\\ d,ĐK:x\ge1\\ PT\Leftrightarrow2\sqrt{x-1}-\sqrt{x-1}+3\sqrt{x-1}=4\\ \Leftrightarrow\sqrt{x-1}=1\Leftrightarrow x=2\left(tm\right)\\ e,PT\Leftrightarrow2x-1=16\Leftrightarrow x=\dfrac{17}{2}\\ f,PT\Leftrightarrow\left|2x-1\right|=\sqrt{3}-1\Leftrightarrow\left[{}\begin{matrix}2x-1=\sqrt{3}-1\\2x-1=1-\sqrt{3}\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{\sqrt{3}}{2}\\x=\dfrac{2-\sqrt{3}}{2}\end{matrix}\right.\)
9 tháng 11 2021
Bài 3:
\(a,Q=\dfrac{1+5}{3-1}=3\\ b,P=\dfrac{x+\sqrt{x}-6+x-2\sqrt{x}-3-x+4\sqrt{x}+9}{\left(\sqrt{x}-3\right)\left(\sqrt{x}+3\right)}\\ P=\dfrac{\sqrt{x}\left(\sqrt{x}+3\right)}{\left(\sqrt{x}-3\right)\left(\sqrt{x}+3\right)}=\dfrac{\sqrt{x}}{\sqrt{x}-3}\\ c,M=\dfrac{\sqrt{x}}{\sqrt{x}-3}\cdot\dfrac{3-\sqrt{x}}{\sqrt{x}+5}=\dfrac{-\sqrt{x}}{\sqrt{x}+5}\)
Vì \(-\sqrt{x}\le0;\sqrt{x}+5>0\) nên \(M< 0\)
Do đó \(\left|M\right|>\dfrac{1}{2}\Leftrightarrow M< -\dfrac{1}{2}\Leftrightarrow-\dfrac{\sqrt{x}}{\sqrt{x}+5}+\dfrac{1}{2}< 0\)
\(\Leftrightarrow\dfrac{2\sqrt{x}-\sqrt{x}-5}{2\left(\sqrt{x}+5\right)}< 0\Leftrightarrow\sqrt{x}-5< 0\left(\sqrt{x}+5>0\right)\\ \Leftrightarrow0\le x< 25\)
Bài 4:
\(a,A=\dfrac{16+2\cdot4+5}{4-3}=29\\ b,B=\dfrac{2\sqrt{x}-9-x+9+2x-3\sqrt{x}-2}{\left(\sqrt{x}-2\right)\left(\sqrt{x}-3\right)}\\ B=\dfrac{x-\sqrt{x}-2}{\left(\sqrt{x}-2\right)\left(\sqrt{x}-3\right)}=\dfrac{\left(\sqrt{x}-2\right)\left(\sqrt{x}+1\right)}{\left(\sqrt{x}-2\right)\left(\sqrt{x}-3\right)}=\dfrac{\sqrt{x}+1}{\sqrt{x}-3}\\ c,P=\dfrac{x+2\sqrt{x}+5}{\sqrt{x}-3}\cdot\dfrac{\sqrt{x}-3}{\sqrt{x}+1}=\dfrac{x+2\sqrt{x}+5}{\sqrt{x}+1}\\ P=\dfrac{\left(\sqrt{x}+1\right)^2+4}{\sqrt{x}+1}=\sqrt{x}+1+\dfrac{4}{\sqrt{x}+1}\\ P\ge2\sqrt{\left(\sqrt{x}+1\right)\cdot\dfrac{4}{\sqrt{x}+1}}=2\sqrt{4}=4\\ P_{min}=4\Leftrightarrow\left(\sqrt{x}+1\right)^2=4\Leftrightarrow\sqrt{x}+1=2\Leftrightarrow x=1\left(tm\right)\)
NP
0
27 tháng 5 2022
\(\left(x+2\right)\left(\dfrac{360}{x}-6\right)=360\)
\(ĐK:x\ne0\)
\(\Leftrightarrow\left(x+2\right)\left(\dfrac{360-6x}{x}\right)=360\)
\(\Leftrightarrow360-6x+\dfrac{720-12x}{x}=360\)
\(\Leftrightarrow360x-6x^2+720-12x=360x\)
\(\Leftrightarrow6x^2+12x-720=0\)
\(\Delta=12^2-4.6.\left(-720\right)\)
\(=17424>0\)
`->` pt có 2 nghiệm
\(\left\{{}\begin{matrix}x_1=\dfrac{-12-\sqrt{17424}}{12}=-12\\x_2=\dfrac{-12+\sqrt{17424}}{12}=10\end{matrix}\right.\) ( tm )
Vậy \(S=\left\{-12;10\right\}\)
2
27 tháng 5 2022
`48/[x+4]+48/[x-4]=5` `ĐK: x \ne +-4`
`<=>[48(x-4)+48(x+4)]/[(x-4)(x+4)]=[5(x+4)(x-4)]/[(x-4)(x+4)]`
`=>48x-192+48x+192=5x^2-80`
`<=>5x^2-96x-80=0`
`<=>5x^2-100+4x-80=0`
`<=>5x(x-20)+4(x-20)=0`
`<=>(x-20)(5x+4)=0`
`<=>` $\left[\begin{matrix} x=20\\ x=\dfrac{-4}{5}\end{matrix}\right.$ (t/m)
Vậy `S={-4/5;20}`
TC
27 tháng 5 2022
ĐK : \(x\ne\pm4\)
\(\Leftrightarrow\cdot\dfrac{48\left(x+4\right)+48\left(x-4\right)}{\left(x+4\right)\left(x-4\right)}=\dfrac{5\left(x+4\right)\left(x-4\right)}{\left(x+4\right)\left(x-4\right)}\)
\(\Leftrightarrow48x+192+48x-192==5x^2-80\)
\(\Leftrightarrow96x=5x^2-80\)
\(\Leftrightarrow5x^2-96x-80=0\)
\(\Leftrightarrow5x^2+4x-100-80=0\)
\(\Leftrightarrow4\left(x-20\right)+5x\left(x-20\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x-20=0\\5x=-4\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=20\\x=-\dfrac{4}{5}\end{matrix}\right.\)
R
6 tháng 11 2015
tick cho mình đi rồi mình gửi bài cho còn không tick thì mình không bày đâu nhé
28 tháng 4 2020
Gọi x là số xe cần chuyển hàng ( x>0)
Ta có mỗi xe xếp 15 tấn thì còn thừa 5 tấn
=> lượng hàng càn chở là: 15x+5
Ta lại có mỗi xe xếp 16 tấn thì còn thiếu 3 tấn
=> lượng hàng cần chở là: 16x-3
<=> x=8
Vậy lượng hàng cần chở là: 15.8+5=125 tấn.
Mình mới làm qua thui, bạn đọc qua rùi sửa lại nhé.
~Chúc học tốt~
S
9 tháng 2 2019
\(\hept{\begin{cases}2x+2y=10-2xy\\x^2+y^2=5\end{cases}}\)
\(\Rightarrow x^2+y^2-10+2xy=5-2\left(x+y\right)\Leftrightarrow\left(x+y\right)\left(x+y\right)-10=5-2\left(x+y\right)\)
\(\text{Đặt: x+y=a}\)
\(a^2-10=5-2a\Rightarrow a^2-10-5+2a=0\Rightarrow a^2+2a-15=0\)
\(\)\(\Leftrightarrow a^2+2a+1=16\Leftrightarrow a+1=\pm4\Leftrightarrow\orbr{\begin{cases}a=-5\\a=3\end{cases}}\)
\(+,a=-5\Rightarrow x+y=-5\)
\(\Rightarrow xy=10\Rightarrow x^2+y^2+10-2xy=0\Rightarrow\left(x-y\right)^2=-10\left(\text{loại}\right)\)
\(+,a=3\Rightarrow x+y=3\Rightarrow xy=2\)
\(\Rightarrow x^2+y^2+10-2xy=11\Rightarrow\left(x-y\right)\left(x-y\right)=1\Rightarrow x-y=\pm1\)
\(\text{Giả sử: x ít nhất bằng y}\)
\(\Rightarrow x-y=1\Rightarrow\hept{\begin{cases}x=2\\y=1\end{cases}}\)
\(y\ge x\Rightarrow\hept{\begin{cases}y=2\\x=1\end{cases}}\)
đến đây thì ez rồi
H
1 tháng 3 2020
b, \(\Delta'=b'^2-ac=\left[-\left(m-1\right)\right]^2-1.\left(-m-3\right)=m^2-2m+1+m+3\)
\(=m^2-m+4=m^2-m+\frac{1}{4}+\frac{15}{4}=\left(m-\frac{1}{2}\right)^2+\frac{15}{4}>0\)
Vậy pt (1) có 2 nghiệm x1,x2 với mọi m
Theo hệ thức vi-et ta có: \(\hept{\begin{cases}x_1+x_2=2\left(m-1\right)\left(2\right)\\x_1x_2=-m-3\left(3\right)\end{cases}}\)
Ta có: \(x_1^2+x_2^2=10\Leftrightarrow\left(x_1+x_2\right)^2-2x_1x_2=10\)
<=>\(4\left(m-1\right)^2-2\left(-m-3\right)=10\)
<=>\(4m^2-8m+4+2m+6=10\)
<=>\(4m^2-6m+10=10\Leftrightarrow2m\left(2m-3\right)=0\)
<=>\(\orbr{\begin{cases}m=0\\m=\frac{3}{2}\end{cases}}\)
c, Từ (2) => \(m=\frac{x_1+x_2+2}{2}\)
Thay m vào (3) ta có: \(x_1x_2=\frac{-x_1-x_2-2}{2}-3=\frac{-x_1-x_2-8}{2}\)
<=>\(2x_1x_2+x_1+x_2=-8\)
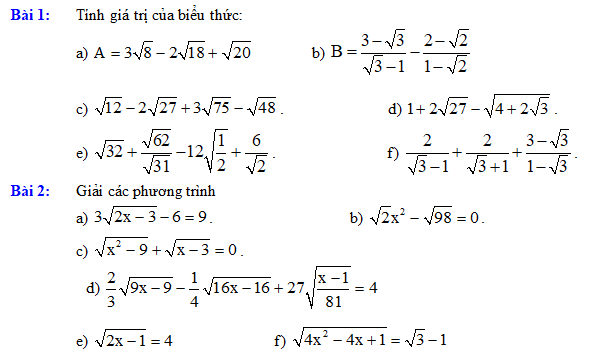
\(\dfrac{\sqrt{15}-\sqrt{20}}{10-\sqrt{75}}\)
\(=\dfrac{\sqrt{15}-2\sqrt{5}}{10-5\sqrt{3}}\)
\(=\dfrac{\sqrt{5}(\sqrt{3}-2)}{-5(\sqrt{3}-2)}\)
\(=\dfrac{-\sqrt{5}}{5}\)
\(=\dfrac{\sqrt{15}-2\sqrt{5}}{10-5\sqrt{3}}=\dfrac{\sqrt{5}\left(\sqrt{3}-2\right)}{5\left(2-\sqrt{3}\right)}=\dfrac{-\sqrt{5}\left(2-\sqrt{3}\right)}{5\left(2-\sqrt{3}\right)}=\dfrac{-\sqrt{5}}{5}=-\dfrac{1}{\sqrt{5}}\)